Maximum number of pieces of equal area you can obtain by cutting a pizza a certain number of times

Question

If I cut a perfectly circular pizza through its center 6 times at 30° angles, I get 12 pieces of equal area.

If I don't have to cut through the center, I can cut in a grid shape to divide the pizza into 16 pieces, but only the middle four will be of equal area.

Theoretically I could cut the pizza into a total of 22 pieces using 6 cuts, but more often than not none of them would be of equal area.

For 6 cuts, what is the largest number of pieces of equal size I can obtain (using only straight cuts that go through the entire pizza)? What about $n$ cuts?

---

And since people are posting answers to this effect, no, you are not allowed to fold the pizza or rearrange slices. This is not a lateral thinking puzzle.

Answer 1

UPDATE: I've added a large $N$ solution for multiples of 3 that slightly betters OP's solution at $3\times (\frac N 3 - 1)^2 + 9$, see end of this post.

Just to show that @humn is not the only one capable of wasting eyewatering amounts of pizza here are

15

tiny but equal pieces of pizza made using 6 cuts.

Due to symmetries there are only tree kinds of pieces; equalizing those costs 2 degrees of freedom which we can afford: Let $P$ be the point in the upper center where the blue and orange triangles meet. Then we can adjust the distance of P to the center and the angle between the lines meeting at $P$.

$N = 3n$ solution:

Example $N=12$, 36 slices:

Answer 2

☆   Revised to introduce a general solution of limited value, to include a solution for 7 cuts, and to acknowledge a better 6-cut solution   ☆

What about $n$ cuts?

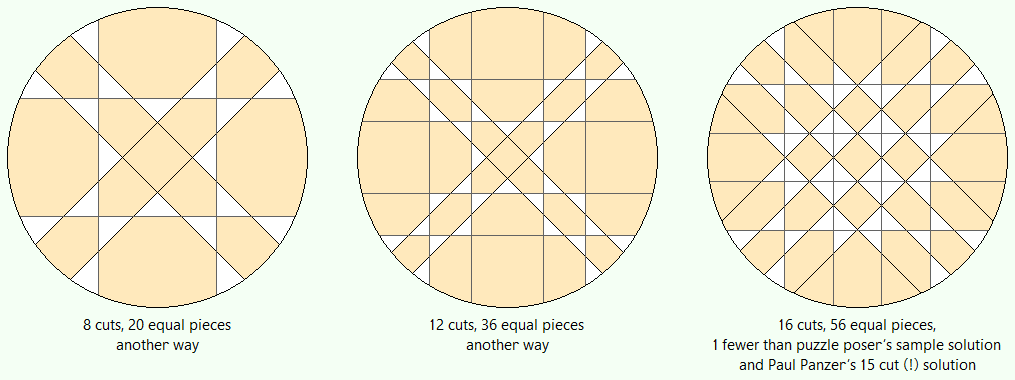
Hats off to Paul Panzer’s tasty general solution, which produces more equally sized pieces than puzzle poser Joe Z.’s general sample solution for  $n = 6k > 6$  cuts.   The parabolic result-curves of both those general solutions, however, begin slower than a linearly growing general solution that produces $4(n{-}3)$ equally sized pieces from $n = 2k \ge 6$  cuts.   This linearly growing general solution is an improvement only in the narrow domain of  $6 \,{<}\, n \,{<}\, 12$  cuts.

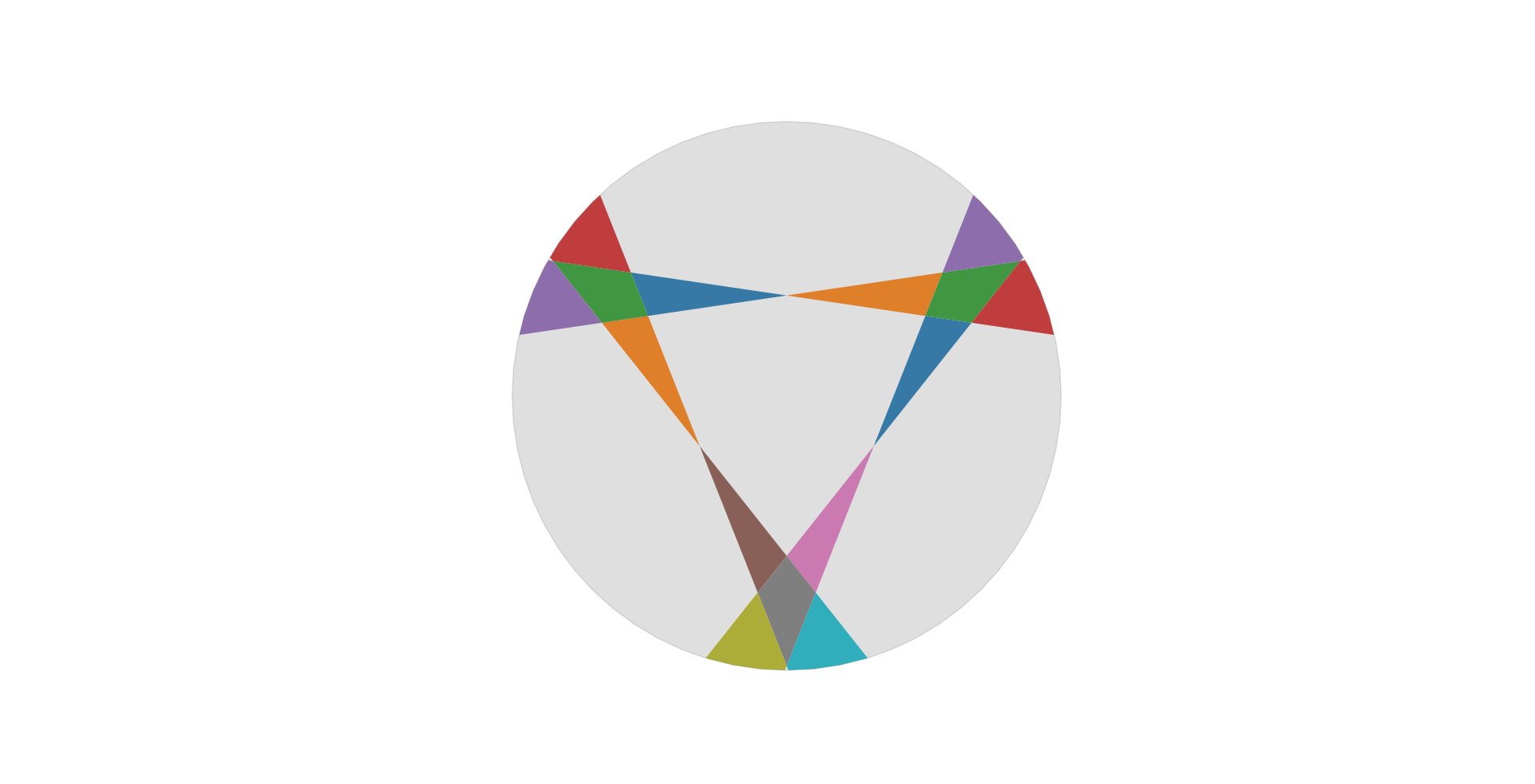
None of the general solutions so far cover the case of $n \,{=}\, 7$ cuts. Here is a solution with rectangular symmetry whose number of equal pieces can easily be matched by Paul Panzer’s specific solution for 6 cuts merely by slicing a single segment of pizza along an appropriately measured chord.

         7 cuts, 16 equally sized pieces

• Triangles E through L are congruent with area a b / 2.

• Suitably adjusted values for a and b can make the areas of pieces A and B also a b / 2, and thus of pieces C, D, M, N, O and P as well.   This is because increasing the value of a decreases the sum of areas A+B while increasing the value of b increases the ratio of areas B/A.

Original post follows (slightly edited), which led to the solutions above.

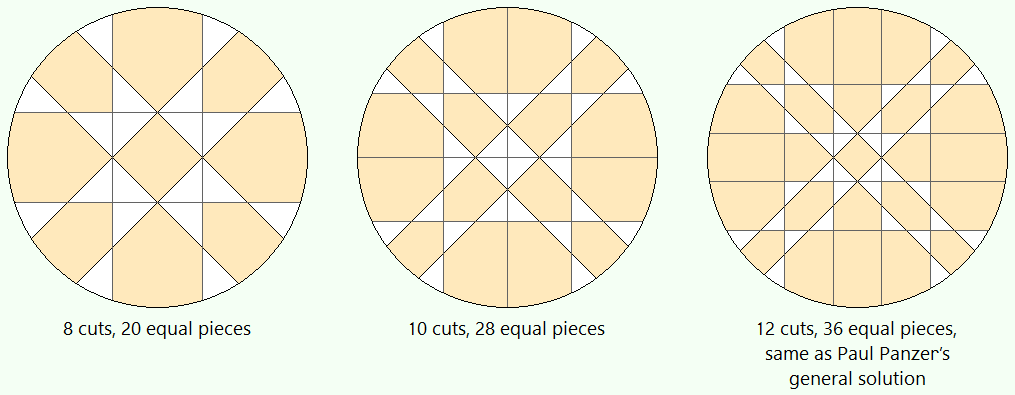
For 6 cuts, what is the largest number of pieces of equal size I can obtain (using only straight cuts that go through the entire pizza)?

This is not the most possible with $n \,{=}\, 6$ cuts but here are 14 equally sized pieces, A through N, out of 20 total pieces. This has 1 equal piece fewer than Paul Panzer’s beautiful 3-fold symmetric solution and 2 equal pieces more than both the  $2n \,{=}\, 12$  “naively” sliced sectors of the puzzle statement and the  $ \displaystyle \raise1ex\strut \small \big( {\raise-.4ex n \over 2} \kern.05em{-}\kern.1em 1 \big) \!\!\; \raise1.8ex{\scriptsize 2} \normalsize \! + 8 = 12 $   equal pieces produced by the general algorithm in puzzle poser Joe Z.’s sample solution.

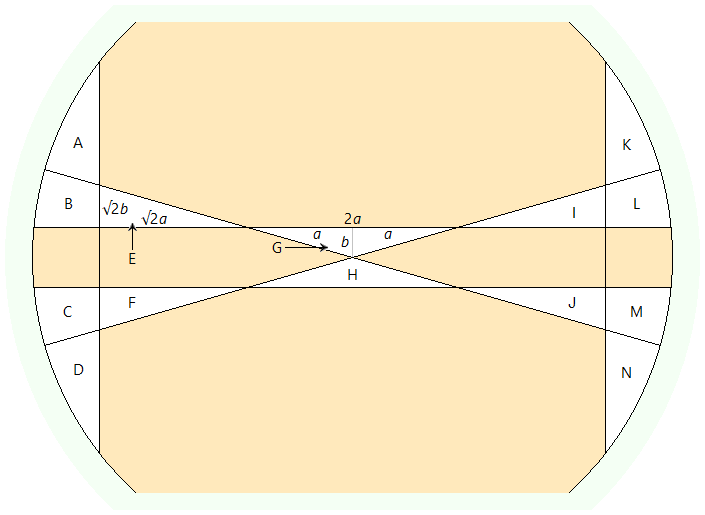
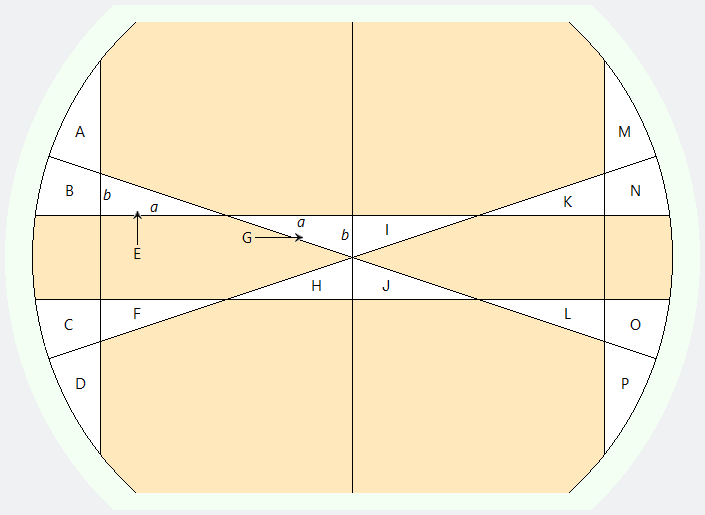
• The dimensions shown for triangles E and G clearly make both of their areas a b, and thus of pieces F, H, I and J as well.

• Suitably adjusted values for a and b can make the areas of pieces A and B also a b, and thus of pieces C, D, K, L, M and N as well.   This is because increasing the value of a decreases the sum of areas A+B while increasing the value of b increases the ratio of areas B/A.

A series of experiments led to the above dissection . . .

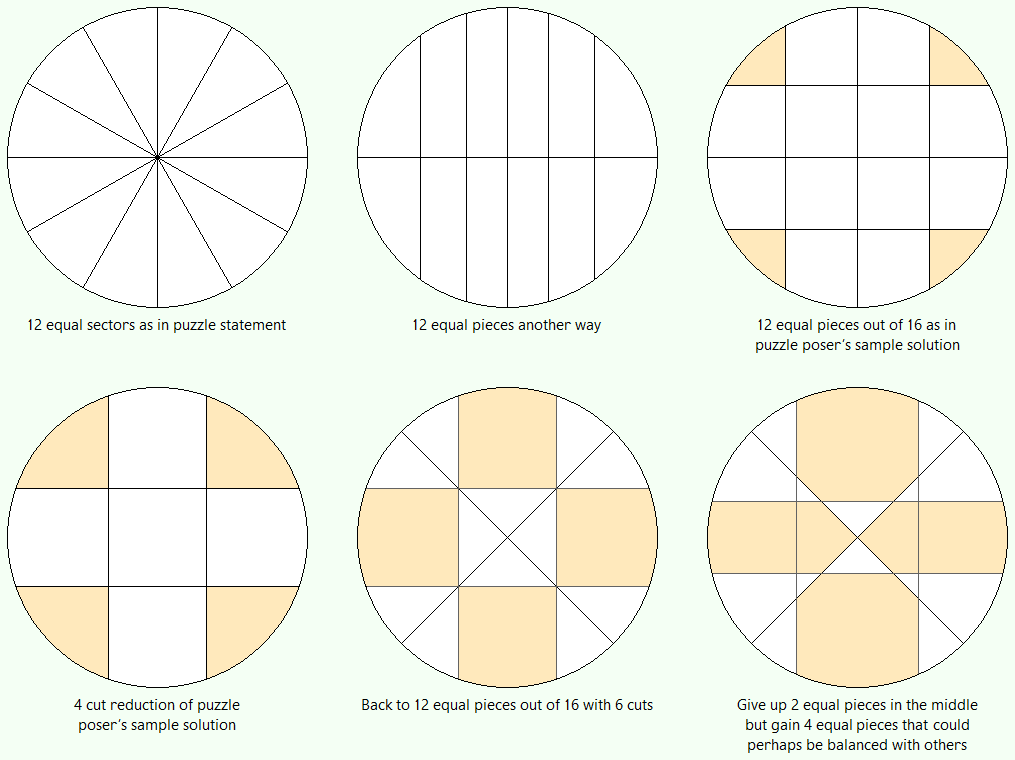
. . . along with some later experiments.

Answer 3

If you can fold the pizza, with one cut you could ideally create infinite slices. Just fold the pizza N times along the symmetry axis and cut it in the middle.

What you obtain is 2(N-1) triangular slices folded in half, and ready for eating.

5 folds, 1 cut (I know what you're thinking, dirty mind):

Answer 4

I'm aware of an algorithm for $(\frac{n}{2}-1)^2 + 8$ pieces given an even $n \ge 6$ cuts as follows:

Inscribe a square $S$ inside the circle. Let $d$ be some distance from the edge of the square, and cut $\frac{n}{2}$ times, equally spaced between $d$ away from one edge to $d$ away from the opposite edge, both horizontally and vertically (for a total of $n$ cuts).

By the Intermediate Value Theorem, there exists some value $d$ for which the 8 edge pieces adjacent to the corners and the inside pieces all have equal area, which is a total of $(\frac{n}{2}-1)^2 + 8$ pieces.

This overtakes the naive solution of $2n$ at $n = 8$. It gives $17$ pieces for $8$ cuts, $24$ pieces for $10$ cuts, $33$ pieces for $12$ cuts, etc.

There may be a solution better than this one, though. Anyone else willing to give it a shot?

Answer 5

I can only create 64 slices.

Step 1. Cut the pizza from North to South (2 slices)

Step 2. Cut the pizza from East to West (4 slicles)

Step 3. Lay the 4 slices on top of each other pointing East and cut from East to West (8 slices)

Step 4. Lay the 8 slices again on top of each other and slice (16 slices)

Step 5. Repeat 32 slices

Step 6. Repeat again 64 slices

The maximum number of slices is thus given by 2^n, where n is the number of cuts.

Answer 6

With a pizza, I guess the answer is logically 12, like you said. A taller cylinder is a different scenario, as you could cut through the middle height-wise, but this is not viable with a pizza. You could technically cut a cylinder into n discs of equal size.

Answer 7

Cut two equilateral triangles? This should give you both the same size pieces on the outside, as well as same size pieces on the inside.