Red and White Squares

Question

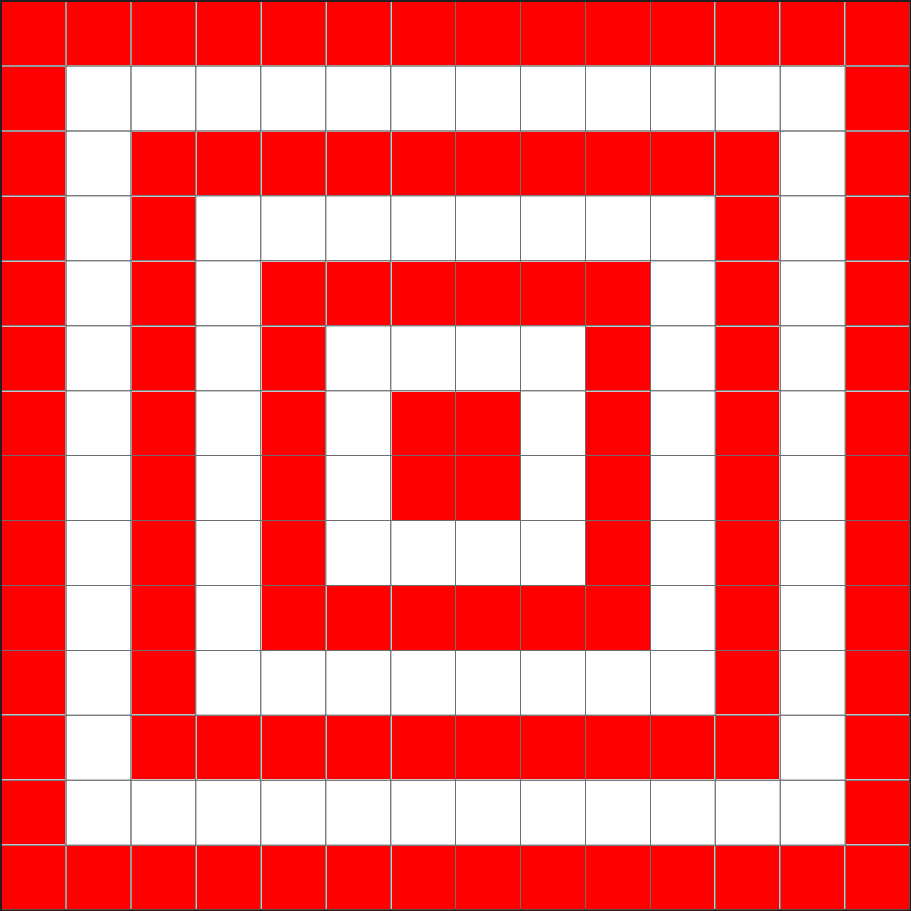
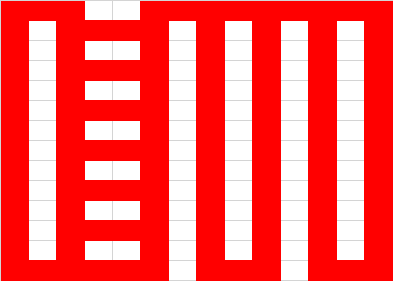
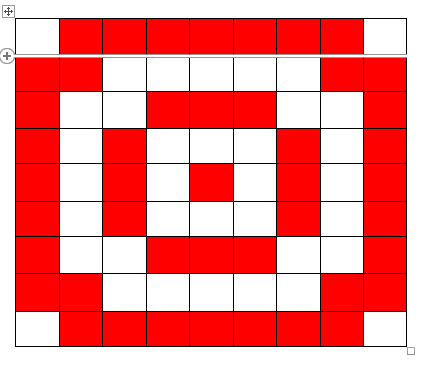
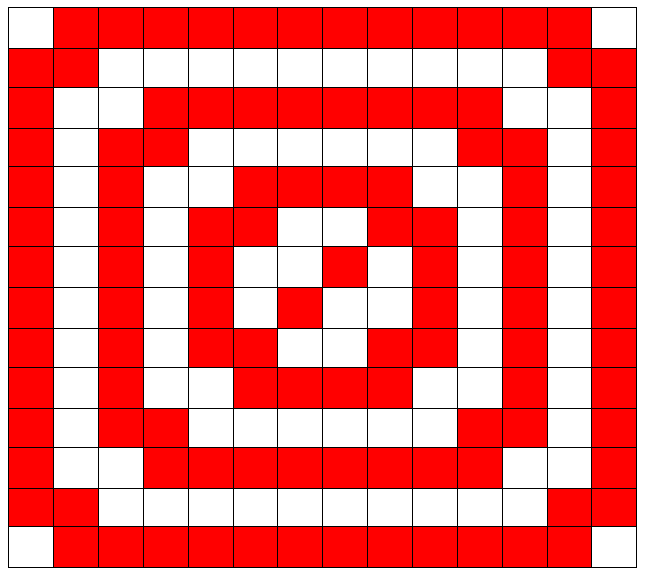
There are two grid boards with the dimension of 9x9 and 14x14, consist of all white squares. You are supposed to color some squares with a red color on the conditions below:

• In a board, if two square has a common edge, they are neighbor squares.
• Every square needs to have at least 2 colored neighbor squares (excluding itself)

so

What is the least number of red squares you can have with the condition above?

Answer 1

I think the answer for $14 \times 14$ is

$112$

Achieved as follows

While the best I've achieved for $9 \times 9$ is

$50$

Achieved as follows

Answer 2

For $14$x$14$ I got...

$112$

With this ...

Or with this...

Answer 3

I assume

the boards are independent

Then one solution would be

171 red squares.

As shown below

for 51 squares

And

for 120 squares

No idea if this is minimal though

Answer 4

9*9 solution:

45 reds

Looks like:

14*14 solution:

110 reds

Looks like: