An entry for Fortnightly Topic Challenge #40, have fun with non-Chess puzzle! ;)
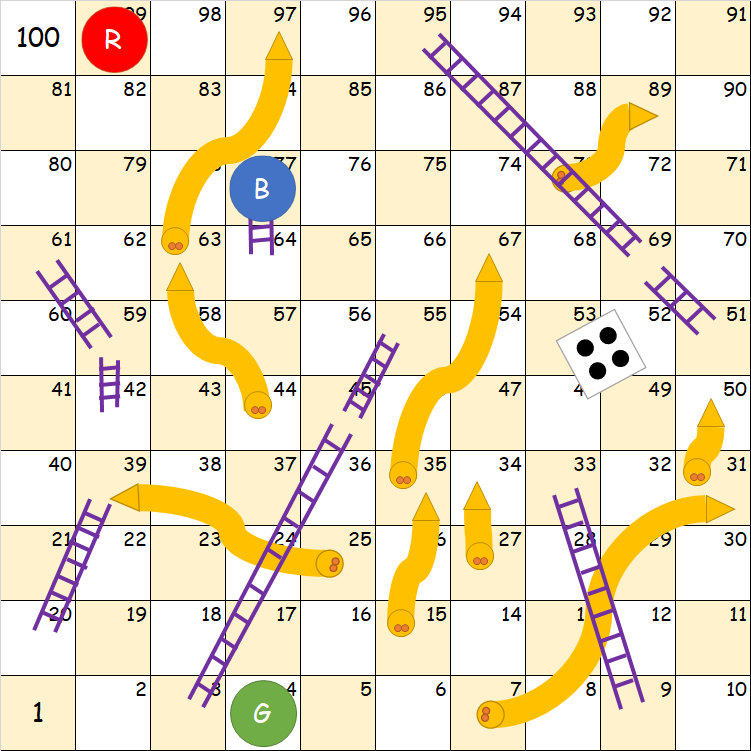
Three young children: Red, Green, and Blue; were playing a classic game of Snakes and Ladders, when suddenly their mom called them to have a dinner.
When they came back to their room, they forgot whose turn it was; even they were not sure either about the order of the play!
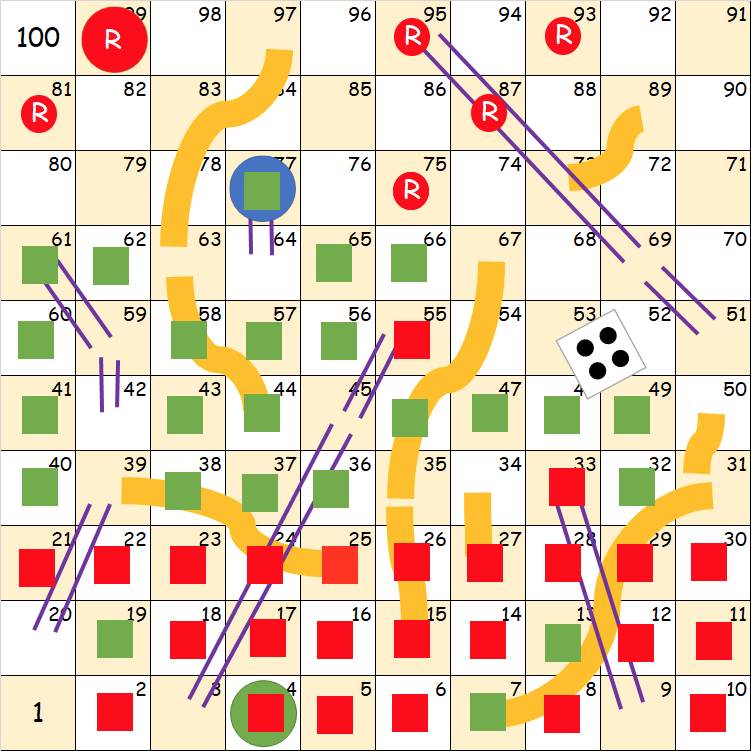
This was the last condition of the board, including the die. Could you tell them whose turn it is and the order of the play?
Some notes and clarifications:
- They put their markers at tile 1, at the beginning.
- They will win if their markers land exactly on tile 100. They are using the "bouncing-back" version (roll 3 will move the marker from tile 99 to 98).
- As long as they roll 6, they will get an extra roll after moving the marker.
- The ladders should be used to go to a higher number only, and the snakes are used to go to a lower number. For the "interrupted" spaces (the squares being described by @Bass in his first comment below), both are immediately used (e.g. just as landing on 39 will bring you to 25, landing on 20 will bring you to 25 too).
- If someone lands on a square where there is already a player, it doesn't send him back to square 1.