9
$\begingroup$

We have $6$ thin sticks with the same length parallel to each other shown below:

enter image description here

The distance between neighbor sticks are unknown and for sure smaller than half the length of the sticks themselves as seen in the diagram. Though it could be any arbitrary distance smaller than half the length of a stick.

So

By just moving $\mathbf 4$ sticks or fewer among them, can you form $\mathbf 8$ equilateral triangles?

Note: There are at least two answers that I know.

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ There's a special case that cannot be solved given the current constraint that "The distance between neighbor sticks ... could be any arbitrary distance smaller than the length of a stick": If every gap is wider than $\frac{\sqrt{3}}{2} \approx 0.866$ times the length of a stick, then a stick at a 60 degree angle won't be able to reach both of the two unmoved sticks. $\endgroup$
    – Bass
    Commented Jul 21, 2018 at 22:45
  • $\begingroup$ @Bass i actually tried not to mention any value not to give a hint but yes it should be less than 0.866, not smaller than the length of stick only. $\endgroup$
    – Oray
    Commented Jul 22, 2018 at 3:24
  • $\begingroup$ precision sufficient to form a geometric clue is not needed, perhaps the question should say less than half the length of a stick $\endgroup$
    – Jasen
    Commented Jul 22, 2018 at 3:25

1 Answer 1

Reset to default
13
$\begingroup$

Make one of these, maybe?

enter image description here

(Didn't see any requirements forbidding different sizes or overlapping.)

Here's another configuration:

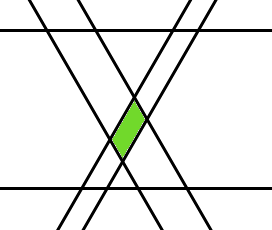
enter image description here

Each corner of the coloured area is the tip for two triangles, one pointing up, the other pointing down. (I deliberately made the shape a bit irregular: as long as the angles are correct, every triangle will be equilateral.)

This shape isn't as nice as the first one though: there's going to be a lot of useless stick ends jutting out, just waiting to poke someone's eye out. The first shape can always be constructed so that every part of every stick is needed to form the triangles.

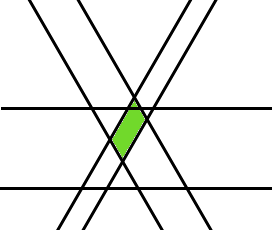
A third answer can be found between these two: move the top horizontal stick in the second shape down, so that it separates the top corner of the coloured area from the others. This flips one of the triangles, making the top part look like that in the first shape, but otherwise, everything stays the same.

Like so:

enter image description here

Edit: General solution

Now that I had some time to think about it, these are all (more or less) the same solution. As long as there's two sticks in all the three orientations corresponding to the sides of an equilateral triangle, and every stick crosses (or touches) all the non-parallel ones (with all the crossings and touchings happening at separate points), there will always be exactly 8 equilateral triangles: the oblique lines will always have exactly 4 crossing points, and each horizontal line will complete a triangle out of each crossing: it doesn't actually matter whether the horizontal line is above or below the crossing point. Here are a couple of examples not covered by the three cases above:

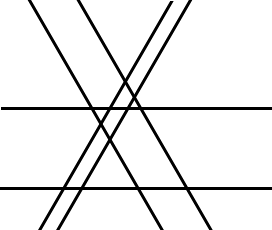
enter image description here enter image description here

Because it would be wasteful to put any sticks at any other angles than those that make equilateral triangles with the unmoved sticks, there are only two other orientation distributions to check: 1-1-4 (max. 4 triangles can be made) and 2-1-3 that has a maximum of 6 triangles. This means that this approach covers all possible solutions. (std. disclaimer: unless I'm missing something.)

Improve this answer
$\endgroup$
1
  • $\begingroup$ You can generalize. If you have two planes parallel to each of the four faces of a tetrahedron they will create ?? tetrahedra. And on into highre dimensions. $\endgroup$
    – Ethan Bolker
    Commented Jul 22, 2018 at 14:22

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.