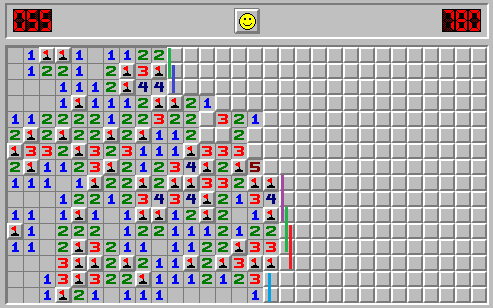
I can get this much:
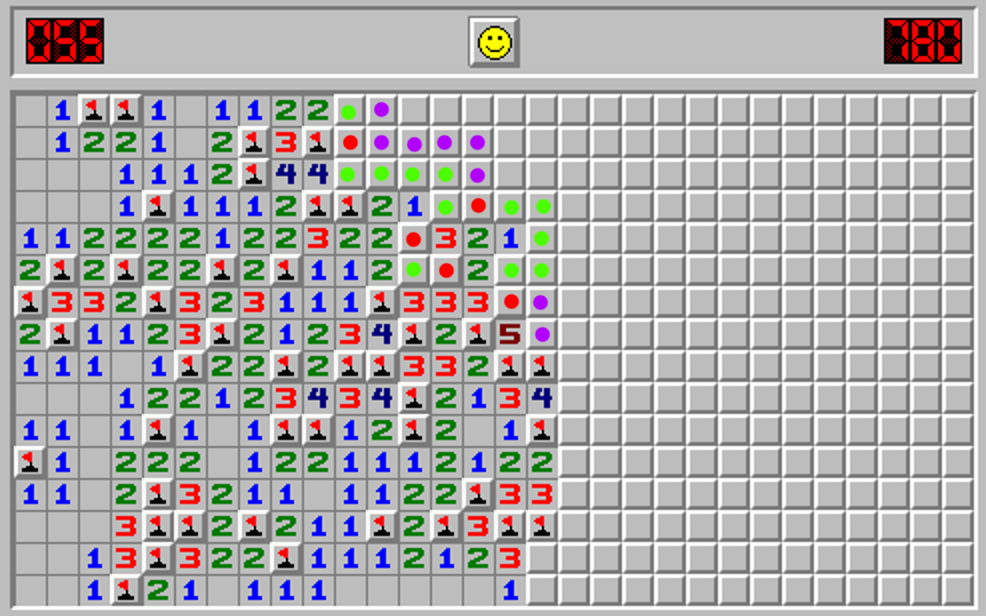
which is to say,
25 cells.
Here's how it goes.
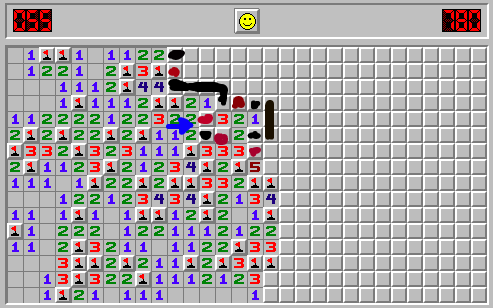
The green and red cells are, respectively, mine-free and mined. We begin by counting cells near the 3 in the 321 configuration to the northeast: exactly one to S+SW, at most one to W+N, hence one to NE (and exactly one to W+N, which gives us a bunch of mine-free cells around the 1). I think the inferences that give us the other red and green cells are straightforward.
Then
the purple cells are ones whose states we can definitely determine, though I can't tell you what the answer will be. For instance,look at the one "in the corner" at the top. We get that because the green cell to its southwest has only one neighbour; therefore, when we look at it (safe because it's green) we will discover that neighbour's state. Continuing with this sort of reasoning gives us the other purple cells at the top, and the upper of the two purples next to the 5 at the right. The other one next to the 5 is because we know how many mines are around the 5 and there's only one cell left.
After this
there will be some other cells we can expand -- e.g., one of those two next to the 5 must be un-mined -- but how many new cells this tells us the state of is not determinable so far as I can tell, and I think the number could be zero.
[EDITED to add:] Here's a sketch of how to prove that
after these we need not be able to determine any further cell-states.
Note that I am writing this after reading other people's solutions :-) but I haven't read them in much depth and am not deliberately copying anything.
So, first of all,
it's obvious that we can't, or at least mightn't be able to, do anything in the lower half. E.g., right at the bottom we need one mine but it could go in either of the two available cells; provided the two lower purple cells haven't given us anything extra (which clearly they needn't) the six cells above could be (top to bottom) any of X--X-X, -X--X-, or --X--X, which between them allow each of those cells to be in either state.
What about the top region? Well,
if all the upper purple cells turn out to contain mines (which they could) then we plainly learn nothing "beyond" them at the very top.
So the only question is
whether then the five known-clear cells next to the 1 near the top right have to tell us anything about the seven unknown-and-not-purple cells next to them. The answer is no. Let's suppose the upper of the two lower purple cells is mined and the lower unmined. Now, reading clockwise from the cell two above that 1, if we have X-X-X-- then those five cells say 3313; if we have -XXX--X then they say the same; and likewise if we have -X-X--X; and between them these possibilities put each of those cells into both possible states.
I conclude that
indeed it is possible that no other cell's state can be determined after finding those 25.