An enigmatic sudoku

Question

I entered the dungeon through a small, dark tunnel. After a seemingly endless walk I finally reached a small room. At the other side of the room, there was a big iron door. And in front of the door, there was a man holding a big key, which I was sure was the key to the door.

As I entered the room, the man said: "Hello, stranger. I guess you want to enter the dungeon of cluelessness."

"I indeed want to enter the dungeon" I said. "ButI never heard it called dungeon of cluelessness. People always refer to it just as the dungeon."

"Well," the man replied, "this dungeon is called the dungeon of cluelessness, as only the clueless want to enter it. It is full of the most complicated puzzles ever made. Fail to solve just one of them, and you are doomed."

"I thought I have to fight monsters?"

"Well, some of the puzzles are indeed monsters. They kill everyone who comes across, as no one ever could solve them."

"Well, I do not fear any puzzles" I replied.

"You should." the man replied. "You should really turn around and go. But if you still insist on entering the dungeon, I'll require you to first solve a puzzle. It's far easier than the puzzles you'll encounter in there. If you can't solve it, you certainly won't survive in the dungeon, so I won't let you in. But if you solve it, and still insist on entering the dungeon,I'll let you in. It's still stupid, but well, it's your life that you risk."

"Oh, great!" I said. "I'm good in puzzles! What type of puzzle is it?"

"A Sudoku" the man replied.

I was surprised. A Sudoku? And that was supposed to be the terribly hard puzzle? I mean, sure, not every Sudoku is simple, but how hard could it be?

"So where is that Sudoku?" I asked.

"I've drawn it here on the blackboard, ready for you to write the numbers into it."

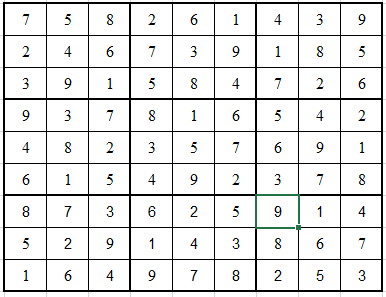
I looked at the blackboard, and didn't believe what I saw: The field was a standard 9-by-9 Sudoku field, but it was completely empty! Not a single number was pre-filled.

"Why are there no numbers written in it?" I asked.

"Well, that's your job. I mean, if the Sudoku were already filled out, there would not be anything left to do for you."

"Well, yes, sure, but shouldn't there be any hints?"

"Oh, there are hints" the man replied and pointed to a sheet of paper pinned to the wall right next to the blackboard. "They are written down there."

I approached the paper and read:

Things you need to know if you want to solve the Sudoku:

The diagonals are like the rows and columns.

The anti-diagonal is in best order.

About the subsquares, you should know the following:

Upper left: Diagonal hints are squared unless left. There are odd hints on the left, and even a middle row. The perfect hint is right in the middle. There is an odd number of corner hints; that number is one of them.

Upper middle: The first is even prime, the rest is unknown.

Upper right: Five hints, like dots on dice. There is even a diagonal. It starts squared, with a perfect end.

Middle left: In that position is the smallest composite. The rest is silence.

Center: A wizard did it.

Middle right: The one in the middle right you have to figure out yourself. Or rather, you have to figure out everything else.

Lower left: Everything has already been said.

Lower middle: The square is even in the center. That's all.

Lower right: The diagonal is going down. The numbers are multiple of their number.

Now, that was unexpected. It was clear that this text was somehow telling me which hints to place in the Sudoku, so I could then solve it. But what exactly did they mean?

Answer 1

I believe I have the answer:

The diagonals are like the rows and columns.

The anti-diagonal is in best order.

These two clues are pretty straight forward, the diagonals can only have one of each number [1-9] in them and the anti-diagonal has them in the $1,2,3,...,9$ or $9,8,7,...,1$ order.

About the subsquares, you should know the following:

Upper left: Diagonal hints are squared unless left (1). There are odd hints on the left(2), and even a middle row(3). The perfect hint is right in the middle(4). There is an odd number of corner hints; that number is one of them(5).

(1) The diagonal numbers are square numbers except for the left one so the middle and bottom right are 1,4 or 9.
(2) The left column has odd numbers.
(3) The middle row has only even numbers.
(4) 6 (the lowest perfect number) is in the middle right.
(5) There are 3 odd numbers in the corners and one of them is the 3.

Upper middle: The first is even prime, the rest is unknown.

The top left is a 2.

Upper right: Five hints, like dots on dice. There is even a diagonal. It starts squared, with a perfect end.

There are 5 hints (3 of them mentioned in the anti-diagonal) The diagonal has only even numbers. The top left is a square number, so 4, and the bottom right is 6.

Middle left: In that position is the smallest composite. The rest is silence.

That position is the middle left. the smallest composite number is 4.

Center: A wizard did it.

It's a magic square. (@Maria Deleva)

Middle right: The one in the middle right you have to figure out yourself. Or rather, you have to figure out everything else.

The middle right is a 1.

Lower left: Everything has already been said.

Not really a hint.

Lower middle: The square is even in the center. That's all.

The center square is an even square so 4.

Lower right: The diagonal is going down. The numbers are multiple of their number.

There are 3 numbers on the diagonal, each one is a multiple of 3, going in descending order.

Once I figured out what went where (with a little trial and error) I tossed it into a sudoku solver, as it involved using solving techniques I'm not super familiar with, and got my above answer.