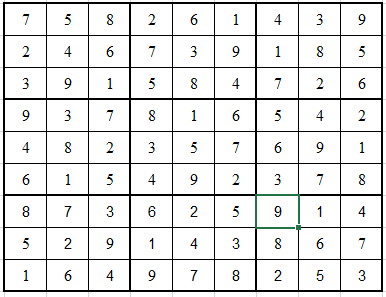
I believe I have the answer:
The diagonals are like the rows and columns.
The anti-diagonal is in best order.
These two clues are pretty straight forward, the diagonals can only have one of each number [1-9] in them and the anti-diagonal has them in the $1,2,3,...,9$ or $9,8,7,...,1$ order.
About the subsquares, you should know the following:
Upper left: Diagonal hints are squared unless left (1). There are odd hints on the left(2), and even a middle row(3). The perfect hint is right in the middle(4). There is an odd number of corner hints; that number is one of them(5).
(1) The diagonal numbers are square numbers except for the left one so the middle and bottom right are 1,4 or 9.
(2) The left column has odd numbers.
(3) The middle row has only even numbers.
(4) 6 (the lowest perfect number) is in the middle right.
(5) There are 3 odd numbers in the corners and one of them is the 3.
Upper middle: The first is even prime, the rest is unknown.
The top left is a 2.
Upper right: Five hints, like dots on dice. There is even a diagonal. It starts squared, with a perfect end.
There are 5 hints (3 of them mentioned in the anti-diagonal) The diagonal has only even numbers. The top left is a square number, so 4, and the bottom right is 6.
Middle left: In that position is the smallest composite. The rest is silence.
That position is the middle left. the smallest composite number is 4.
Center: A wizard did it.
It's a magic square. (@Maria Deleva)
Middle right: The one in the middle right you have to figure out yourself. Or rather, you have to figure out everything else.
The middle right is a 1.
Lower left: Everything has already been said.
Not really a hint.
Lower middle: The square is even in the center. That's all.
The center square is an even square so 4.
Lower right: The diagonal is going down. The numbers are multiple of their number.
There are 3 numbers on the diagonal, each one is a multiple of 3, going in descending order.
Once I figured out what went where (with a little trial and error) I tossed it into a sudoku solver, as it involved using solving techniques I'm not super familiar with, and got my above answer.