Hi guys I have been trying this for ages and can't work it out. Is it actually possible? P.S you can't move the doughnut/cut pieces.
1 Answer
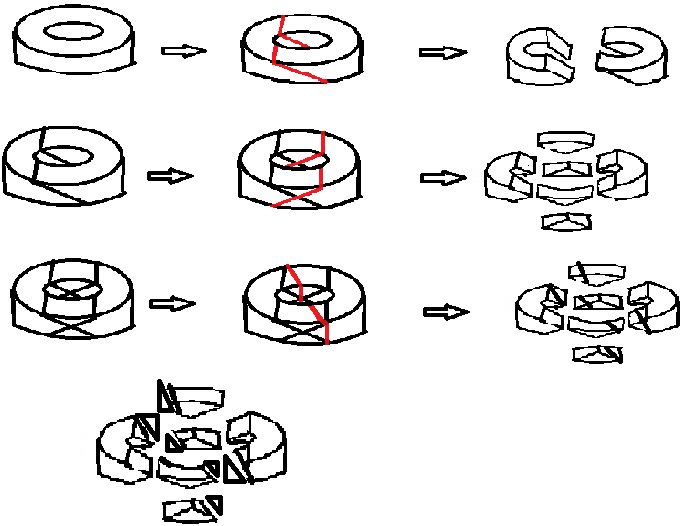
The first cut
cut the doughnut on a diagonal sideways. That is, think about a horizontal cut through the doughnut, and tilt it diagonally. This should give you two pieces, half circles with overlapping ends – the more overlapped, the better.
The second cut
cut the doughnut on the opposite (symmetrical) diagonal, forming an "X" set horizontally along the side of the doughnut. This should give you six pieces – two large half-circles, and a wedge on the top and bottom of the cut, on each side of the doughnut. The hole in the middle of the doughnut makes the pieces six instead of four, if the X were cut from the top rather than the side.
The third cut
line up a cut vertically, from the top of the doughnut straight down, but diagonally (as viewed from above). It should go from the right side of the front "X" to the left side of the back "X". This should cut every piece you already have into two pieces – cutting a corner each off of, say, the right side of all the front-and-right three, and the left corner each off the back-and-left three.
The 12 pieces
The pieces are now, the front top and bottom wedges, and two corners cut off the inner right side of those wedges, the right side half-circle, and the middle wedge cut off the outer left side of that half circle, the back top and bottom wedges, with two corners cut off the outside back edge of those wedges, and the left side half-circle, and the middle wedge cut off the inner right side of that half-circle.
My crude drawing, to help with confusion
-
$\begingroup$ All it takes is moving the intersection point of the 3 planes into the body of the doughnut, then chanting everything slightly to get one more piece (see the linked duplicate question's answer). $\endgroup$– ErikECommented Aug 6, 2016 at 17:04
-
$\begingroup$ @ErikE - I looked at that answer, and I looked at that picture - and I just can't get it, can't make it make sense in my head. There's not enough overlaps to get another piece cut, anywhere along the way. I think I need the in-between steps to make the angles fit, because their exploded view just looks like one of the chips broke in half and they didn't notice. I actually figured this answer out, while trying to make that one make sense - and left it as an answer because an answer of twelve actually fit this version of the question. $\endgroup$– MeghaCommented Aug 6, 2016 at 17:29
-
$\begingroup$ 3 planes coinciding make 8 sections, yes? Position the intersection within one side of the doughnut. Then, 7 of those sections can, with proper angling, be made to slice through the opposite side of the doughnut. Since 2 of those sections don't leave a new piece (they are part of the semicircle on each side) that leaves 5 more. What might help is, instead of imagining aligning planes, point one of the original pyramid-shaped sections directly at the opposite side of the doughnut. If you review that solution and my description, you might be able to see it now. $\endgroup$– ErikECommented Aug 6, 2016 at 18:03
-
1$\begingroup$ Absolutely correct for the question asked, however if the position of the last cut is altered slightly (shift it right by 1/4 the arc of the upper wedge), you can get a bit of the right-most half circle off the top as well as the bottom (without losing any other pieces), yielding 13 sections. $\endgroup$ Commented Aug 7, 2016 at 8:25