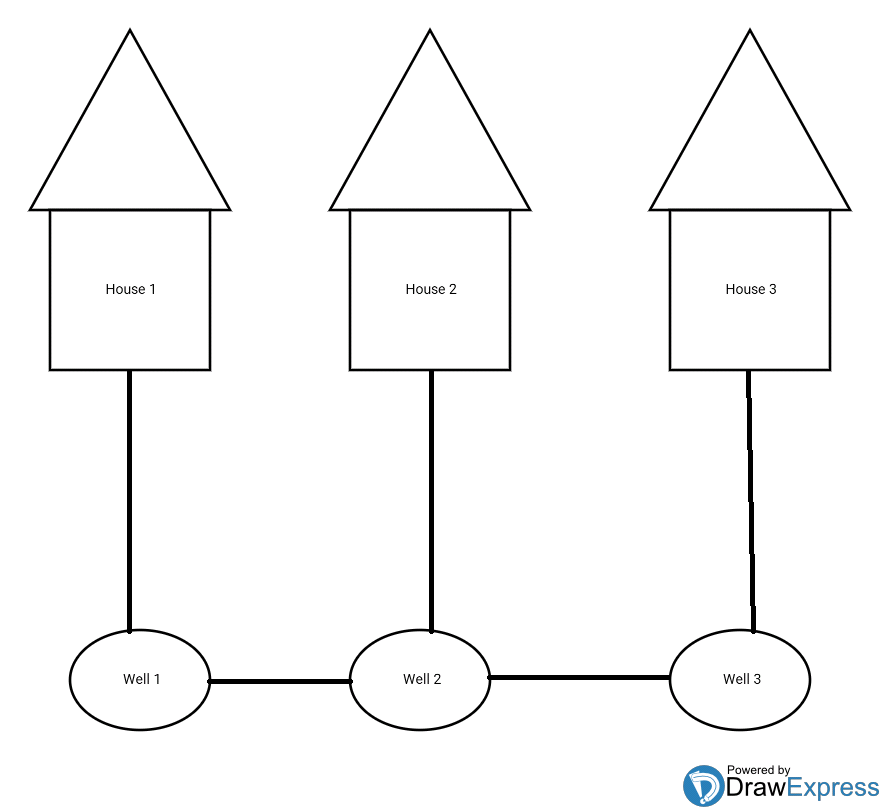
Connect every house with every well without the lines intersecting.

I am not sure if this puzzle has a solution. I have been puzzled by it for a long long time. An old man from my village mentioned this puzzle to me 12 years ago. I have never been able to solve it, I'm hoping someone here can.

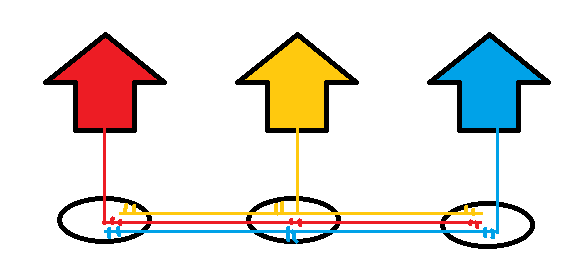
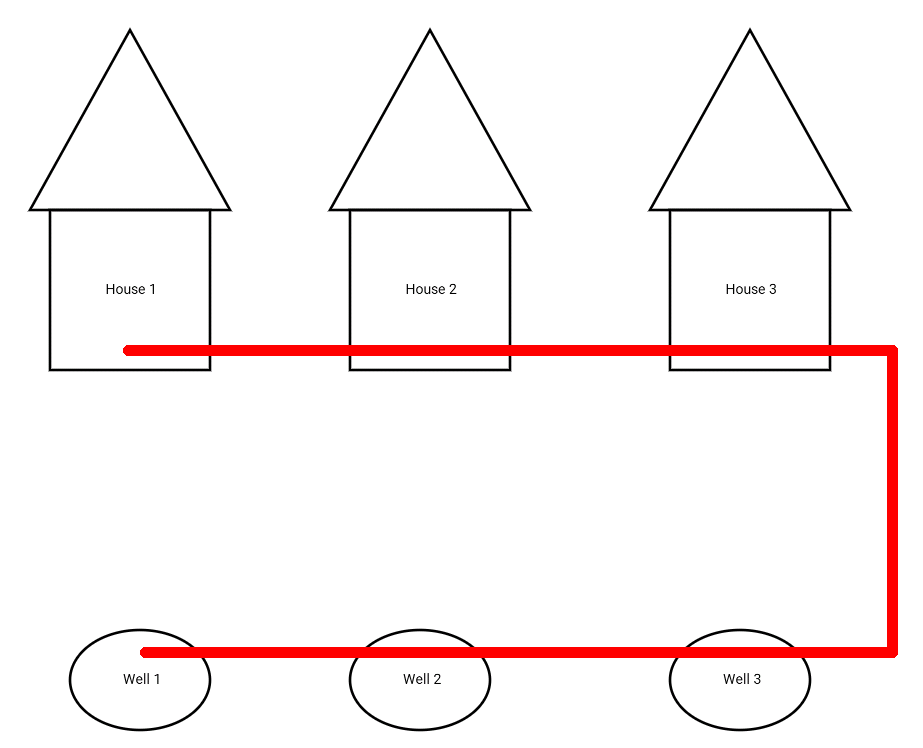

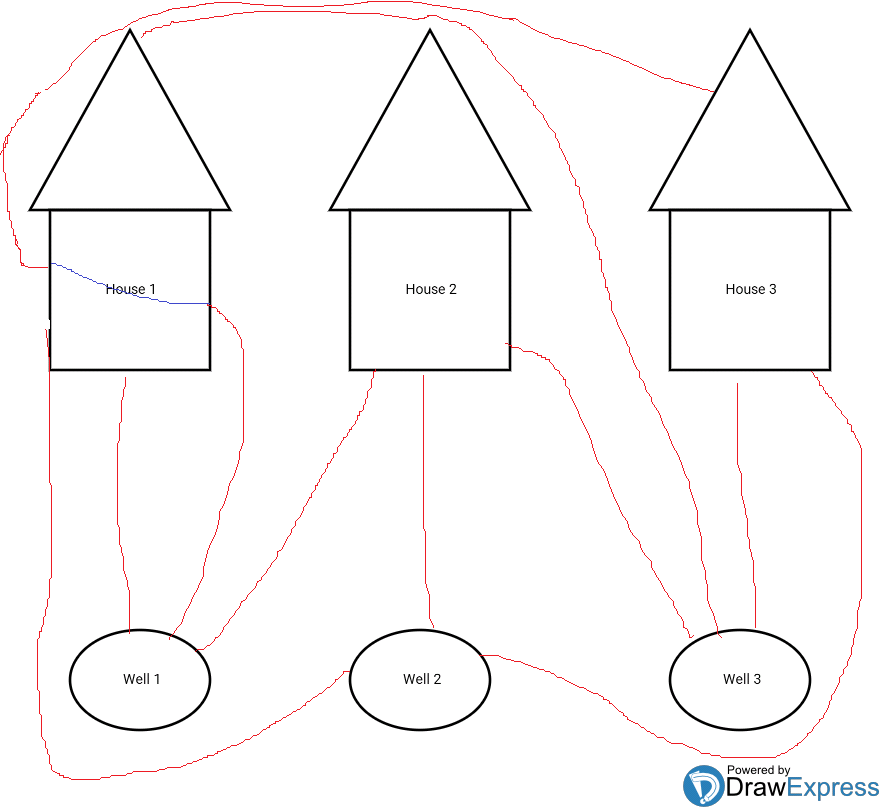
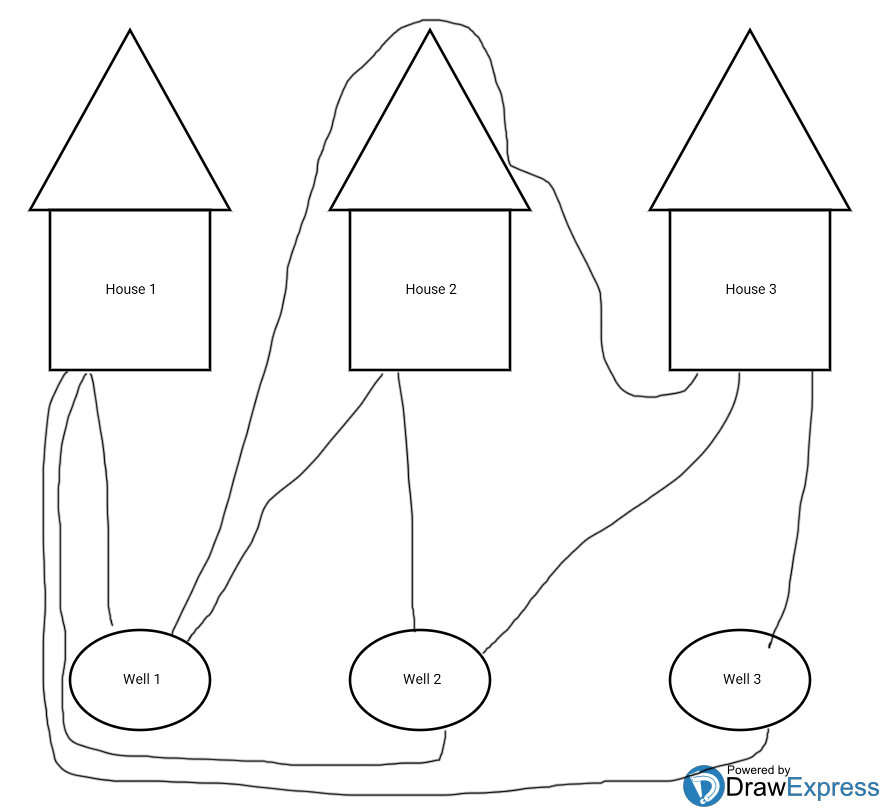 Hi,
Hi,