A simple geometric math puzzle (too simple for MO). Seeing two overlapping square beermats, I had the idea: One mat is the other rotated by an angle. All colored areas are the same (say 1, arbitrary unit). What is the size of the square and the rotation angle?
Bonus if you can solve it in your head even without the pic. (Which is suggestive; the suggestion is correct.)
Superbonus if you do the same question with two circular mats.
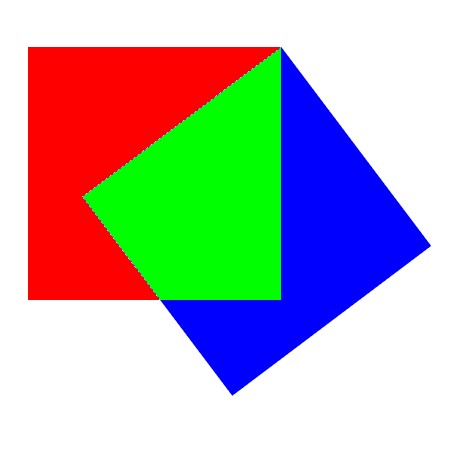
The size of the square is
2, because it is the sum of the red and the green region, and the question says each colored region has an area of 1.
It is clear that the green region is symmetric
with respect to its diagonal that connects the intersection point of two square edges and the rotation center, because the two triangles separated by the diagonal both contain a right angle, share the common hypotenuse, and have a corresponding pair of legs of equal length (the sides of the squares).
This means the angle spreaded by the green region near the rotation center is
$2\arctan(1/2)\approx 0.9273\ {\rm rad}\approx 53.13^\circ$. According to the analysis above. The green region has an area of 1 and can be divided into two triangles with right angle with an area of 1/2 each, with the total area of the square being 2. This means the intersection point is the midpoint of the square. which in turn means the angle for each of these two triangle at the rotation center is $\arctan(1/2)$ and the total angle spreaded by the green region at the rotation center is $2\arctan(1/2)$.
which means the rotation angle is,
$\pi/2 - 2\arctan(1/2) \approx 0.6435 {\rm rad}\approx 36.86^\circ$
For the circular mat problem,
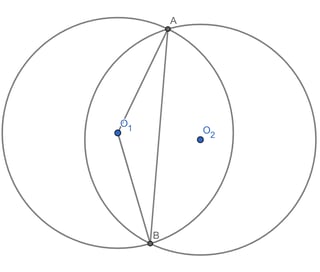
It boils down to
calculating the area of the circular segment $\stackrel{\frown}{AB}$, which is given by the difference of the sector $O_1AB$ and the triangle $O_1AB$. For convenience, take the radius of the circle as 1. The area is then given by $(\theta - \sin\theta)/2$, where $\theta$ is $\angle AO_1B$.
So we need to solve the equation
$(\theta - \sin\theta)/2 = \pi/4$, because the question requires that the area of the overlapping region is half the area of the whole circle (with a radius of 1 and an area of $\pi$), and the overlapping shape is the sum of two circular segments with the same area (actually they are congruent, but the proof is not necessary here). So each circular segment has an area of $\pi/4$.
The solution is
$\theta\approx 2.3099\ {\rm rad} \approx 132.34 ^\circ$
which means the rotation angle is,
$ \angle O_1AO_2 = 2\angle O_1AB = \angle O_1AB+\angle O_1BA = \pi - \theta \approx 0.8317\ {\rm rad} \approx 47.65 ^\circ$.
The ratio of the area of any colored region with the area of a full square is
$\frac{1}{2}$
and the two (boundaries of the) squares intersect at
the center point of the obvious edge, and this allows to compute the angle.
First we show the first assertion:
let us denote by $r,g,b$ the areas of the colored regions. By assumption, they are equal. But $r+g$ is equal to the area of one square, so we know that each square is twice the size of any colored region.
Then, we show that the proposed setting achieves the desired condition:
consider a line joining the midpoint of an edge of a square to a corner opposite to this edge. The this line divides the square in two regions, which one of the areas being easily shown to be a quarter of the size of the square. Adding to this piece its reflection along the line gives us a kite shape, the area of which is exactly half of the area of the square. Now draw the reflection of the starting square along the line. This gives two squares having the desired property.
Of course, the solution is unique since
the green area strictly decreases with the angle, until it is $0$, so $\frac{1}{2}$ is only attained once.
I do not have an answer for circles!
