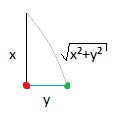
(This is the sequel to this puzzle. It has a similar setup, but believe me, the solution is very different. Be careful! The answer is counterintuitive. It shocked me at first.)
You are a pirate. Previously, you captured a rogue mutineer. She begged for a second chance on the crew, in exchange for the location of her criminal headquarters: Marauders' Circular Cove.
In promise of great treasure, you raided the cove at night. But it was a trap! Now smugglers are coming out of the forest, more than you can count.

(Pictured: there are WAY MORE than this)
Your ship moves at the same speed as the smugglers. The smugglers cannot swim. If you manage to take even one step onto the shore, then you can instantly beach the ship and escape into the cover of darkness.
Right now (and only right now, i.e. not after the chase starts), you can bribe some of the smugglers to leave, so that you only have to evade $n$ of them. Your goal is to reach the shore without getting caught. The smugglers' goal is to catch you when you reach the shore, or starve you out on the lake forever.
How many smugglers can you evade, if the cove is shaped like a perfect circle?
The ship and smugglers are all points of zero radius. Everyone can always see everyone else's position. This is not a loophole-finding contest: there are no issues with reaction time, scurvy, et cetera.

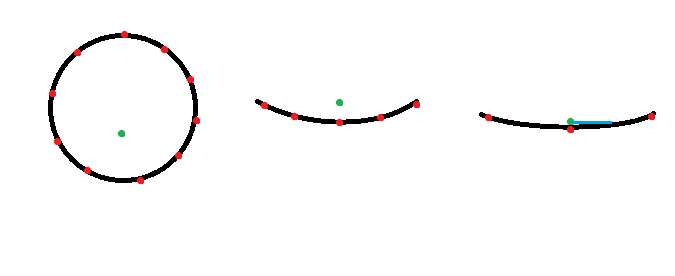 Suppose you have a very very high number of smugglers. The strategy to escape is approaching the coast as much as possible (the more the smugglers, the closest you have to be), then follow a straight line (a chord) which is always shorter than the smugglers' path to reach you.
Suppose you have a very very high number of smugglers. The strategy to escape is approaching the coast as much as possible (the more the smugglers, the closest you have to be), then follow a straight line (a chord) which is always shorter than the smugglers' path to reach you.