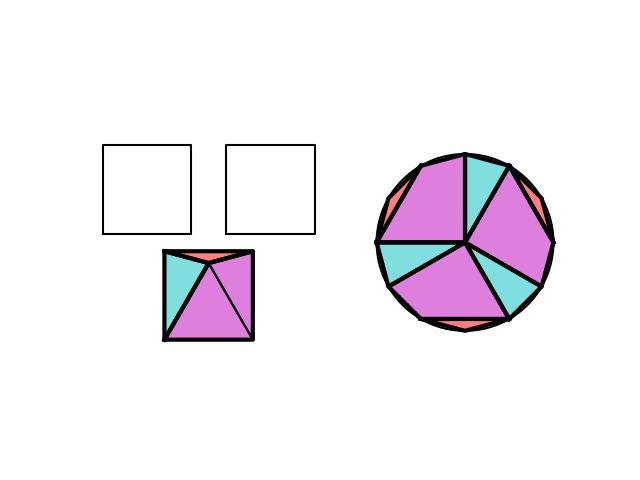121
$\begingroup$

It seems that once upon a time some politicians tried to pass a law fixing the value of π to be exactly 3. The idea being to "make math simpler so that our children can get better at math".

Don't let this happen.

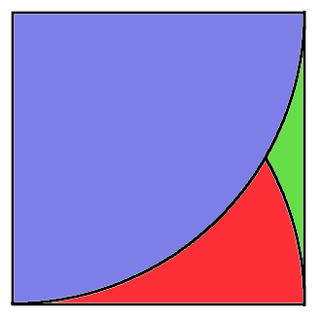
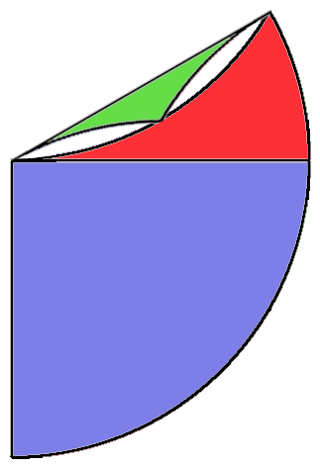
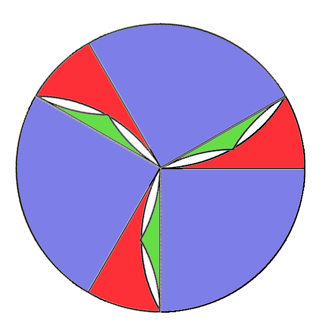
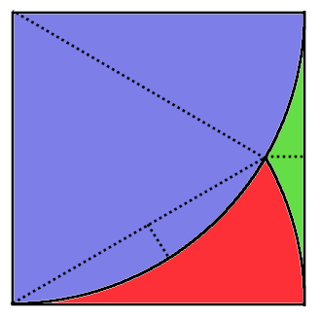
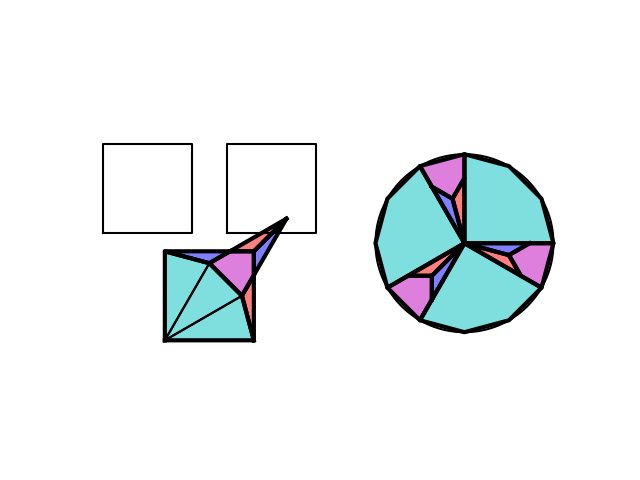
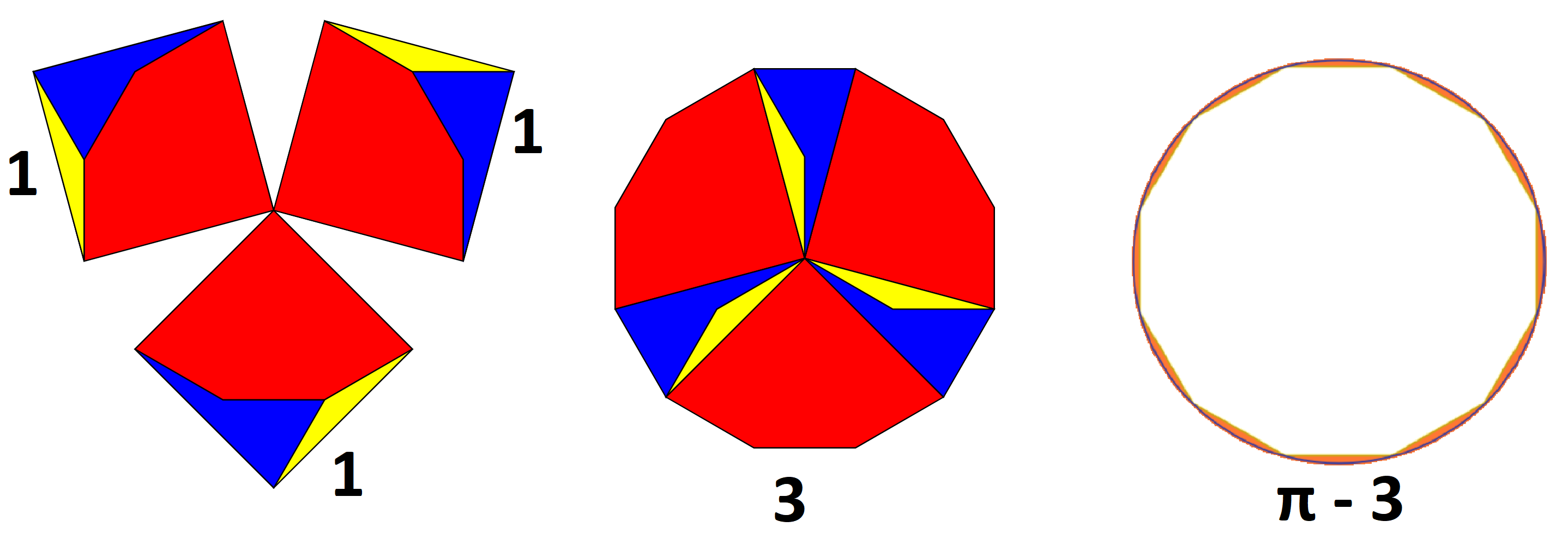
The area of a disk is given by A = πr². If you take r = 1 you have A = π. Prove that A > 3 by fitting 3 unit squares on a disk with unit radius. Cut the three squares into a finite number of pieces and place these on the disk without overflow, without overlap, and with some space left.

Minimize the number of pieces.

a group of three unit squares next to one circle with unit radius

Banach–Tarski-like tricks are not allowed. ;-) The solution should be practical enough to squeeze three toasts on a round plate or three slices of square cheese on a pizza.

(this puzzle is my own creation)

Improve this question
$\endgroup$
10
  • 8
    $\begingroup$ I also don't see how Banach Tarski could be used here. But if it can, it is not allowed. $\endgroup$
    – Florian F
    Commented Aug 28, 2021 at 9:18
  • 8
    $\begingroup$ Florian and @quest - en.wikipedia.org/wiki/Tarski%27s_circle-squaring_problem - i.e., Banach-Tarski tricks are possible. $\endgroup$
    – Victor Stafusa
    Commented Aug 28, 2021 at 22:45
  • 3
    $\begingroup$ Wow. Actually I mentioned Banach-Tarski as a joke. I know it works in 3D. But as it seems, it wasn't that far-fetched. Thanks for sharing. $\endgroup$
    – Florian F
    Commented Aug 28, 2021 at 22:58
  • 5
    $\begingroup$ Your comment about legislation is seriously over-generalized. One bill was once introduced to committee in the Indiana Legislature. It went no further. Even Hoosiers have some common sense. $\endgroup$
    – David Smith
    Commented Aug 29, 2021 at 17:01
  • 2
    $\begingroup$ Frankly, I didn't know whether that story was real or not. It is a rumor I heard. Take it just as a pretext to introduce the problem. I changed the wording. $\endgroup$
    – Florian F
    Commented Aug 29, 2021 at 21:02

3 Answers 3

Reset to default
138
$\begingroup$

I didn't have a knife with me, so I only used my unit circle cookie cutter to split each square like this:

enter image description here

I then rearranged the parts into this shape:

enter image description here

Since the angle covered by this shape is exactly 120 degrees (see the final spoiler block to confirm), three of these make a nice circle, with some white shining through the gaps:

enter image description here

Since the fit of the green piece into the indentation in the red one is a bit snug (it's an exact fit, actually), let's confirm that there's no overlap:

enter image description here

The gap in the red piece and the tallest part of the green piece both have the height of "1 minus the height of an equilateral unit triangle", so the fit is exact, and we are done.

Improve this answer
$\endgroup$
6
  • 4
    $\begingroup$ That's a hell of a cookie (or a tiny pizza) ;-) $\endgroup$
    – loopy walt
    Commented Aug 28, 2021 at 10:26
  • 12
    $\begingroup$ Well, it's a unit pizza, so you can click on the image to choose a unit that makes it less tiny :-) $\endgroup$
    – Bass
    Commented Aug 28, 2021 at 10:29
  • 1
    $\begingroup$ Do you think it might be possible to achieve 8 pieces, by cutting all 3 squares into the blue piece and the remainder, putting the 3 blue pieces as 3 quarters of the circle and one of the remainders into the 4th quarter, and then splitting the other two remainders and fitting them into the lens shape that's left in the 4th quarter? I don't know if there's enough room in the last step. $\endgroup$
    – xnor
    Commented Aug 29, 2021 at 22:32
  • 2
    $\begingroup$ @xnor that was what I was attempting to do when I came up with this solution, couldn't figure out a way to make it work because everything was just too concave. That's not conclusive, of course. $\endgroup$
    – Bass
    Commented Aug 30, 2021 at 4:43
  • $\begingroup$ You could also do this with straight cuts and the same basic construction. Nicer, IMHO. You end up with a regular 12-sided figure. Update: I guess that ends up similar to @loopywalt. $\endgroup$
    – Dr Xorile
    Commented Sep 8, 2021 at 1:07
67
$\begingroup$

How about this?

enter image description here
This creates from a unit square four pizza slices of a regular 12-gon inscribed in the unit circle. (3 whole ones (can be left together if we want to minimize total number of bits), one pieced together, note that no flipping is required, no need for upside-down pizza =-) )

Why does it work?

The regular 12-gon has 30° slices so we can fit 3 of them in a corner of the square. As for the pieced together slice we need to show that the red and blue triangles are congruent. By chasing angles we see that they are similar with acute angles 15° and 30°. The easiest way to show that they are the same size is by comparing the areas of the squares (3x1) and of the inscribed regular 12-gon (3, essentially because sin 30° = 1/2)

Alternative cut:

Also four UPDATE thanks @Jaap Scherphuis three: leave the left (or right) triangle connected to the bottom one and join the top one to the right (or left) /UPDATE bits per square:
enter image description here
join the top and bottom triangles at their horizontal sides. This will result in a "double slice" to complement the two slices left and right.

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ @RobPratt we could leave the three whole slices in one piece, for a total of 3x4. $\endgroup$
    – loopy walt
    Commented Aug 27, 2021 at 23:51
  • 3
    $\begingroup$ In your alternative cut, you can leave one slice connected to the equilateral triangle, for only 9 pieces in total. $\endgroup$
    – Jaap Scherphuis
    Commented Aug 28, 2021 at 7:20
  • 1
    $\begingroup$ Thank you. Your solution is now complete and correct. But now I have a problem. in the meantime Bass posted a different solution, which is better explained and illustrated. Even though you gave the essence of the solution first, I will accept his solution because of the better presentation. I hope you are not upset by that choice. $\endgroup$
    – Florian F
    Commented Aug 28, 2021 at 10:43
  • 2
    $\begingroup$ @FlorianF no worries. $\endgroup$
    – loopy walt
    Commented Aug 28, 2021 at 10:44
  • 2
    $\begingroup$ @MattTimmermans Careful, I think most people would understand "unit regular n-gon" as one having unit side length. This one is inscribed in a unit circle. $\endgroup$
    – loopy walt
    Commented Aug 28, 2021 at 17:45
13
$\begingroup$

Starting with three squares of side 1, we can rearrange them into dodecagon. The total area of the squares or dodecagon is 3. After we eat dodecagon out of the pizza (wheel), the scraps make up the extra area of 12 crescents.

Pizza = Dodecagon + 12 crescents

Since the area of 12 crescents is visibly above zero, the area of a wheel must be above 3.

enter image description here

I did it with a help of: https://demonstrations.wolfram.com/FreesesDissectionOfARegularDodecagonIntoThreeCongruentSquare/

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ This is almost the same solution as Bass gave one year ago. But thank you for the link to the very relevant Wolfram page, I didn't know about it. $\endgroup$
    – Florian F
    Commented Sep 13, 2022 at 11:58
  • 4
    $\begingroup$ Bass's approach is different. Bass cuts with curved lines, me with straight. Bass approach: Pizza = 3 Squares + 6 Lens. My approach: Pizza = 3 Squares + 12 Crescents. The dodecagon is a common thread between LoopyWalt and my approach, though we also cut the squares differently. $\endgroup$
    – Przemyslaw Remin
    Commented Sep 13, 2022 at 15:58

Not the answer you're looking for? Browse other questions tagged or ask your own question.