4
$\begingroup$

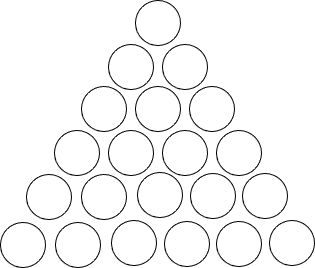
Place a different prime number or perfect square in each of the twenty-one disks that make up the triangle below, so that the number in any disk that lies on two others is precisely the sum of the numbers in those disks. Do so in such a way that the number in the apex is as small as possible.

enter image description here

Source: Based on a puzzle in here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

A simple upper bound:

In barycentric coordinates: $x_{abc} = 9^a 16^b 25^c$. This gives a maximum at the tip $x_{005} = 9765625$.

And a brute-forced computer solution, barring coding mistakes this should be minimal:

                               147456

                           63487*    83969*

                      33211*    30276     53693*

                 17424     15787*    14489*    39204

            10853*     6571*     9216      5273*    33931*

       10529*     324       6247*     2969*     2304     31627*

The starred numbers are prime.

Improve this answer
$\endgroup$
1

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.