0
$\begingroup$

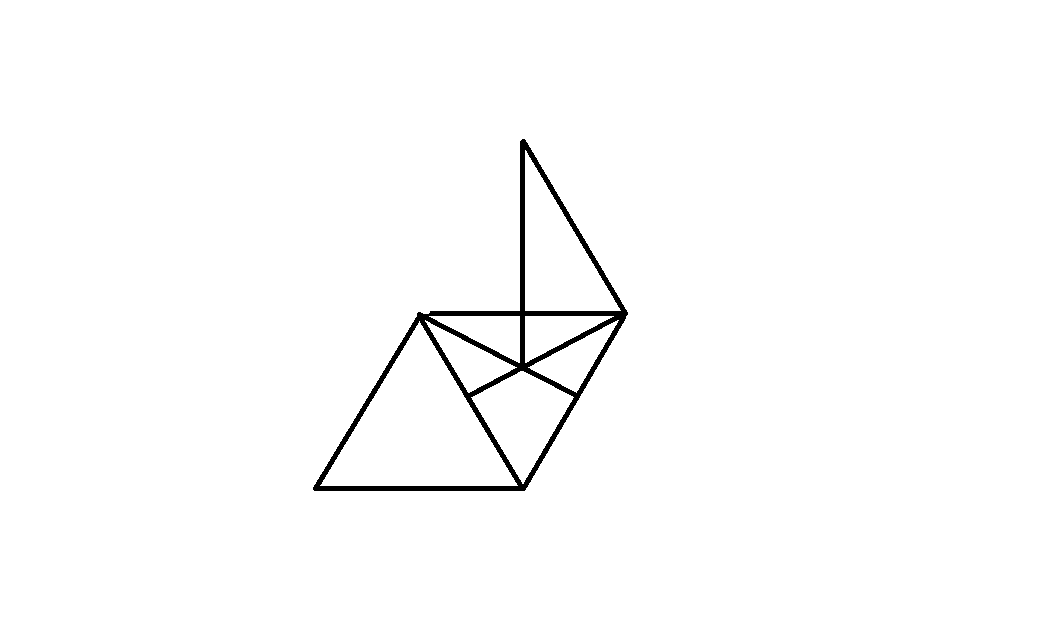
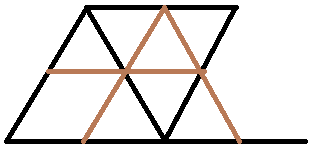
The problem is as follows:

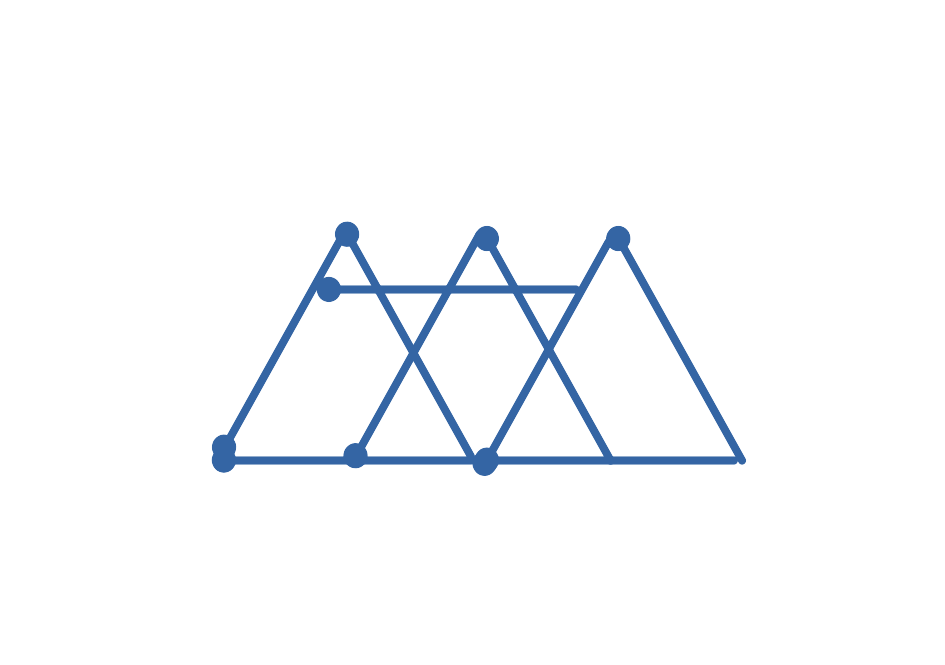
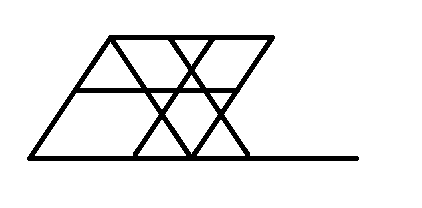
The figure from below shows 9 matches. If only 3 of them are changed from their positions, then what is the most number of triangles that can be made?

Sketch of the problem

The alternatives given in my book are as follows:

  1. 9 triangles
  2. 10 triangles
  3. 11 triangles
  4. 12 triangles
  5. 14 triangles

I found this problem in my book Reason and Logic from 2000s under the chapter on optimization. From the style it seems to be an adaptation from a reprinted copy of Martin Gardner's 50's book of Recreational puzzles.

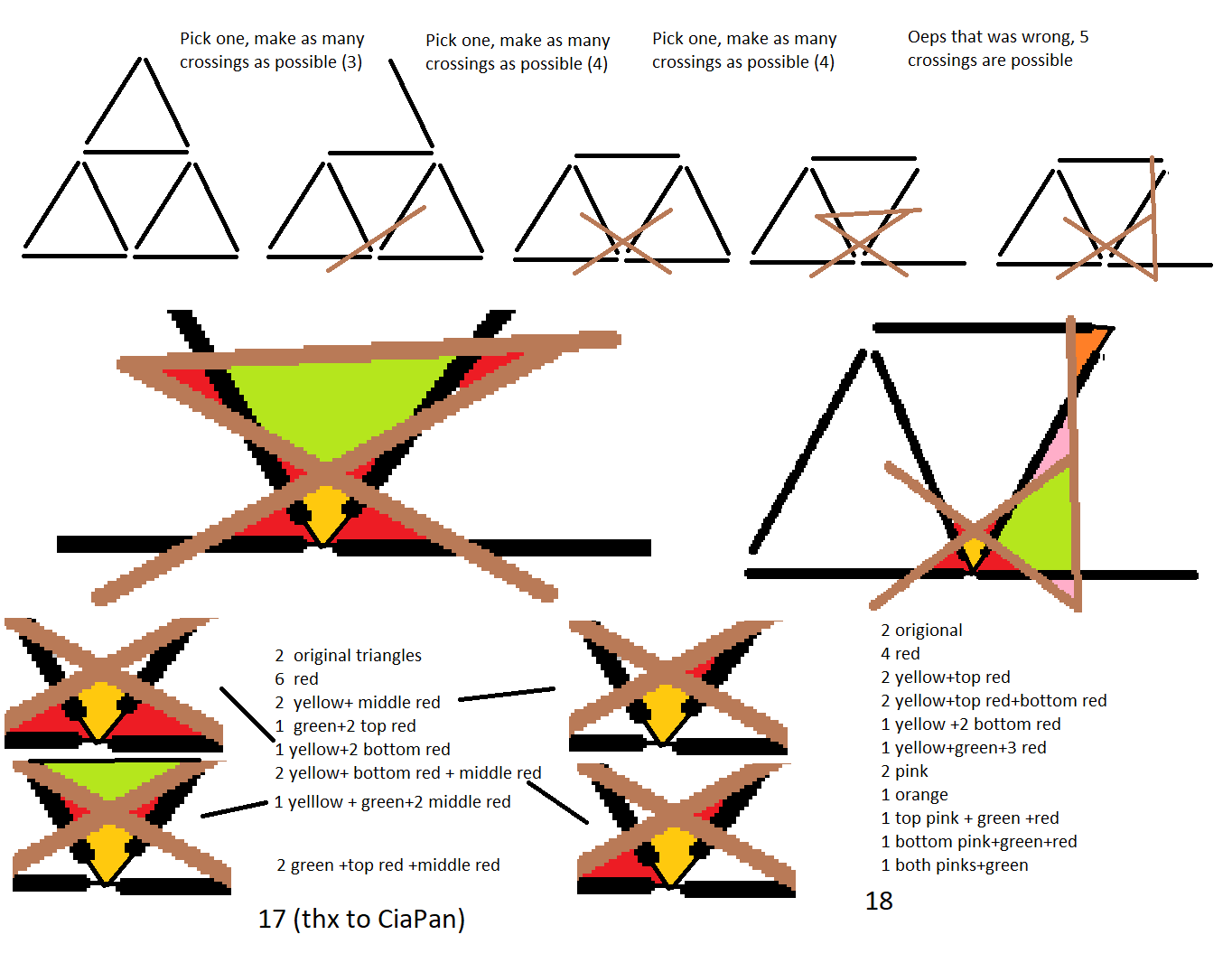
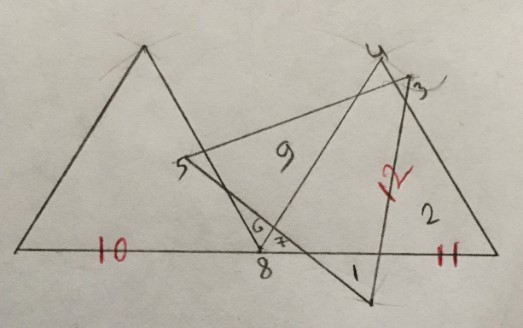
So far what I attempted to do is to move the three matches in the center and arrange them so that the triangle is split in half and the another half. But on counting the most triangles I could get were 9, but this doesn't check with the official answer.

Is there a strategy or method I can use to guess or try with more success to get the right answer? Can someone help me with a step by step approach and a drawing to see where to put those matches?

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ Does the question requires triangles to be equilateral triangles? $\endgroup$
    – tsh
    Commented Nov 20, 2020 at 8:51
  • $\begingroup$ @tsh Apparently it seems that it can be of any kind. But looking on the official solution it seems that the triangles might be equilateral. Did you also got to 10 by using this condition?. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 21, 2020 at 9:10
  • $\begingroup$ We have enough evidence that the specific book has many low-quality puzzles - some are underspecified, some are "guess the author's intent", and one was just plain wrong. I'd really appreciate if you stop posting puzzles from that book. $\endgroup$
    – Bubbler
    Commented Nov 21, 2020 at 12:54
  • $\begingroup$ @Bubbler I'm sorry if my humble book has gave you the sense that it should be discarded right away. Its the only source which I do have. Despite having some errors here and there I still believe its a good book. I will try to verify and prove next time that there is less frequency in errors, have me patience. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 22, 2020 at 20:50

6 Answers 6

Reset to default
2
$\begingroup$

Either I am missing something , or the answers are wrong:

enter image description here

Improve this answer
$\endgroup$
7
  • $\begingroup$ I've checked with the official anwer from my book and states to be 10. However I believe your solution should be the right one though. Does it exist any way to force it to be 10? By the way I attempted to follow your sketch but I don't know where do they come those two yellow triangles. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 19, 2020 at 22:34
  • $\begingroup$ 15 on the left should be 17, IMHO – increase by 2 × (green + top red + middle red). $\endgroup$
    – CiaPan
    Commented Nov 20, 2020 at 12:51
  • $\begingroup$ @ChrisSteinbeckBell It seems like this question is poorly-written. I'd be surprised if there was a way to get it besides "read the mind of the author". $\endgroup$
    – Deusovi ♦
    Commented Nov 20, 2020 at 16:31
  • $\begingroup$ @Retudin I'm sorry but can you please add a clarification for the green color and the 18 triangles figure?. It's hard to count well if I'm not getting your intended interpretation. I'm stuck on the part where it says 1 yellow plus 2 bottom red and the combinations including green. Are you referring to the green colored match sticks? The individual colors are easy to understand but on the combinations where the green appears I'm getting tangled with the interpretation. Can you help me with this part please?. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 21, 2020 at 9:03
  • $\begingroup$ @Retudin This also applies to the 17 triangle figure. For example 1 yellow plus green plus 2 middle red, the green colored match stick makes an cross isn't a triangle, or am I missing something?. Can you clear this doubt please?. Again your solution is very clever but I'm having a hard time at following it. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 21, 2020 at 9:04
1
$\begingroup$

With the restrictions @AxiomaticSystem has proposed

  1. triangles must be regular and
  2. no loose ends allowed

the best I can do is in fact

10 enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ That's a nice variation of what AxiomaticSystem proposed. Perhaps have you used inkscape to draw your figure?. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 22, 2020 at 20:34
  • $\begingroup$ @ChrisSteinbeckBell No, that's libre office draw. Not great but does the job $\endgroup$
    – Paul Panzer
    Commented Nov 22, 2020 at 21:10
1
$\begingroup$

Here is a solution for equilateral triangles.

A solution to this puzzle

If I didn't count incorrectly, there should be

13

equilateral triangles.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for that clever answer. Honestly I thought that the greatest number allowing certain conditions it could be 10 but even using equilateral triangles you could obtain more. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 22, 2020 at 20:35
1
$\begingroup$

The best I can do is three equilateral triangles The total number of triangles is 12.

nov22

Improve this answer
$\endgroup$
4
  • $\begingroup$ You moved 6 matches, not 3. $\endgroup$
    – Jaap Scherphuis
    Commented Nov 21, 2020 at 23:45
  • $\begingroup$ @Jaap scherphuis.I tried again and now only 3 matches are moved. $\endgroup$
    – Vassilis Parassidis
    Commented Nov 22, 2020 at 3:34
  • $\begingroup$ @VassilisParassidis If you don't mind, can you increase the resolution of your drawing?. I cannot distinguish very well the numbers and the notations which you have made in the picture. Maybe you can use paint or some software for a clearer version. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 22, 2020 at 20:42
  • $\begingroup$ @Chris Steinbeck Bell. Better now? $\endgroup$
    – Vassilis Parassidis
    Commented Nov 22, 2020 at 21:36
0
$\begingroup$

The best I could do

1

12 triangles solution

Improve this answer
$\endgroup$
1
  • $\begingroup$ I don't know, but are you triangles drawn in scale?. Because I feel one side is bigger than what the size of the other match sticks allow. I mean the upper triangle in the figure looking from the right. The two match sticks appear to be bigger than the others. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 22, 2020 at 20:39
0
$\begingroup$

I believe the missing information is either that

the matches have to lie on an implied triangular grid, or that the endpoints of the moved matches have to lie on other matches.

Either way, we have this suggestive ten-triangle diagram:

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ It seems that this might be the intended solution or perhaps the author forgot to include the paragraph which you mentioned. $\endgroup$
    – Chris Steinbeck Bell
    Commented Nov 21, 2020 at 9:06
  • $\begingroup$ You need even more restrictions. Yours still allow the brown / to be moved a little to the right for more triangles. $\endgroup$
    – Retudin
    Commented Nov 21, 2020 at 11:12
  • $\begingroup$ The endpoint of the bottom right match doesn't conform, does it? $\endgroup$
    – Paul Panzer
    Commented Nov 21, 2020 at 19:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.