Can you fold a square into a square of one-third the area

Question

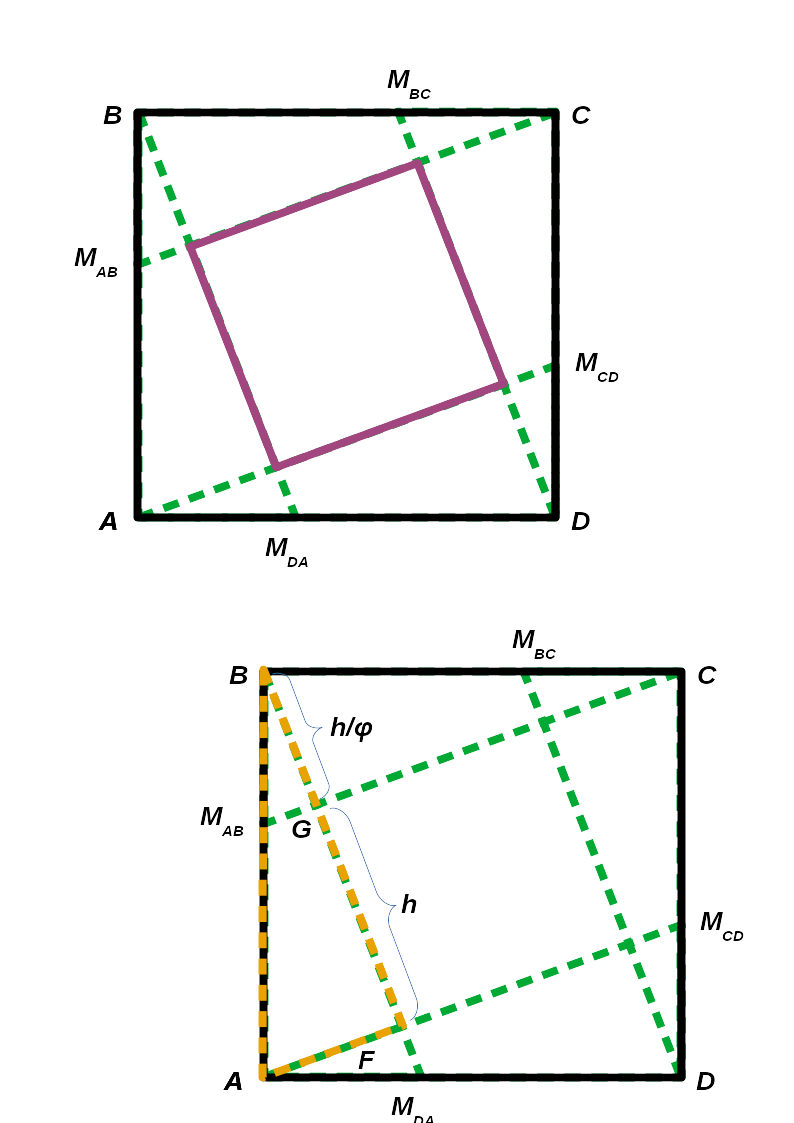
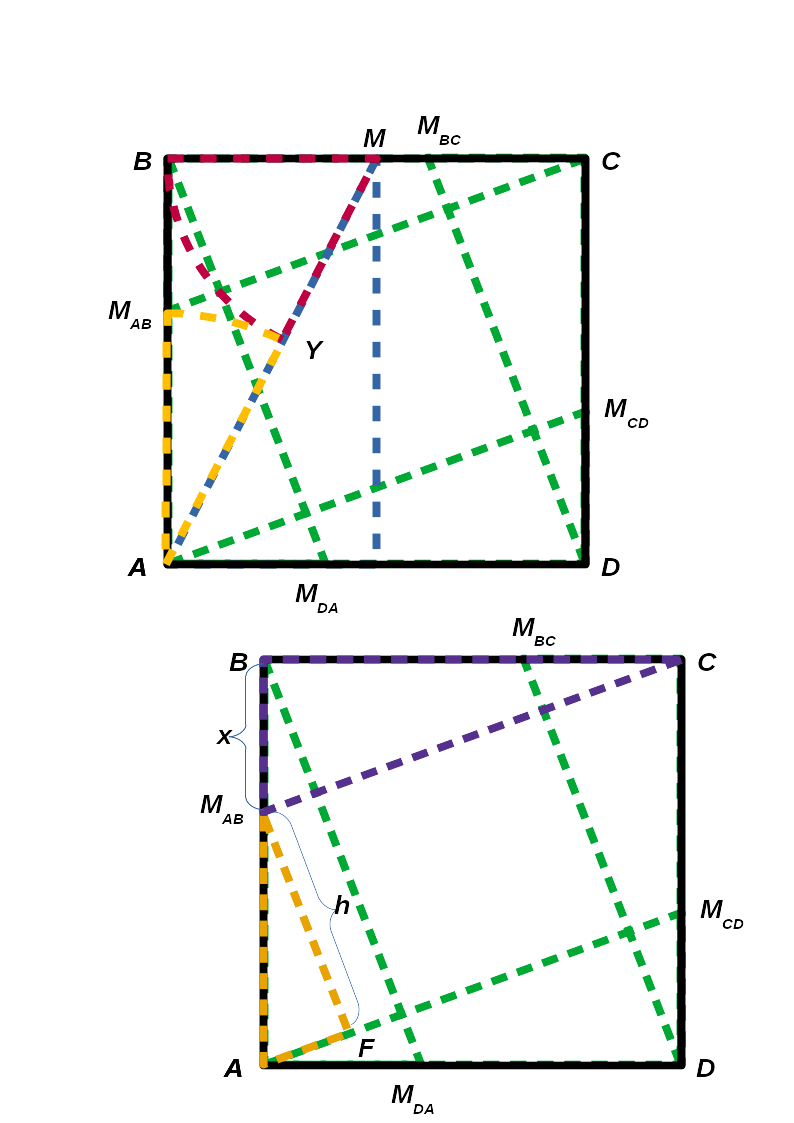
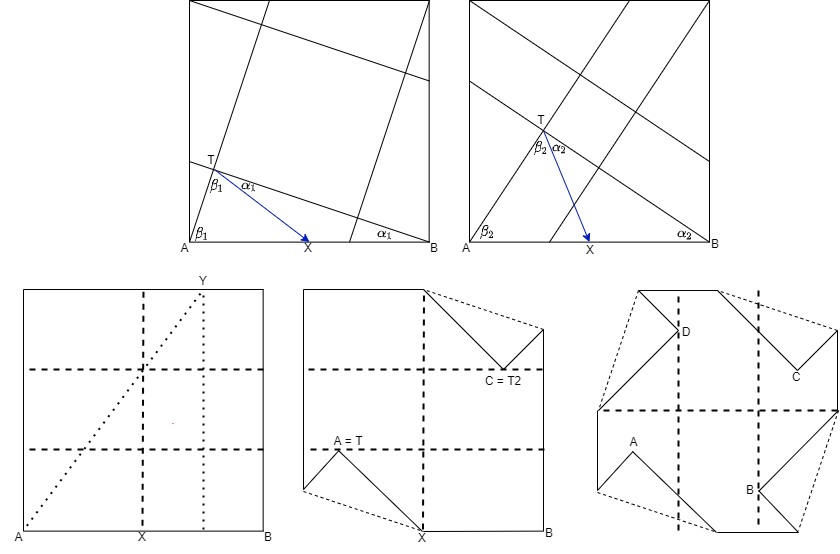
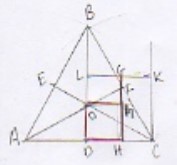
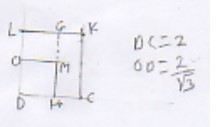
I do not love origami, but Mitsuko gave me an idea for a extremely hard and (not that?) beautiful puzzle. I'm really curious whether anyone here can solve it.

So here's the puzzle. You are given a large perfectly square piece of paper with no marks on it. With this square, you have to make a square of exactly one-third the area of the original square. You are given no tools such as a ruler or scissors, and all you can do is fold the paper. How do you solve this?

EDIT: Though I did not explicitly ask it, I would like a 'beautiful' solution, which for me means just using geometry not algebra. I just realized that such a solution is possible. A simple explanation, a centered solution and little folds are also a plus i.m.o. but if I soon get a correct geometry based solution (like in the one-fifth problem I based this one on), I will accept that one instead of one of the correct algebra based ones I already have or may get.

Note: There are several answers now with different approaches. I can only accept one, but I'd suggest reading a few least, if you are interested in ways to solve this.

Answer 1

This one uses (very likely) minimal number of folds.

EDIT: Removed the reassembly-based proof and added a more formal proof.

The steps

Fold vertically in half to produce $EF$, and fold through $C$ so that $D$ is placed over $EF$. Then $DG$ is one side of the 1/3 square.



Fold through $BD$ and fold through $G$ perpendicular to $AD$, and then unfold both folds. Then we get the square $DGHJ$.



We used 4 folds in total.

In order to fold into a square, fold $GH$ first, then $DH$ to find $J$, then $HJ$, and then unfold $DH$. This uses 5 folds in total.

The proof

Think of the mirror image of the first part:



We can observe that the triangles $CD'F$ and $DC'F$ are congruent (have same shape and size) because they are mirror images of each other. This means $D'F = C'F$ and therefore $D' = C'$. By the property of paper folding, $D'C = DC = C'D$, and since $D' = C'$, the triangle $DCD'$ is an equilateral triangle. So the angle $DCD'$ is $60^\circ$. Again by the property of paper folding, the angles $DCG$ and $D'CG$ are equal, which means they are both $30^\circ$. Since $\tan 30^\circ = 1/\sqrt3$, it follows that $DG = (1/\sqrt3) DC$, and $DG^2 = DC^2/3$, thus finishing the proof that the new square has one third area of the original.

Answer 2

The golden ratio has been described as something popping up all over nature, science and arts, so we shouldn't be too surprised at bumping into it here.

Our strategy is based on the following configuration (first picture top). We want to make rotationally symmetric folds from each corner (green lines). Those will delineate a smaller square in the center, so all we need to do is adjusting the angle of the green lines such that the area of the center square becomes $1/3$
This will happen precisely when the area $h^2$ of the center square doubles the area of the yellow triangle (first picture bottom). Define $\phi$ as the ratio $\frac {\overline{FG}}{\overline{GB}}$. The area of $AFB$ is $\frac{h^2(1+1/\phi)}{2 \phi}$ because it has perpendicular sides $\overline{FB} = h + h/\phi$ and $\overline{FA} = h/\phi$ ($\overline{FA} = \overline{GB}$ by symmetry). Solving for $\phi$ that we get $\phi^2=1+\phi$ confirming that $\phi$ is indeed the golden ratio. By the intercept theorem the intersection points $M_{AB}$ etc. divide the sides also by $\phi$.

Implementation in terms of actual folding. With the benefit of hindsight I notice that this is very similar to textbook construction of golden ratio:

Fold the square $ABCD$ in the middle horizontally or vertically. WLOG let $BC$ and $DA$ be the sides cut in half. Fold from corner $A$ to the midpoint $M$ of nonadjacent side $BC$. Mark the crease $MA$ half a unit from $M$. (By folding either $BM$ or $CM$ onto $MA$.) Call this point $Y$. The distance $AY$ is $\frac{\sqrt 5 - 1} 2$. Transfer this distance to the $AB$ side (measuring from $A$). Call this point $M_{AB}$ Fold $M_{AB}C$. Using the edge this creates we can directly fold the perpendicular sides and afterwards also the fourth.

Pictures

Alternative proof that $h^2 = 1/3$. I'll leave it here since there are actually people who prefer this kind of technical approach over more "wordy" ones.

We need to show the distance $h$ between opposite creases is $\sqrt{\frac 1 3}$. Let $F$ be the nearest point to $M_{AB}$ on $M_{CD}A$. Then triangles $M_{AB}BC$ and $AFM_{AB}$ are similar. Write $x=\overline{M_{AB}B}$ then $\frac {1-x}{h} = \sqrt{1+x^2}$ by similarity. Setting $h=\sqrt{\frac 1 3}$ and solving for $x$ yields
$\frac {1}{3} = \frac{1+x^2-2x}{1+x^2}$
$0 = 2 + 2x^2 - 6x$
$(x-\frac 3 2)^2 = \frac 5 4$
with solutions
$x = \frac {3 \pm \sqrt{5}}{2}$
of which only
$x = \frac {3 - \sqrt{5}}{2}$ is within size of the given square. What we have constructed and used above is $1-x = \frac {\sqrt{5} - 1}{2}$

Answer 3

It isn't too hard if we analyze it mathematically.

Observe that

Assume that the original square has side length 1 and area 1. Then the target square has area $1/3$, and therefore side length $1/\sqrt3 = \sqrt3/3$.

Based on this, we plan the strategy as follows:

First construct the length $\sqrt3/2$ using the equilateral triangle, and then construct $2/3$ of that.

First part:

Fold the paper vertically in half, so that $B$ overlaps with $A$ and $D$ overlaps with $C$:

A-------B      A---E---B
|       |      |   |   |
|       |      |   |   |
|       |  =>  |   |   |
|       |      |   |   |
|       |      |   |   |
C-------D      C---F---D

Then fold along a line passing through $F$ so that $E$ overlaps with the line $AC$. Let's call the new point $G$:

A---E
|   |
G   |
|\  |
| \ |
|  \|
C---F

Then $CF=1/2$ and $FG=1$, so $CG=\sqrt3/2$.

Second part:

Ignore all the folds in the first part except the point $G$.

A-------B
|       |
G       |
|       |
|       |
|       |
C-------D

Fold horizontally through $G$ (it can be done by folding through $G$ while putting $A$ on the line $GC$), and ignore the part above $G$. Now fold horizontally three times so that $GC$ is evenly divided into four segments:

G-------+
|       |
J-------J'
|       |
H-------H'
|       |
K-------K'
|       |
C-------D

Then fold a line passing through $C$, so that $G$ overlaps with $JJ'$. Mark the intersection of line $G'C$ with $HH'$, and unfold it back. We just got a trisection of the line segment $GC$; the line segment $CX$ has exactly $2/3$ length of $GC$, and therefore length $1/\sqrt3$.

G--------+       G--...
|        |       |
J-----G'-J'      |
|    /   |       X
H---X----H'  =>  |
|  /     |       |
K-/------K'      |
|/       |       |
C--------D       C--...

So we've just got one side of the target square. Finally,

Fold through the diagonal $BC$ so that $X$ overlaps with $CD$. Mark the overlapping point of $X$ as $Y$. Then fold horizontally through $X$ and vertically through $Y$. Then we get the wanted square on the lower left corner of the original sheet of paper.

A-----+-B
|     | |
X-----+-+
|     | |
|     | |
|     | |
C-----Y-D

Answer 4

I found an i.m.o. more elegant answer myself. And I gave up hope that with the good answers already submitted someone else will post something similar. Thus my answer:

Observation 1:
We can enclose a smaller square of any size in a square using 4 identical triangles like TAB in the first two drawings.
Observation 2:
We can draw a line from the top T of such a triangle to its base using the same angles, since the top has an angle of 90 degrees. When that line crosses the line AB (at point X) we can conclude that TX = XB and TX = XA because of the identical angles alpha and beta respectively.
Since XA+XB is 1: TX is 1/2 for any such smaller square, and X is the midpoint of a side.
Observation 3:
The volumes of the 4 triangles must be 2/3 if the volume of inner square is to be 1/3. That means each individual triangle has volume 1/6, and thus that the distance between the top and the base is 1/3.

Construction step 1:
Like in the third drawing, one can get the required 1/2, 1/3 and 2/3 lines as follows: Fold all for the 1/2 line, then fold half for the 3/4 line, then fold from 3/4th of a side to the opposing corner. This line will cross the half line at 1/3. Use that now marked point to fold the 1/3 and 2/3 line.
Construction step 2:
Fold a line from the midpoint X such that A is on the 1/3 line, like drawing 4. >!Now A covers T exactly. Do the same thing at the opposing corner.
Construction step 3:
Turn the paper a quarter and repeat step 1 and 2. All the corners of the paper are folded onto the corners of the 1/3-volume smaller square. (as in the last drawing)
Construction step 4:
Fold the lines AB,BC,CD and DA (of the last drawing) to the the asked for 1/3 volume square.

Answer 5

[

Let's construct an equilateral triangle $ABC$ where $AB=4$ and let's draw the three midlines $BD, CE, AF$ and the segment $DC=2$. From point $C$ draw perpendicular line $CK=DC$. From point $D$ let's obtain a segment $DL=DC$ and draw the line $LK$. The quadrilateral $DCKL$ is square by construction. From point $D$ let's take a segment $DH=OD$ and from point $H$ draw a perpendicular and obtain on that line a segment $HM=OD$ and draw the straight line $OM$. The quadrilateral $ODHM$ is a square. Extend the line $HM$ until it cuts the line $LK$ at point $G$. From the point of intersection $O$ of the three midlines, the distance to the vertices of the triangle $ABC$ is two-thirds of $BD$. $(BD)^2=4^2-2^2=12$ and $BD=2√ 3$. So $OD=(2√ 3)/3$, but $(2√ 3)/3=2/√ 3$. So the area of the square $ODHM=(2/√ 3)^2=4/3$. This result tells us that the area of the square $ODHM$ is equal to one-third of the area of the square $DCKL$. Let's fold the rectangle $GHCK$ under the rectangle $LDHG$. After that let's fold the rectangle $LOMG$ under the square $ODHM$. With two folds we were able to achieve what the question was asking.