9
$\begingroup$

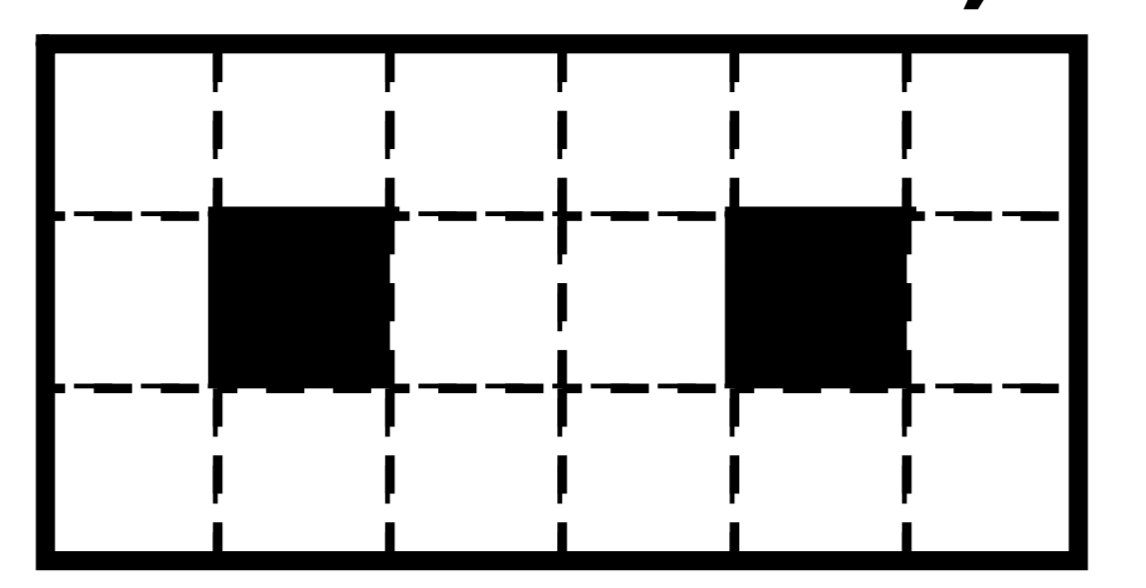
enter image description here

A 3 x 6 rectangle has 2 holes in it as shown. Can you cut it into 3 polyominoes with different areas so that they can form a square? The pieces can’t be flipped when they form the square and two solutions are the same if they are identical after rotation and/or reflection.

Improve this question
$\endgroup$
7
  • $\begingroup$ If diagonal cuts are allowed, are they really polyominoes? $\endgroup$
    – Rand al'Thor
    Commented Jun 27, 2020 at 21:00
  • 1
    $\begingroup$ Oh sorry, you can’t make diagonal cuts. @Randal'Thor $\endgroup$
    – Display maths
    Commented Jun 27, 2020 at 21:08
  • 1
    $\begingroup$ Yes you’re right @hexomino $\endgroup$
    – Display maths
    Commented Jun 27, 2020 at 21:08
  • $\begingroup$ You say "a rectangle has 2 holes". Is that the statement of the problem, and the picture is just one possible example? Or is the picture the only one you're asking about? $\endgroup$
    – msh210
    Commented Jun 27, 2020 at 21:23
  • $\begingroup$ If we can't flip pieces, how do we get reflections? $\endgroup$
    – msh210
    Commented Jun 27, 2020 at 21:25

2 Answers 2

Reset to default
8
$\begingroup$

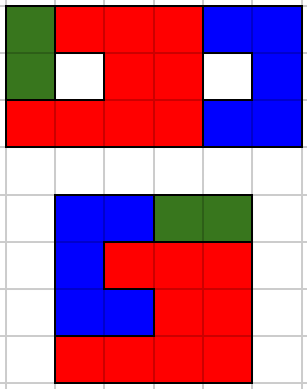
For this one, you don't even need to rotate the pieces:

enter image description here

Text version:
rrbggg
r br g
rrrrgg
becomes
gggb
rrgb
rggr
rrrr

And the final ones, which are closely related:

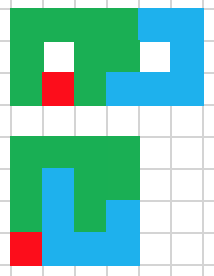
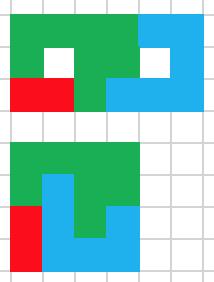
enter image description here    enter image description here
Text version:
ggggbb ggggbb
g gg b g gg b
grgbbb rrgbbb
becomes
gggg gggg
gbgg gbgg
gbgb rbgb
rbbb rbbb

Improve this answer
$\endgroup$
5
  • $\begingroup$ Nice! There is one left. $\endgroup$
    – Display maths
    Commented Jun 28, 2020 at 12:53
  • $\begingroup$ @Displaymaths found it! Nice puzzle! $\endgroup$
    – Glorfindel
    Commented Jun 28, 2020 at 13:05
  • $\begingroup$ Oh sorry, there is a last one. $\endgroup$
    – Display maths
    Commented Jun 28, 2020 at 13:11
  • $\begingroup$ Oh, of course, it's a small variation. I'll add it in a few minutes. $\endgroup$
    – Glorfindel
    Commented Jun 28, 2020 at 13:13
  • $\begingroup$ There are only two more if you allow flipping, if you want to get those as well since you are on a roll... $\endgroup$
    – theonetruepath
    Commented Jun 28, 2020 at 16:05
7
$\begingroup$

Here's one solution:

enter image description here

Other solutions may be possible.

Improve this answer
$\endgroup$
1
  • $\begingroup$ True, there are more. $\endgroup$
    – Display maths
    Commented Jun 27, 2020 at 22:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.