Smallest square with pentominoes

Question

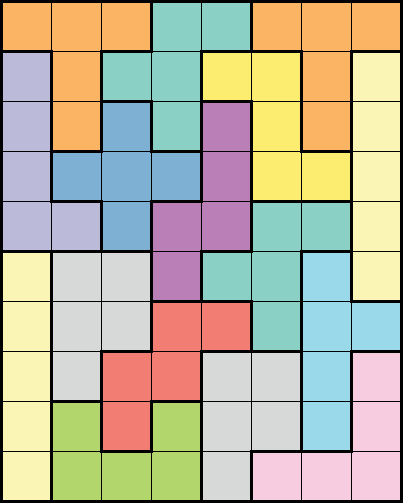
I created a puzzle by myself for the first time and this is it:

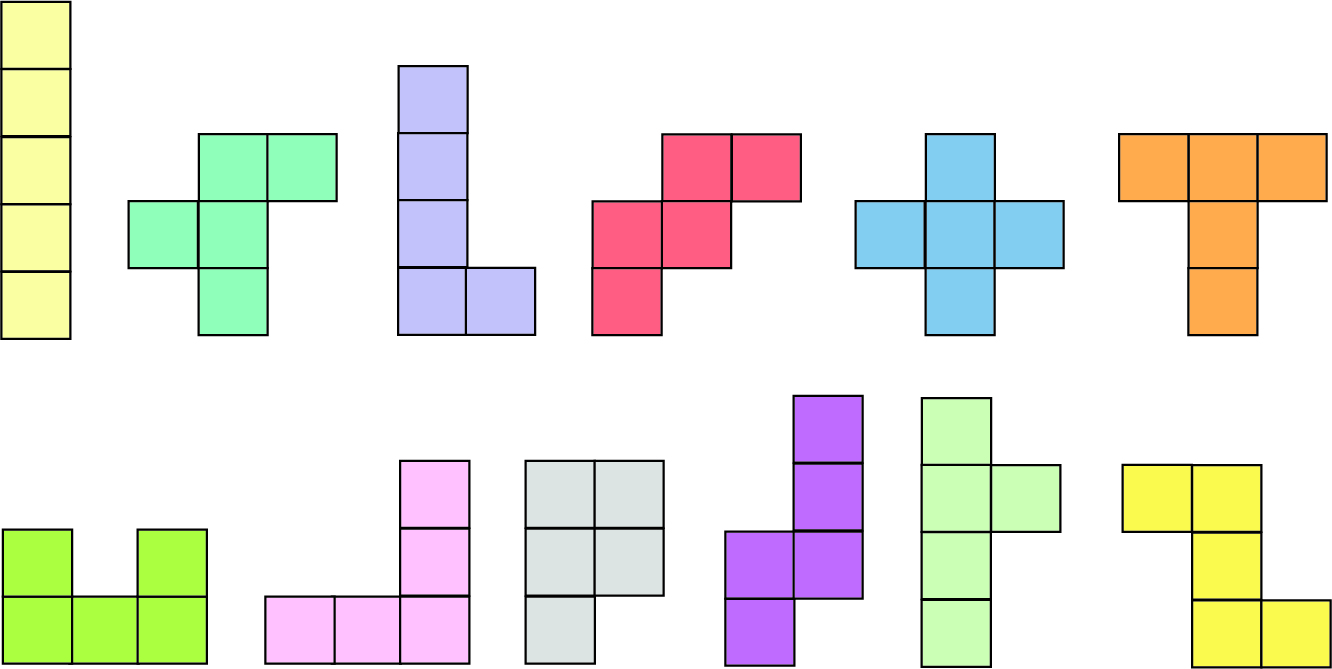Using all the pentominoes from the figure at least once, what is the least size of square (or rectangle, whichever comes first) that we can create?

You cannot consider any holes here. But, changing the orientation of the pieces are allowed.

Moreover, how does the problem look like if changing the orientation of the pieces are not allowed?

Hi welcome to PSE! puzzling.stackexchange.com/questions/47070/… Does this answer your question? — PDT, Commented Sep 4 at 17:29
Are there empty squares (or holes) allowed? I this case square of 9x9 can is possible from 7x9 solution of @PDT link. 8x8 if exists contains 4 "holes". — z100, Commented Sep 4 at 17:43
10x10 as smallest possible square without hole is a trivial extension from 6x10 solution using 4 times two horizontal I elements. — z100, Commented Sep 4 at 17:52
The fixed-orientation version has solutions with fewer than 20 pieces. I think you should stick with that, as allowing rotation makes this too similar to the basic pentomino puzzle. — Daniel Mathias, Commented Sep 6 at 2:52

Daniel Mathias · Accepted Answer · 2024-09-10 03:23:13Z

1

Smallest rectangle with pieces having fixed orientation:

16 pieces

answered Sep 10 at 3:23

Daniel Mathias

17k2 gold badges36 silver badges71 bronze badges

1

$\begingroup$ I did not check for solutions allowing rotation. There may be a 5x12 or 6x10 rectangle with these as one-sided pentominoes. $\endgroup$
– Daniel Mathias
Commented Sep 10 at 3:25

Add a comment |

1 Answer 1