What is a Lovely Word™?

Question

_{This is in the spirit of the What is a Word/Phrase™ series started by JLee with a special brand of Phrase™ and Word™ puzzles.}

If a word conforms to a special rule, I call it a Lovely Word™.

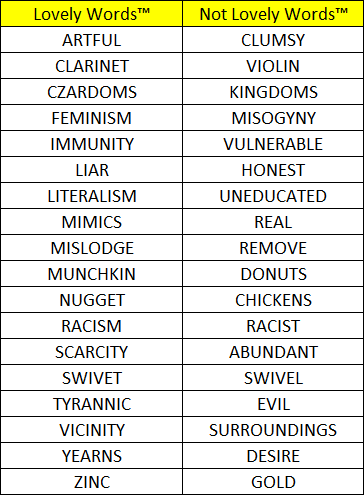
Use the following examples below to find the rule.

And, if you want to analyze, here is a CSV version:

Lovely Words™,Not Lovely Words™
ARTFUL,CLUMSY
CLARINET,VIOLIN
CZARDOMS,KINGDOMS
FEMINISM,MISOGYNY
IMMUNITY,VULNERABLE
LIAR,HONEST
LITERALISM,UNEDUCATED
MIMICS,REAL
MISLODGE,REMOVE
MUNCHKIN,DONUTS
NUGGET,CHICKENS
RACISM,RACIST
SCARCITY,ABUNDANT
SWIVET,SWIVEL
TYRANNIC,EVIL
VICINITY,SURROUNDINGS
YEARNS,DESIRE
ZINC,GOLD

Addition 1:

A. I've found a second answer to the problem, one that I do not want. I will put some counter-examples here.

The following words are Not Lovely Words™: AW, BOWL, CARS, QUEENS.

B. In the chart, MUNCHKIN is listed as a Lovely Word™. This is disputable.

Addition 2:

In the chart, MISLODGE is listed as a Lovely Word™. Whether or not this is disputable is disputable.

Hint 1:

All Lovely Words™ will have an even number of letters. All listed counter examples also have an even number of letters to give you more to work with.

Hint 2:

Try the following:
- Can you find the abnormality in one of the Lovely Words™?
- Can you find the two letters with mutual everlasting love?

The rule isn't complex, although it's not exactly as simple as a doornail. You could determine whether or not a word is a Lovely Word™ by staring at it for perhaps ten seconds.

Answer 1

[EDITED to replace a wrong answer -- thanks, greenturtle3141 -- with what I hope is now a right one.]

I think a word is Lovely (tm) when

it is made up of a succession of compatible pairs of letters, where two letters are compatible if, when written as capitals, they are homeomorphic (i.e., topologically equivalent).

This depends a bit on

the typeface used; in particular, G may either be homeomorphic to a single line or have a bifurcation. Clearly here the latter is the case because it occurs in a pair with E.

This criterion is almost equivalent to

counting the number of "ends", but note that B and O both have no ends but fail to be homeomorphic (they have different numbers of loops; a topologist would observe that their fundamental groups or first homology groups differ). Similarly for A/R (which have loops) versus, say, C (which doesn't).

Lots of things are disputable because of

serifs. E.g., does I have two or 4 ends?

The abnormality mentioned in the second hint is

in the word MISLODGE, where the G is actually in a different typeface or something of the kind -- compare it with the ones in NUGGET. (That should have been a hell of a giveaway and I'm annoyed I didn't notice it until writing this.)

Perhaps the letters with "mutual everlasting love" referred to in the second hint are

P and Q, the only two letters with just one end, which can therefore only ever occur with one another.

Or they might be

D and O, which are the only two homeomorphic to circles. These, unlike P and Q, can actually occur next to one another in a word.