5
$\begingroup$

As the title says it is a compete the sequence.

My thoughts:

From all the circle ones that aren't a circle, you can make a circle by rotating the pieces. From all the triangle ones that aren't a triangle, you can make a triangle by moving one line. But I can't figure out a rule for the square-ish things. (Of course the rules I came up with for the circle and triangle might be wrong.)

Improve this question
$\endgroup$

4 Answers 4

Reset to default
7
$\begingroup$

I'll go with:

The top right answer

Why?

There's a triangle, a circle and a 'U' shape. They each appear both 'normal', once with both halves mirrored along a vertical axis, and once with the left side intact and the right rotated 180 degrees.

Based on what's there already:

the three states for triangle and circle, and both the normal and mirrored state of the 'U' shape

what we need is:

left side of the U intact, right side rotated 180 degrees.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ But if we keep the left side intact, how does the left side of the circle flip when going from second row to third row? $\endgroup$
    – user265554
    Commented Sep 22, 2015 at 14:34
  • $\begingroup$ Because we started from the circle, not from the double-half-circle. $\endgroup$
    – Hellion
    Commented Sep 22, 2015 at 14:57
  • $\begingroup$ These rules apply to the circle objects but not to the triangle objects $\endgroup$
    – user265554
    Commented Sep 22, 2015 at 15:06
  • $\begingroup$ What @Helion said: the transformations I described are all starting from the base form, not from an already transformed state. $\endgroup$
    – Tim Couwelier
    Commented Sep 22, 2015 at 15:06
  • $\begingroup$ @user265554 Yes they do. The original triangle is on row 2 column 2. The varation where both halves are mirrored is on row 1 colum 3. The variation where the left half stays untouched and the right half is rotated 180 degrees is row 3 column 2. If you're going to downvote for an error on my part, do make sure it's an actual error. $\endgroup$
    – Tim Couwelier
    Commented Sep 22, 2015 at 15:09
1
$\begingroup$

I think it is the,

Third one in the first row
There is a circle, a mirrored half of a triangle, and an upside down T in each each row

The first row is correct

The second row, you cut it vertically and switch the sides

The third row, you cut it down the middle vertically and flip the right side over the x axis and the left side over the y axis

The reason it isn't a circle, triangle, and U is because of the second row. There is no way to move the triangle and U to get the circle while preserving their shape, therefore, they need to move the same way the circle will which gives you the shapes in the first row. the rows do not have the same moves to get to the end result although they do build on each other in number of moves. The first row has 0 moves, the second has 1, and the third has 2.

Improve this answer
$\endgroup$
6
  • $\begingroup$ But how does the circle's left side change and not the right side when going from the second row to the third? $\endgroup$
    – user265554
    Commented Sep 22, 2015 at 14:36
  • $\begingroup$ @user265554 the rows are unrelated. You don't need to know how the second row was flipped to get to the third row. The first two rows are there to help you figure out the shapes. If it wasn't for the second row it could be a circle, triangle, and U shape rather than an circle, mirrored half of a triangle, and an upside down T $\endgroup$
    – SirParselot
    Commented Sep 22, 2015 at 14:39
  • $\begingroup$ But if you take the whole circle from the first row, and flip the right side over the x-axis and the left side over the y-axis, you get a shape that is the first shape from the third row but which has been rotated 180 degrees $\endgroup$
    – user265554
    Commented Sep 22, 2015 at 14:47
  • $\begingroup$ @user265554 That is valid but what's your point? If you follow that rule for each row you will get a different second row while the first and third rows match. My point is that there is no one set of moves that you can apply to each row and get the same shapes (Orientation matters) therefore each row will have a different set of moves or none at all $\endgroup$
    – SirParselot
    Commented Sep 22, 2015 at 14:55
  • $\begingroup$ If the rules for getting the third row from the first row are to flip the the right side over the x-axis and the left side over the y-axis, you can't get the first object of the third row from the second object of the first row with these rules. $\endgroup$
    – user265554
    Commented Sep 22, 2015 at 15:03
0
$\begingroup$

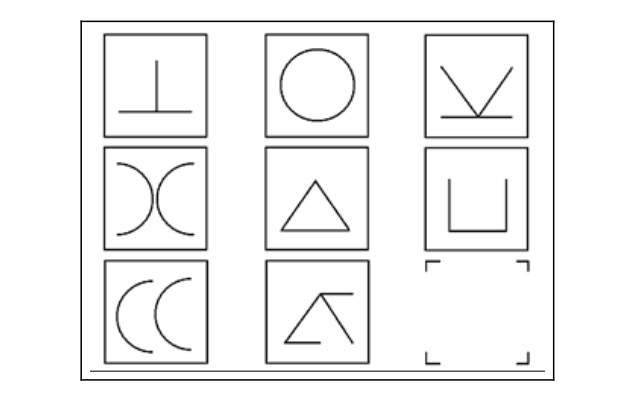
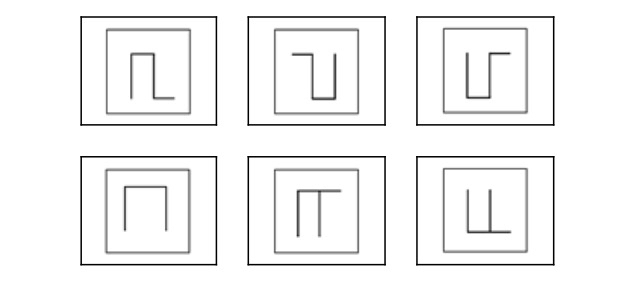
It is

the third one in the first row.

Let's look at the triangles:

Row 2, Column 2: First picture: the intact triangle

Row 3, Column 2: Second picture: right half of the triangle has been rotated horizontally and vertically

Row 1, Column 3: Third picture: other half of the triangle has been rotated horizontally and vertically, afterwards that whole picture is again rotated horizontally

And now for the circles

Row 1, Column 2: First picture: the intact circle

Row 3, Column 1: Second picture: half of the circle has been rotated horizontally and vertically

Row 2, Column 1: See: half of the circle has been rotated horizontally and vertically, and then again rotated horizontally

Aaaand finally the .. squarish things?

Row 2, Column 3: intact U

Row 1, Column 1: again, rotating the right half vertically and horizontacally

That is the one in the new Row 1, Column 3

Row 1, Column 1: final picture

You can also see a pattern in the rows and columns:

Triangles

Rows: 2, 3, 1

Columns: 2, 2, 3

Circles

Rows: 1, 3, 2

Columns: 2, 1, 1

Squares

Rows: 2, 1, 3

Columns: 3, 1, 3

So all in all it's this:

Rows are: 1,2,3 - 1,2,3 - 1,2,3

Columns are: 1,1,2 - 2,2,3 - 3,3,1

Cheers.

Improve this answer
$\endgroup$
-1
$\begingroup$

1.3
Divide image in half, flip half; and reconstruct the whole image.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Welcome to PSE. Please hide your answers in spoilers in the future. $\endgroup$
    – rhsquared
    Commented Jan 18, 2019 at 16:33
  • 1
    $\begingroup$ Welcome to Puzzling.SE! In the future, please use the ">!" spoiler notation for answers. $\endgroup$
    – Brandon_J
    Commented Jan 18, 2019 at 16:33

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.