Minimally clued 5x5 Hidato

Question

_{Puzzle attribution: Me}

---

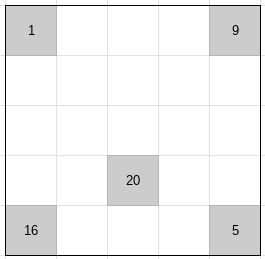
Goal of Hidato:

Fill in a grid with a series of consecutive numbers that connect each other orthogonally or diagonally

To get the green checkmark $\color{green}✓$:

• Solve the puzzle (showing solution process is optional) or show that no solution exists/the solution is not unique

The puzzle:

+---+---+---+---+---+
| 1 |   |   |   | 9 |
+---+---+---+---+---+
|   |   |   |   |   |
+---+---+---+---+---+
|   |   |   |   |   |
+---+---+---+---+---+
|   |   |20 |   |   |
+---+---+---+---+---+
|16 |   |   |   | 5 |
+---+---+---+---+---+

Answer 1

The solution is

not unique. In the grid below, the 15 and 25 can be entered in the empty spots in either order.

 1  12 11 10 9
 13 2  23 22 8
 14 24 3  21 7
 .  .  20 4  6
 16 17 18 19 5

You can also reverse 21-25 as follows (and then you can still swap 25 and 7):

 1  12 11 10 9
 13 2  23 24 8
 14 22 3  25 7
 15 21 20 4  6
 16 17 18 19 5