1
$\begingroup$

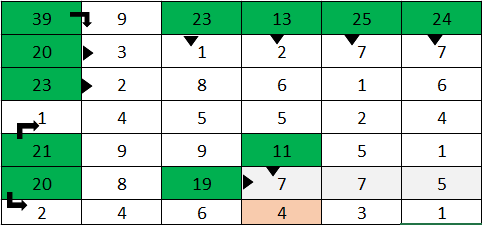
I'm trying to solve the following quiz from my daily quiz calendar (I have it in paper so I cannot give a link). It's like a crossword puzzle but with numbers.

The empty cells should be filled with numbers from 1-9 and should be the green cells in sum.

19 = 7+7+5 is prefilled. And the 4 in 11=7+4 is filled from me.

I tried to solve it with a linear equation system but I failed.

Does anybody have tips on how to solve this?

enter image description here

Improve this question
$\endgroup$
10
  • $\begingroup$ Welcome to Puzzling! Please note that for puzzles you found elsewhere proper attribution is required. $\endgroup$
    – Glorfindel
    Commented Feb 15, 2021 at 8:54
  • $\begingroup$ This bears many similarities to a Kakuro puzzle, although here you can clearly have the same number twice in a sum, as evidenced by the prefilled adjacent 7's and the 6-box '20' on the bottom row (21 is the smallest number that can be made from 6 separate integers: 1+2+3+4+5+6). You might find some Kakuro logic is still widely applicable here - you may find it useful to look that up... $\endgroup$
    – Stiv
    Commented Feb 15, 2021 at 8:58
  • $\begingroup$ I tried to do it with sums like in Kakuro but there were too many possibilites for me. And I didn't know how to start. And because one can have the same number more than one time it seems that there are infinitely many possibilities to sum up the single numbers. $\endgroup$
    – jane doe
    Commented Feb 15, 2021 at 9:04
  • 2
    $\begingroup$ @Randal'Thor If the digits in a sum had to be distinct, the bottom row could not be filled at all, since it has 6 digits and 1+2+3+4+5+6=21>20. $\endgroup$
    – Jaap Scherphuis
    Commented Feb 15, 2021 at 9:33
  • 1
    $\begingroup$ The cells immediately to the right of the 21 and 20 clues are only in the vertical 39 clue, so without any further constraints, their values could be swapped. So unless they are somehow forced to be the same, there are multiple solutions or there is some constraint we haven't been told about. $\endgroup$
    – Jaap Scherphuis
    Commented Feb 15, 2021 at 10:05

1 Answer 1

Reset to default
1
$\begingroup$

Here is the official solution from my puzzle book. Sorry for the confusion. Because there is only one solution printed I thought the solution is unique. Now I saw that this was missleading.

And I can't see any further restrictions in the solution.

@all Thanks for your help :)

enter image description here

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Unfortunately this solution isn't unique though, as the lower pair of 9 and 8 in the second column can be switched around and the solution still stands. Coupled with the earlier remarks in comments on the question itself, this feels like a puzzle that the makers didn't fully think through... $\endgroup$
    – Stiv
    Commented Feb 15, 2021 at 10:46
  • 1
    $\begingroup$ Yes you are right. I wanted to solve it without fully seeing the proposed solution so I only had a short look because I wanted to know if there is more than one solution printed. I realized that even 9 and 8 can be switched when I checked my solution with the "official one". (and of course mine was totally different.... ) It's a pitty that the makers didn't make further assumptions leading really to a unique solution. $\endgroup$
    – jane doe
    Commented Feb 15, 2021 at 10:52
  • 3
    $\begingroup$ @Stiv Not only that - in any 2×2 area, you can increase two of the (diagonally opposite) numbers and decrease the other two. $\endgroup$
    – Deusovi ♦
    Commented Feb 15, 2021 at 16:14
  • 1
    $\begingroup$ @Deusovi Very true - I guess this explains why Kakuros have the requirement for uniqueness, no unchecked lines (like the 9-9 in row 5 here) and often a very 'higgledy piggledy' shape to their grid! $\endgroup$
    – Stiv
    Commented Feb 15, 2021 at 16:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.