6
$\begingroup$

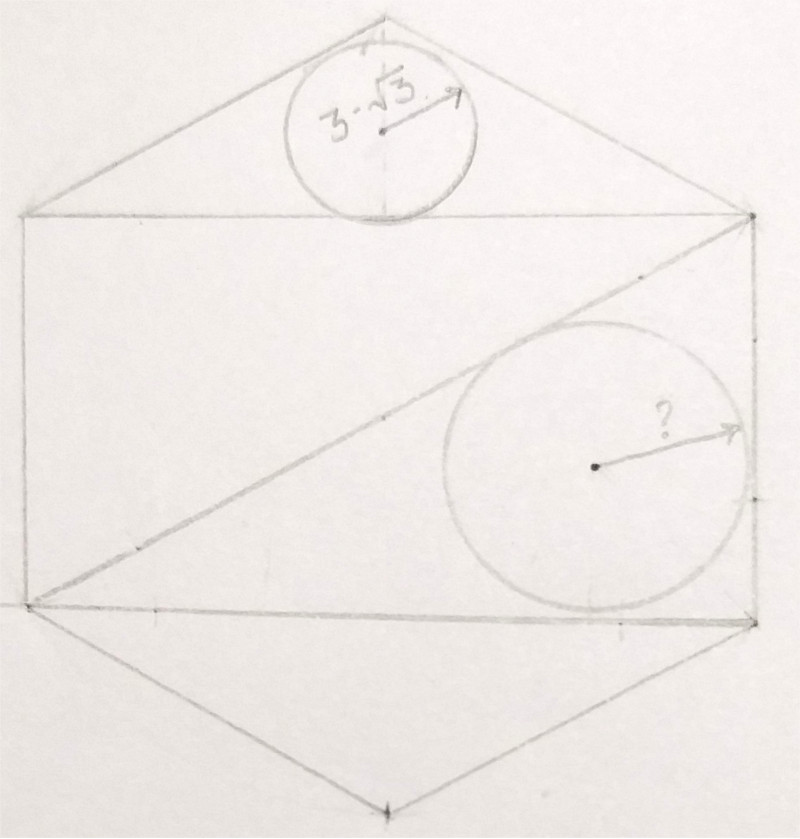
Here's a small mathematical puzzle I came up with recently:

Find the radius of the larger incircle, given that the radius of the smaller incircle is $3-\sqrt{3}$. The hexagon is a regular hexagon, with all sides equal enter image description here

P.S: the drawing was hand drawn and the incircles don't exactly touch all sides of the triangle. Sorry for that :P

Improve this question
$\endgroup$
0

1 Answer 1

Reset to default
8
$\begingroup$

The answer is

2

Easiest derivation:

obviously the ratio of areas of the two triangles is $2:1$; the areas are also proportional to incircle radius times triangle circumference. The circumferences are $2+\sqrt 3$ and $3+\sqrt 3$ in units of the hexagon side so $R = 2 (2+\sqrt 3) (3-\sqrt 3)^2 : [(3-\sqrt 3)(3+ \sqrt 3)] = 2$

Improve this answer
$\endgroup$
2
  • $\begingroup$ clever approach, but how did you get the circumferences? $\endgroup$
    – ThomasL
    Commented Aug 6, 2020 at 20:35
  • $\begingroup$ @ThomasL The sides of the triangles in question can all be related to either the height or the side of a regular triangle; the easiest way to make that totally obvious is splitting the regular hexagon into six regular triangles. $\endgroup$
    – Paul Panzer
    Commented Aug 6, 2020 at 20:43

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.