2
$\begingroup$

All diagonals of a convex pentagon are drawn, dividing it in one smaller pentagon and 10 triangles. Find the maximum number of triangles with the same area that may exist in the division.

The best I could do (or anyone can do) is that to draw a regular pentagon, which will give 2 sets of triangles, each having equal area, which means the maximum number for now is 5.

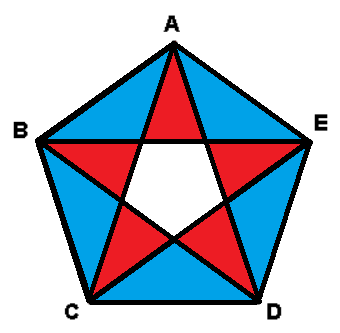
enter image description here

Can it be done better? Also can it be proved that the answer obtained (by someone) will be maximum?

Improve this question
$\endgroup$

3 Answers 3

Reset to default
5
$\begingroup$

We can do

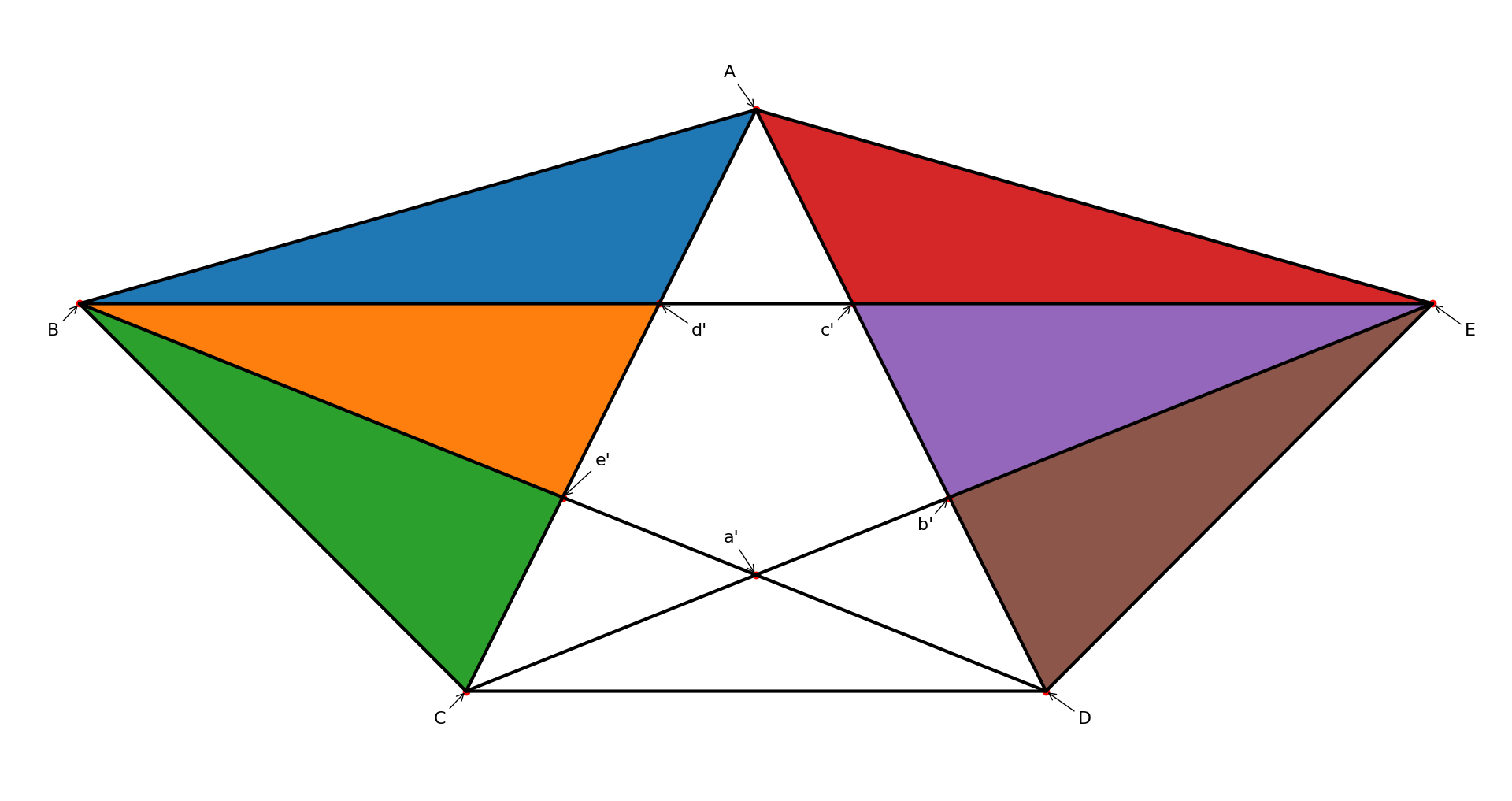
six: ![enter image description here
start with isosceles ACD cut AC and AD at third length yielding b,d and c,e. Find B by intersecting cd with eD and E by intersecting cd with Cb.

Things we can't do:

Making consecutive red-blue-red (OP colors) triangles equal: For example, X=Adc,Y=AcE,Z=Ecb. Because X and Y have the same height and area their bases must be equal, i.e. dc = cE. Similarly cb = cA. But that means that dA and bE are parallel, contradiction.

This still leaves open the possibility of

seven triangles, though.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Hmm. If you started with a different isosceles triangle, that would effectively perform an affine transformation on your entire figure, stretching or shrinking it horizontally. This would keep the ratios of the areas of all the triangles the same. So you can't stretch this to get an additional triangle of equal area. $\endgroup$
    – user3294068
    Commented Jan 11, 2021 at 20:27
  • 1
    $\begingroup$ @user3294068 if I understand correctly that is more or less the line of argument AxiomaticSystem have worked out in their answer. $\endgroup$
    – Paul Panzer
    Commented Jan 11, 2021 at 20:31
  • $\begingroup$ It's the motivating idea for the entire comment, actually, but a good line to pick up. $\endgroup$
    – AxiomaticSystem
    Commented Jan 13, 2021 at 14:00
3
$\begingroup$

Continuing Paul's result:

given the constraint on blue and red triangles, there are two possible configurations for 7 triangles: Paul's six plus aCD, and the six with the colored "inner triangles" switched with the white ones.

Let's check them, using the fact that since only areas are considered we can do arbitrary linear maps to fix points:

The former: Let $A,C,D$ be $(0,0),(3,0),(0,3)$ so that $e,d,c,b$ are $(2,0),(1,0),(0,1),(0,2)$ by the area requirement. Solving the diagonals yields $B,E,a = (4,-3),(-3,4),(\frac{6}{5},\frac{6}{5})$, from which we obtain that the six have area $\frac{3}{2}$ and $aCD$ has area $\frac{9}{10}$.

The latter: Let $A,B,E$ be $(0,0),(3,0),(0,3)$ so that $d,c$ are $(2,1),(1,2)$. We need $aB$ to be bisected by $AC$ so that $BCe=aCe$ - assuming symmetry about $x=y$ this happens when $D,C = (3,6),(6,3)$ which sets $b,e,a$ = $(\frac{3}{2},3),(3,\frac{3}{2}),(3,3)$. Unfortunately, while $bDE=abD=aCe=BCe=\frac{9}{4}$, $ABd=Acd=AcE=\frac{3}{2}$ and we can't get 7 this way either.

Therefore,

six triangles is best possible.

Improve this answer
$\endgroup$
2
$\begingroup$

This may be a bit too simple. and not the intended solution, but

the regular pentagon image in the question contains 10 triangles of equal area. Each of these triangles consists of one blue and one adjacent red triangle.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ Um I think you misunderstood. I am not talking about triangles formed by combining other triangles, I am talking about the single triangles formed itself. $\endgroup$
    – Anonymous
    Commented Jan 10, 2021 at 10:32
  • 1
    $\begingroup$ @Anonymous You should clear that up in your question because, for me, Jaap's answer is perfectly acceptable. $\endgroup$
    – hexomino
    Commented Jan 10, 2021 at 11:42
  • $\begingroup$ @hexomino Seconded. $\endgroup$
    – Paul Panzer
    Commented Jan 10, 2021 at 12:01

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.