5
$\begingroup$

Next level: Nurikolor (Level 2)

Welcome to my new puzzle: the NURIKOLOR, which is essentially the colored version of Nurikabe, but not exactly, with a few twists. The rules are simple:

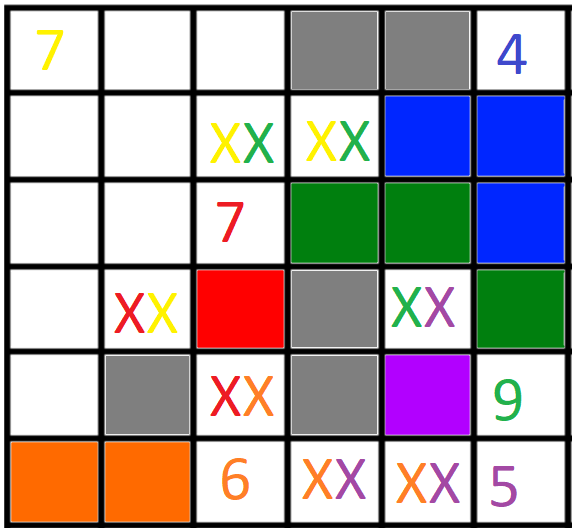
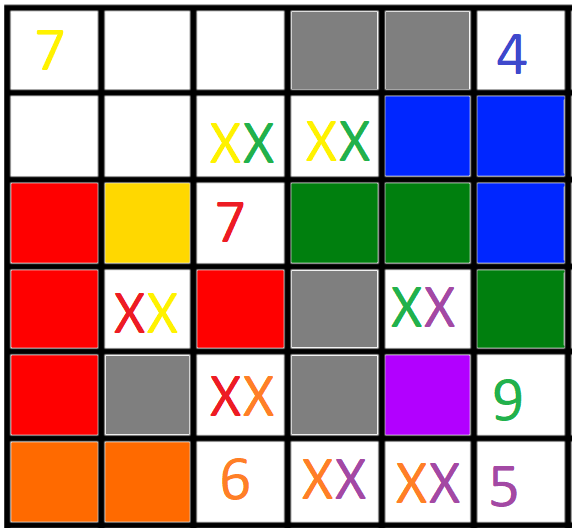
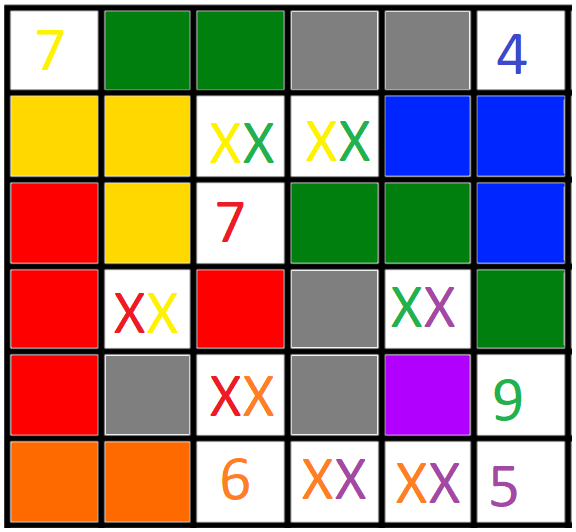
  • There are colored numbers on the grid, which indicate the number of tiles the group of its color holds.
  • There are tiles with 1 "X", which indicate the color of the tile.
  • There are tiles with 2 "X"s, which indicate intersections of colors. All intersections are shown, and these are the only intersections.
  • Grey tiles are not part of any group; they just serve as barriers.
  • The goal is to have every non-grey tile covered by a type of color.
  • 2 by 2 non-grey squares of the same color are illegal.
  • In future levels, there will be multiple numbers of the same color. They must not intersect.

Sorry, example deleted due to technical errors. Guess you have to try to read the rules instead... Sorry!

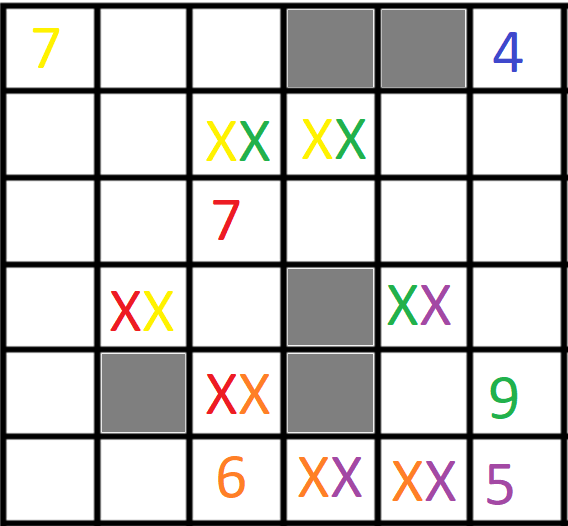
Now, can you solve this puzzle? (Level 1) Puzzle 1

Colorblind version:

y7 -- -- xx xx b4
-- -- yg yg -- --
-- -- r7 -- -- --
-- ry -- xx gp --
-- xx ro xx -- g9
-- -- o6 op op p5

r = red, y = yellow, g = green, b = blue, p = purple, o = orange, xx = gray
Improve this question
$\endgroup$
6
  • $\begingroup$ Note: If this is easy, things will get progressively harder. $\endgroup$
    – Player1456
    Commented Oct 11, 2020 at 6:13
  • $\begingroup$ I assume the coloured numbers also indicate the colours of their tile? $\endgroup$
    – boboquack
    Commented Oct 11, 2020 at 6:52
  • 1
    $\begingroup$ Is there an error in this puzzle? If all intersections are marked then we have 39 total numbers but only 38 spaces including intersections. (It works if the yellow 8 is actually a 7...) $\endgroup$
    – Stiv
    Commented Oct 11, 2020 at 6:55
  • $\begingroup$ Yes, I have an error... the "8" is actually a "7". My bad, will change. $\endgroup$
    – Player1456
    Commented Oct 11, 2020 at 7:14
  • $\begingroup$ @boboquack: Yes, that is true. $\endgroup$
    – Player1456
    Commented Oct 11, 2020 at 7:16

1 Answer 1

Reset to default
3
$\begingroup$

Some initial deductions:

Nurikolor

At this point, the cell in R5C1 cannot be yellow as there would be too many yellow cells otherwise. So that cell must be red. This allows us to fill in some cells making sure to leave connectivity for both the red and yellow islands.

Nurikolor_2

Finally, we can finish up making sure that there are no 2 x 2 yellow islands cells and green island cells are connected.

Nurikolor_3

Improve this answer
$\endgroup$
1
  • $\begingroup$ This was meant to be easy, congrats! Things will get harder as we go on. $\endgroup$
    – Player1456
    Commented Oct 11, 2020 at 7:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.