Lots of pieces are tangled here.
Can you find mate in one?
Small hint to guide you in the right way:
If you look at this position as a normal chess puzzle, the answer might be easy. However, notice the retrograde-analysis tag. To fully solve the mystery in this position you will need to dig deeper!
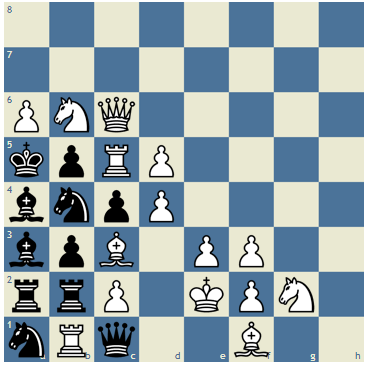
Since the puzzle turned out to be way hairier than expected, my earlier answer got quite messy and hard to follow, so here's a complete rewrite. It's still very clunky, but I hope it's much clearer than the earlier one. Also, there's an executive summary at the end, if you are not interested in all the gory details.
First, we'll need to make some general observations. If they seem to be overly complex, try to hang on: every single one of these will turn out to be important. (Yes, really!)
Observation 1: White has 15 pieces on board, and black has a doubled pawn on the b-file, and a pawn on the c-file.
Consequence 1: The only white piece that was captured was taken by black's a-pawn. No other white pieces were taken.
Consequence 1.1: The five missing black pawns were either taken or promoted on the file they started on.
Observation 2: Assuming minimal captures, white's pawns are all identifiable, up to the pawns on d-file being interchangeable. The only missing white pawn is the h-pawn.
Consequence 2: The piece taken by black's a-pawn was white's promoted h-pawn. (Or another white piece, and the h-pawn was promoted to replace it. Since it doesn't matter in which order the capturing and the promoting happened, I'll just keep talking about "capturing promoted pawns" when I mean both of these possibilities.)
Observation 3: White's b-pawn has ended up on the d-file, and black's c-pawn is still on the board. Also, all black's non-pawn pieces are still on the board.
Consequence 3: White's b-pawn has taken a promoted black pawn on the c-file, and the black d-pawn on the d-file. (Either that, or two promoted black pawns. The former case is the relevant one though.)
Observation 4: White's g-pawn has taken black's f-pawn on f3.
Summary so far: Out of the 5 missing black pawns, we have accounted for 2 captured as pawns (on d and f files) and one captured promoted on the c-file.
Observation 5: In order to promote the h-pawn, white must have cleared the path somehow. There are three options:
- white took black's h-pawn
- white's h-pawn took black's g-pawn
- white's h-pawn took another black piece on the g-file, and a pawn was promoted to replace it.
Consequence 5: no matter which of the three cases occurred, black lost one promoted/promotable pawn.
Observation 6: black's e-pawn was always blocked from promoting. The above cases have accounted for all the other black pieces, so it was impossible for white's d- and e-pawns to have swapped places by capturing, which could have opened the way for the e-pawn to promote.
Consequence 6: Either one or two black pawns were promoted. If two were promoted, one of them was captured on the g-file. In either case, one promoted pawn was captured by white's b-pawn on the c-file. In other words, all promoted black pawns have been captured by white pawns.
At first glance, it looks like
1. Nxc4#
could be the solution. Sure, why not. Let's check that the position could have been reached by legal play, though!
The black pieces are pretty much clumped up, so we can list all black's possible last moves:
If black's last move was Nb4, then it follows that white's previous move must have been Bxc3+. If not, then the white bishop would have been giving check on white's turn, which is of course illegal. But this poses a problem: there cannot have been anything on c3 for the white bishop to capture, because above we have accounted for all promoted black pieces (see "consequence 6"), and the only pawn that could have reached that square is still on c4.
From all this, is follows that
It cannot be white's turn!
Given the information from above, we can try to figure out white's last move. It must be something that enables a new previous move for black, since if it doesn't, we know from above that black has no moves.
Let's explore the four possibilities for this case:
Possibility 1: White's last move was Nb6. This enables a pawn move for black's previous move, but then the white move before that is impossible; there's no way the white rook can be giving check:
Possibility 2: White moved something that enabled the black queen to move last
Possibility 3: White took black's e-pawn, which was black's previous piece to move
Possibility 4: White moved something that enabled the black knight to move last
This looks promising, but there's still the problem of finding a suitable move.
This enables a move for the black knight, because it opens up a new previous move for white: now the bishop can have come from somewhere that's not on the black king's diagonal.
In other words, three half-moves ago, the board must have looked like this:
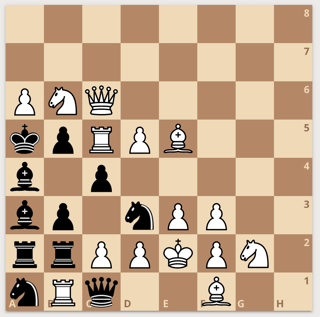
(white's dark square bishop could also be elsewhere on the long diagonal)
From there, the only way to reach the current position is
1. Bc3+ - Nb4 2. d4
Which finally allows for the long sought after mate in one:
2. - cxd3 e.p.#!
Phew! What a ride!
At the first glance, it looks like
1. Nxc4#might be the answer. However, a very complicated analysis proves that it cannot be white's turn to play: there are no legal games that can reach this position with white to play.
Starting again with the assumption that it's blacks turn, another round of analysis proves that in all possible legal games leading to this position with black to play, white's latest move can only have been "pawn from d2 to d4".
With white's previous move categorically established to have beend2 to d4, black's c-pawn is now allowed to capture en passant (cxd3 e.p.#), which checkmates the white king.
I may have missed some specific variations, so please drop a comment if you notice one. And thanks again, OP, for another brilliant puzzle!
To add on to Glorfindel's answer,
...cxd3 is also mate, assuming white just played d2 to d4. The question doesn't state if we're finding mate for white or black.
Nxc4
is mate because
pawn b5 is pinned
cd e.p.
Explanation
1.
It is black's turn to move. The only black piece for which there are free fields it could have moved from in the previous half move is the Queen. However, on all candidate origins the queen would have been checking the white King. In such a position, white must move (its King out of check) or loses by checkmate.
2.
No mate opportunities for black under null move heuristics. Assuming that black had moved in the last half move, the legal moves that would put white into check are: - capture c2 with either Queen or Rook. This can be countered by Bd2. - Move the Queen to d2 or d-f1. The white Rook or King would capture in turn
3.
Conclusion: the preceding half move of white must have created a move opportunity not available under null move heuristics. That can only be a pawn move allowing for capture an passant. The only white pawn on rank 4 is on file d.
