Can you find the Mate in 1?

Question

Once again, here is a new retrograde chess puzzle! This one might land on the trickier side of things, but don't let that deter you from giving it a shot. Your task here, at least, is straightforward enough:

Can you find mate in 1?

(12+14) FEN: b3b3/pRpR1pp1/B1K2pp1/qr5P/Qbkp4/P1P5/rPP2PP1/8

---

Edit: A number of people have voiced some confusion about this retrograde-analysis puzzle. So, in order to help you find the mate in 1, I decided that it's only fair for me to tell you that the last move of the game so far was [REDACTED].

As always, please provide your reasoning in your answer. Have fun solving this! :)

Answer 1

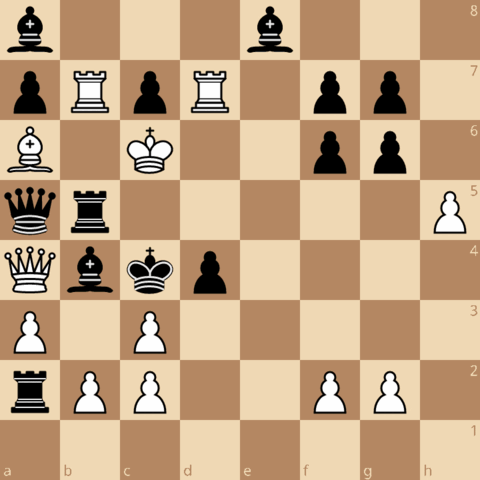
Thank you, for the most fun (and hardest) retrograde chess puzzle I've ever solved! A fantastic puzzle!

The answer is that

Black moved last, Ra2, and that white mates in 1 with Qb3#.

To answer the question of who moved last, and thus who is allowed to mate in 1, we must go on an adventure. First, let's answer

Which king got to its present location more recently?
If the white king got to its present location more recently, which square did it enter from? Not c5, d5, or e5, because the black king was already in the way. Not b6, d6, or c7 because the black pawn on c7 prevents those. And not b7 or d7, because the black bishops on a8 and e8 have been there since before the white king got into place. Thus, the black king got to its present location more recently.

With that in mind, we now ask

Where did the black king enter its present location from? Not b5, c5, or d5, due to the white king. Not b3, c3, or d3, due to the white pawns on b2 and c2. Thus, it must have entered from either b4 or d4. In either case, it must have been in place since the time when the c3 pawn made its capture.

Next let us figure out

What happened to white's missing pieces. White has 4 missing pieces (2 knights, the dark squared bishop, and the e pawn). Black's pawns have made 4 captures: exf7, hxg7, and the promoted light-squared bishop, which was either the original b7 pawn promoting on b1 after 2 captures, or the original b7 or d7 pawn promoting on d1 after 2 collective captures between that pair of pawns. Thus, black's pawns captured all of the missing white pieces.

Let's look more carefully at

The timing of black's promotion. Black promoted to a light-squared bishop before the kings reached their present locations, and in particular before the dxc3 capture. As a result, black could not have promoted on d1, and must have promoted on b1. As a result, we know the locations of all of black's captures: f7, g7, a2, b1.

In particular, something interesting happened to

White's dark squared bishop. The only capture location that is a dark square is f7, so white's dark squared bishop must have been captured there. This exf7 capture must have happened after the dxc3 capture, and after the kings got to their present locations.

Let's therefore examine how various pieces

Arrived to their current locations, relative to the kings reaching their places. First, how did black's dark-squared bishop get to b4. This must have happened after the exf7 capture, and after the kings were in their present location. In contrast, white's a6 bishop must've been in place since before the kings reached their current location, and some piece must've been on b5 since the time when the black king reached its spot - either the black rook or a white piece that moved away. Similarly, b7 and d7 were occupied since before the white king to its location, either by the white rooks, or by black pieces.

Now, we get to the

Queens! If they reached their current locations after the kings got to their current locations, the white queen must've come through e3 -> b6 -> a5, and the black queen must've come through a2 -> b3 -> a4. Whichever entered second would've been blocked. Thus, the queens were in place before the kings got there. In particular, a white knight must've been on b4, moved away revealing check, and had that check blocked by the black bishop, and then the white knight must've gone and been captured. This happened after the black promotion and after the exf7 capture, so that knight must've been captured in the hxg7 capture.

There's another issue:

How did the white pawn from e2 get captured, or promote to replace a captured piece? No captures happened on the e file, and the e file has never been open for promotion - the e8 bishop has been in place since before the exf7 capture. So the other black knight was captured by the e pawn, besides the one that was captured dxc3.

This puts some severe constraints on the recent past of the diagram position.

No pieces have been captured in the recent past. The most recent black capture was hxNg7, and the most recent white capture was either the dxc3 capture or the capture by the e pawn which promoted. In particular, if the last capture was hxNg7, there's not enough white waiting moves for the black rook to get all the way from h8, where it was stuck all game, behind the f8 bishop originally and the e8 bishop more recently. It would take 5 moves for the black rook to get from h8 to a2, which is one move too many, as white has only 3 waiting moves with the h pawn.

The only alternative is that

The most recent capture was by the white e pawn which promoted. This capture must've been on d8 or f8, and would've freed up a black knight both for tempo and to swap in the pinning/discovery squares (d7 and b7). The natural choice is exd8=R, where the white rook can move directly to d7 after a discovery by the black rook d7-e7, which can then get to a2 in only 3 further moves, e7-e1-a1-a2. To make this possible, the black knight must've come from the other pinning/discovery square, b7, and the other white rook must've come from b5, where it was replaced by the black rook from h8 after white's last capture.

This complicated set of maneuvers

Ends up using every single waiting move that white has available. In particular, black cannot move the knight directly from b7 to d8, as this would give double check and mate. Instead, black must move the knight around the other way, b7-c5-e6-d8, which requires a waiting move from the white h pawn when the black knight is on e6. One sequence of moves through this critical section covering the final two captures can be played over at this lichess study that I created. The h pawn must play one waiting move while the black knight maneuvers around and 2 waiting moves while the black rook gets to a2. Thus, there is not a single extra move for white available, so black must play the final move, allowing white to checkmate in 1!

To confirm the solution

I provide this full proof game in chapter 2 of the same study. Here's the PGN for the game: 1. e4 b5 2. a3 b4 3. Ra2 b3 4. Ba6 bxa2 5. Qe2 axb1=B 6. Qc4 Ba2 7. Ne2 Bb3 8. Qa4 Nc6 9. Nc3 Rb8 10. Nd5 Rb5 11. Nb4 Bb7 12. Ke2 d5 13. Kf3 Ba8 14. Kg3 Qb8 15. Re1 Qb6 16. Re3 Qa5 17. Rc3 Nd8 18. Rc5 Nb7 19. Kf3 Kd8 20. Ke3 Rb6 21. Rb5 Bc4 22. Kf4 Be2 23. Ke3 Bg4 24. Kf4 Bd7 25. Ke3 Be8 26. Kf4 Kd7 27. Ke3 Ke6 28. Kf3 Rd6 29. Ke3 Rd7 30. Kd4 Nd8 31. Kc5 Nb7+ 32. Kc6 Nf6 33. Nd3 Nh5 34. Nb4 Ng3 35. Nd3 Ne2 36. Nb4 Nc3 37. Na2 Ke5 38. Nb4 Kd4 39. dxc3+ Kc4 40. Bg5 Rg8 41. Bf6 exf6 42. Nd3+ Bb4 43. Nf4 Rh8 44. Ng6 hxg6 45. e5 Rh5 46. e6 d4 47. Rb6+ Rb5 48. e7 Nc5+ 49. Rb7 Ne6 50. h3 Nd8+ 51. exd8=R Re7+ 52. Rd7 Re1 53. h4 Ra1 54. h5 Ra2 55. Qb3#

Answer 2

Some preliminary thoughts. Inventory:

White has 12 units, so Black has captured 4. Two of these captures were e7xf6 and h7xg6, leaving two for [bPb7], either to get to d4 or to get via a2 to promote on b1.

All the pieces in the diagram are locked in place except bRa2. This imposes pre-conditions on some retractions, as follows:

bBb4 can't go back to f8 until wQa4 has a way to give the check or the rank-4 pin can be broken. How can that pin be broken? bK can't retract while wPc3 is on c3.

Black can't retract exf6 until bBb4 is home on f8. White can't retract dxc3 until Black's capture of the c1 bishop is retracted and that bishop is home.

That led me to believe that two subgoals are

to retract the dark-squared bishops home so that wPd2 and bPe7 can go home.

Here's my try:

Retro -1 ... c5xNd4 -2 Nf5d4 R~ -3 Ne7f5 R~ -4 Nd5e7 b6xNc5 -5 Ne4c5 Bf8b4 -6 Nb4d5+ {discovered check} exBf6

There may be alternatives for

the wNs' and bR's moves. Now wBf6 can retract to c1, wPc3 to d2. A wN can shield bK from wRd7 to let bK escape. Seeing as bPd4 had come from b7, the promoting bP was bPd7, which captured nothing and promoted on d1.

This doesn't establish whose move it is, though, because wPh5 has a spare move. So this is just a partial solution.

Answer 3

Queen to b3 is checkmate in one

Answer 4

First, the obvious solution:

Use uniqueness of the solution. Black has unlimited waiting moves and triangulation by the a2 rook. Therefore, it is impossible to prove it has to be black to move. Assuming the problem is solvable with unique solution, it has to be white to move and the mate in one is Qb3.

Yeah, this doesn't prove the position is reachable in a game or what the previous moves were, but it does solve the problem as stated. "Proper" retrograde solution follows:

Obviously,

black's light squared bishops were in place before wK was, so the king couldn't have come from b7 or d7, while c7 pawn prevents b7, c7 and d7. Other squares are blocked by bK. Thus, the wK was on c6 before bK was on c4.

Now, how this happened?

Black took 4 white pieces, all by pawns, because it had to promote one of pawns to one of light squared bishops - current d pawn either came from b or original b pawn captured on a2 and b1. White is missing a pawn, dark squared bishop and two knights, so these all had to be captured by pawns (white pawn possibly promoted; which might have been taken or still on the board). One black knight was captured by dxc3, the other gives white a single "free" capture.

Other thing to note

Is timing of the dxc3 move. We cannot have everything in place, followed by dxc3 as one of the last moves. If b pawn promoted, all captures but exf6 are on light squares. If d pawn promoted, dxc3 has to happen before. This poses a problem - where did the black king come from, then? Evidently, it needed to come from d4, followed (or immediately preceded) by dxc3. ((it maybe came from b4 but that doesn't change the dxc3 bit, only adding more constrains)).

Of course,

wB was in place before the king was as it couldn't have arrived from the top. Either bR or bB could have arrived after white piece moved from those two squares. bB required wN to move, bR could have arrived in place of a white rook or a knight. But, if coming from the top, bQ couldn't have arrived after the a6 bishop and kings were there, so wQ couldn't have either. Coming from the bottom, wQ couldn't have arrived, blocking bQ too. Thus, queens were there with blockers before kings. To get Bb4 in place (we need exf6 first to free it) we can assume wN was there before. So, a while ago, white had Nb4, then slid the queen in place, followed by black's queen, then wB, then b5 got either bR or wN or wR, followed by king moving from d4 to c4, followed or immediately preceded by dxc3.

Then, after some moves,

exf6 happened. It needs to happen after dxc3 as dark squared bishop needs to be captured there. Because it happens after, white pawn had to take one black knight to promote (e7 pawn blocked its line and after this move e8 bishop does). So, we know what happened with both black knights - d and e pawns took one each, meaning no free knights for grabs by pieces. Also, this means d4 pawn started on d and the b pawn promoted.

So, what happens with the knight?

We have one knight that was previously on b4 which we need to get rid of by pawn capture. Where? It could only fall on g6 - bishops were in place before so the promotion of the b pawn (and required captures) had to happen already, as did the exf6 one. This in turn means the black rook can get out only now (it was blocked by f8 bishop before and e8 one now).

What happened with that rook?

The rook cannot reach a2 in the limited waiting moves by the h pawn. It can reach b5 though. Could a state several moves ago be bNb7, wRb5 and after the rook gets opened and moves to h5, black knight moves from b7 to d6, then (Rb7, Rb5). Nice, but the problem is where could the bN disappear to? Either c3 (had to happen before) or the one pawn that promoted. Either that promoted pawn is on the board or it had to be captured - but at this current point, there are no captures left.

This means

That the bN was taken by the promoting pawn, which is still on the board. I was stuck for a while thinking d7 blocker had to be a black knight that was captured ... but it wasn't a knight, it was the a2 rook - the only piece that can still move around. So, promotion of the white pawn on d8 (to rook, which is on d7 in the final solution) follows by (... Re7) then (Rd7, Re1), (h pawn, Ra1), (h pawn, Ra2). If h pawn moves were h3 and h4 you would get extra white pawn move allowing for being black to move (which can, as stated, triangulate with the a2 rook).

So, is the solution not unique?

Nope! The knight that was on b7 and taken by the promoting pawn had to come from e6 to be taken on d8 - doing jump to d8 directly is not possible due to double check. So, white moved e6-e7 for the black knight to jump to e6 in preparation for Nd8. Then, white had to wait for Nd8 - and this waiting move had to be done by the h pawn as the only mobile white piece. Meaning the previous spoiler had to have h4, h5 as the pawn moves. This means that the black's Ra2 has to be the only possible last move.

Hooah, finally solved it (in a way that feels good enough for me - unique determination of the only possible last player to move by retrograde analysis; but without proof game). But I see that isaacg solved the problem before me and even included proof game; congrats :)

Answer 5

I don't see a forced previous move, several options come to mind. I think I'd go with

the black Queen just took a Knight on a5, since with a white Knight there, the black King would have no moves, and both Rook and Bishop are pinned, so Qxa5 is forced