9
$\begingroup$

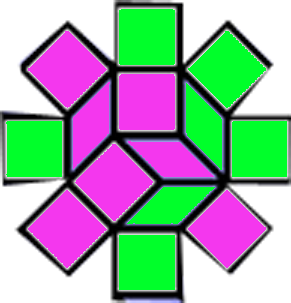
enter image description here

Arrange numbers 1 to 14 to the quadrilaterals, such that:

  1. The difference between 2 numbers in squares having a common edge or corner is greater than 2.
  2. Numbers inside the green quadrilaterals are even numbers.
  3. Numbers inside the purple quadrilaterals are odd numbers.
Improve this question
$\endgroup$
2
  • $\begingroup$ "The difference between 2 numbers sharing the same edge and corner are > 2." does that mean it applies to two squares that share a corner but no edge? $\endgroup$
    – sousben
    Commented Sep 29, 2017 at 7:25
  • $\begingroup$ @sousben : Yes. $\endgroup$
    – Jamal Senjaya
    Commented Sep 29, 2017 at 7:27

1 Answer 1

Reset to default
10
$\begingroup$

This is a possible answer. Enjoy

enter image description here

How I solved it?

Manually, starting from the purple quadrilateral with the most neighbors (13 in the solution). There is only one purple square that is not adjacent to it, therefore the value could only be an extremity, either 1 or 13. The rightmost purple square, being the only non adjacent to the one we filled, has to be a consecutive number. Either 3 or 11 respectively (11 in the solution).

If you apply the same logic

to the next quadrilateral with the most neighbors (1 in the solution), this one also has to be an extremity of the remaining set, either 1 or 9. Once you've filled these 3 quadrilaterals, the rest is pretty straightforward as there is almost always a quadrilateral that can only accept one value, and you either get stuck (I got stuck in all possible configurations with 1 in the starting quadrilateral), or you finish the puzzle.

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.