2
$\begingroup$

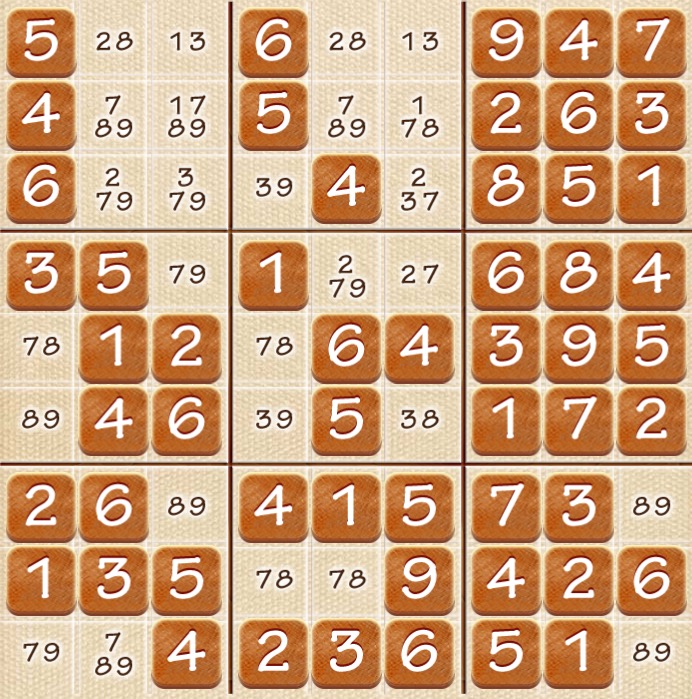
enter image description here

Don't worry, this is the last hint I'll ask for.

I've looked for any position that could exclude others, and what's left is perfectly symmetrical. I've even tried mentally choosing some options to see if I get any contradictions, but nothing obvious jumped at me.

Could you provide me with a hint (and the reasoning behind it) for a next move?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ I found a contradiction if the top left 3x3 grid's bottom right cell is a 9, based on the fact that it removes all possibilities for 9 in the center. $\endgroup$
    – greenturtle3141
    Commented Mar 8, 2017 at 19:57

2 Answers 2

Reset to default
5
$\begingroup$

This position requires an advanced technique called forcing chains.

This technique involves looking at cells which have two candidates and tracing out the implications of each alternative. If they both lead to the same result (for example, eliminating the same candidate from some cell), then you can rest assured that that result is correct.

It is helpful when tracing out the implications of each alternative to use different colored pens or pencils. When drawing out the implications, you may also want to reproduce the candidates below themselves in different colors, so that you don't confuse your exploration of possibilities with permanent inferences.

Here is an example of using this technique on your grid:

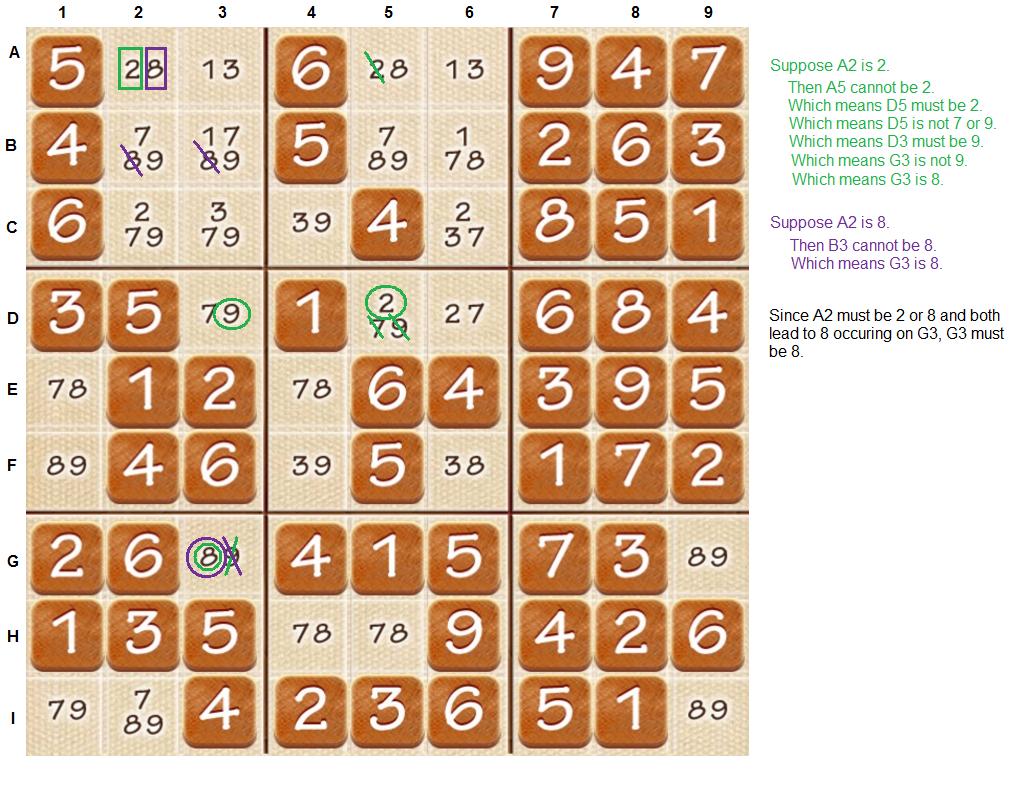
![enter image description here

This technique is based on a general pattern of reasoning which can be represented schematically as:

φ or ψ
φ → χ
ψ → χ
Thus, χ

Improve this answer
$\endgroup$
2
$\begingroup$

Two inferences may be made that greatly simplify the sudoku.

Firstly...

If you were to place a 7 in D6 the top-middle nonnet would not be completable, therefore it must be a 2:

               X
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 · | 1 · 7 | 6 8 4 | X
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

       X     Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 2 7 | 6 8 4 | XY
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

             X
             Y
             Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 8 · | 9 4 7 | X
B| 4 · · | 5 9 · | 2 6 3 | Y
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 2 7 | 6 8 4 |
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · 7 9 | 4 2 6 | Z
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

               X
           Y   Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 8 1 | 9 4 7 | X
B| 4 · · | 5 9 · | 2 6 3 |
C| 6 · · | 3 4 2 | 8 5 1 | YZ
 --------+-------+--------
D| 3 5 9 | 1 2 7 | 6 8 4 |
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · 7 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

               X
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 8 1 | 9 4 7 |
B| 4 · · | 5 9   | 2 6 3 | X : X is 7 but not 7 -> contradiction.
C| 6 · · | 3 4 2 | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 2 7 | 6 8 4 |
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · 7 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

Secondly...

If you were to place a 9 in D3 the top-middle nonnet would not be completable, therefore it must be a 7:

       X
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 | X
E| · 1 2 | · 6 4 | 3 9 5 |
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

   X   Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| · 1 2 | · 6 4 | 3 9 5 |
F| 8 4 6 | · 5 · | 1 7 2 | X
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 · | Y
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

   X           Y       Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | · 6 4 | 3 9 5 | X
F| 8 4 6 | · 5 3 | 1 7 2 | Y
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 | Z
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

           X
   W       Y           Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | 8 6 4 | 3 9 5 | X
F| 8 4 6 | 9 5 3 | 1 7 2 | Y
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 |
H| 1 3 5 | · · 9 | 4 2 6 |
J| 9 · 4 | 2 3 6 | 5 1 8 | WZ
 --------+-------+--------

     X     Y 
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | 8 6 4 | 3 9 5 |
F| 8 4 6 | 9 5 3 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 |
H| 1 3 5 | 7 · 9 | 4 2 6 | Y
J| 9 7 4 | 2 3 6 | 5 1 8 | X
 --------+-------+--------

           X Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | 3 4 · | 8 5 1 | X
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | 8 6 4 | 3 9 5 |
F| 8 4 6 | 9 5 3 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 |
H| 1 3 5 | 7 8 9 | 4 2 6 | Y
J| 9 7 4 | 2 3 6 | 5 1 8 |
 --------+-------+--------

       X     Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 2 · | 9 4 7 | Y
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · 7 | 3 4 · | 8 5 1 | X
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | 8 6 4 | 3 9 5 |
F| 8 4 6 | 9 5 3 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 |
H| 1 3 5 | 7 8 9 | 4 2 6 |
J| 9 7 4 | 2 3 6 | 5 1 8 |
 --------+-------+--------

               X
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 2 · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · 7 | 3 4   | 8 5 1 | X : X cannot be any value -> contradiction
 --------+-------+--------
D| 3 5 9 | 1 · · | 6 8 4 |
E| 7 1 2 | 8 6 4 | 3 9 5 |
F| 8 4 6 | 9 5 3 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 |
H| 1 3 5 | 7 8 9 | 4 2 6 |
J| 9 7 4 | 2 3 6 | 5 1 8 |
 --------+-------+--------

Setting these two values to what they logically must be, the rest of the sudoku is solvable entirely by "naked singles":

   X         Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 | Y
E| 8 1 2 | · 6 4 | 3 9 5 | X
F| · 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

   X       Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 | Y
F| 9 4 6 | · 5 · | 1 7 2 | X
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | · · 9 | 4 2 6 |
J| · · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

   X       Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | · 5 · | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 · 9 | 4 2 6 | Y
J| 7 · 4 | 2 3 6 | 5 1 · | X
 --------+-------+--------

           X Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 · · | 2 6 3 |
C| 6 · · | · 4 · | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 · | 1 7 2 | X
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 7 9 | 4 2 6 | Y
J| 7 · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

           X Y Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 · · | 9 4 7 |
B| 4 · · | 5 8 · | 2 6 3 | Y
C| 6 · · | 9 4 · | 8 5 1 | X
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 | Z
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

       X     Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 · · | 6 2 · | 9 4 7 | Y
B| 4 · · | 5 8 · | 2 6 3 |
C| 6 · 3 | 9 4 · | 8 5 1 | X
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

     X         Y
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 8 · | 6 2 · | 9 4 7 | X
B| 4 · · | 5 8 · | 2 6 3 |
C| 6 · 3 | 9 4 7 | 8 5 1 | Y
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 · 4 | 2 3 6 | 5 1 · |
 --------+-------+--------

     W
     X Y       Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 8 1 | 6 2 · | 9 4 7 | Y
B| 4 · · | 5 8 1 | 2 6 3 | Z
C| 6 2 3 | 9 4 7 | 8 5 1 | W
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 |
 --------+-------+--------
G| 2 6 · | 4 1 5 | 7 3 · |
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 9 4 | 2 3 6 | 5 1 · | X
 --------+-------+--------

       W
     V X       Y       Z
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 8 1 | 6 2 3 | 9 4 7 | Y
B| 4 7 9 | 5 8 1 | 2 6 3 | VW
C| 6 2 3 | 9 4 7 | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 · | X
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 9 4 | 2 3 6 | 5 1 8 | Z
 --------+-------+--------

                       X
   1 2 3   4 5 6   7 8 9
 --------+-------+--------
A| 5 8 1 | 6 2 3 | 9 4 7 |
B| 4 7 9 | 5 8 1 | 2 6 3 |
C| 6 2 3 | 9 4 7 | 8 5 1 |
 --------+-------+--------
D| 3 5 7 | 1 9 2 | 6 8 4 |
E| 8 1 2 | 7 6 4 | 3 9 5 |
F| 9 4 6 | 3 5 8 | 1 7 2 |
 --------+-------+--------
G| 2 6 8 | 4 1 5 | 7 3 9 | X : complete
H| 1 3 5 | 8 7 9 | 4 2 6 |
J| 7 9 4 | 2 3 6 | 5 1 8 |
 --------+-------+--------

Note:
You could perform either one of the inferences, simplify the board and then spot the other more easily.

Improve this answer
$\endgroup$
5
  • $\begingroup$ We all know the Sudoku move of last resort: Guess a value and proceed to solve the puzzle based on that guess.  If you hit a contradiction (impossible situation), you know your guess must have been wrong, so backtrack and restart, knowing that that value is wrong. ISTM that that’s all you’re doing here.  Do you have some elegant procedure for identifying these values that cause a different 3×3 square to become unsolvable, or is it just brute force? $\endgroup$
    – Peregrine Rook
    Commented Mar 11, 2017 at 23:13
  • $\begingroup$ Well if you want the strategy name it's called Alternating inference chains. But it is effectively guess and check, much like a lot of "advanced" strategies. Another way to do the same would be 3D Medusula - which is colouring the values which reveals the same contradictions (and many others on the same path at the same time). $\endgroup$
    – Jonathan Allan
    Commented Mar 11, 2017 at 23:17
  • $\begingroup$ The other answer is also a "suppose X is Y" ... "contradiction" too BTW - if you have a pattern recognition method feel free to add value by answering! $\endgroup$
    – Jonathan Allan
    Commented Mar 11, 2017 at 23:18
  • $\begingroup$ There is also an X-Cycle of length 6 but I do't think it sticks out as a pattern to recognise either. $\endgroup$
    – Jonathan Allan
    Commented Mar 11, 2017 at 23:23
  • $\begingroup$ @PeregrineRook also note that you don't have to start at the values I started with it works for most (maybe even all) of the incorrect values on this grid! $\endgroup$
    – Jonathan Allan
    Commented Mar 11, 2017 at 23:38

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.