4
$\begingroup$

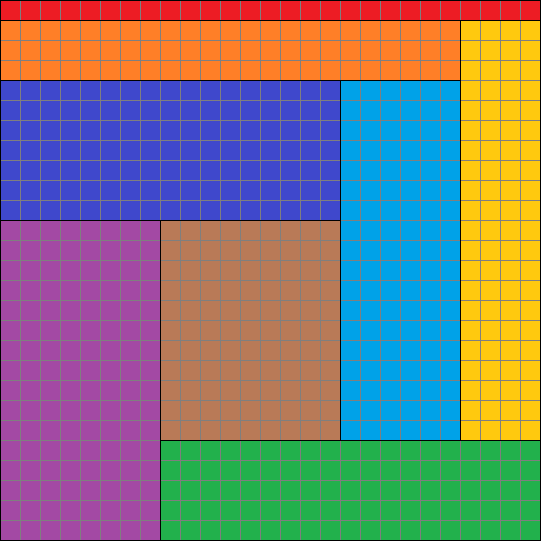
How can I cut a 27×27 square into 8 incomparable rectangles?

A rectangle with width w and height x is incomparable with a rectangle of width y and height z iff $w<y\land x>z$ or $w>y\land x<z$. (Assume $\text{width}\le\text{height}$.)

All rectangles must have integer side lengths.

The book Fractal Music, Hypercards and more... by Martin Gardner says

but eight rectangles can tile a square of side 27

Please don't look in the book; there are no answers there.

Improve this question
$\endgroup$
5
  • $\begingroup$ "(Assume width <= height)" Does this mean 4×7 and 8×5 rectangles are comparable? $\endgroup$
    – AxiomaticSystem
    Commented Aug 15 at 1:53
  • $\begingroup$ @AxiomaticSystem Yes, they are. $\endgroup$
    – Lucenaposition
    Commented Aug 15 at 1:54
  • 4
    $\begingroup$ The layman's definition for incomparable rectangles is just "neither will fit inside the other", which seems somewhat easier to grasp than the given notation. $\endgroup$
    – Bass
    Commented Aug 15 at 2:37
  • 1
    $\begingroup$ @Bass It might be possible to rotate one rectangle (by an angle that is not 0 or 90 degrees or something equivalent) and fit it inside the other. For example, a 1x6 can fit inside a 5x5 (with rotation). $\endgroup$
    – Lucenaposition
    Commented Aug 15 at 2:39
  • 1
    $\begingroup$ @Bass's statement implies parallel edges, which would be explicitly stated in a proper description. $\endgroup$
    – Daniel Mathias
    Commented Aug 15 at 2:42

1 Answer 1

Reset to default
9
$\begingroup$

The widths and heights of the rectangles are:

1:27 3:23 4:21 5:19 6:18 7:17 8:16 9:11

They tile the square:

solution image

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.