Find two numbers based on either their multiplication or exponent

Question

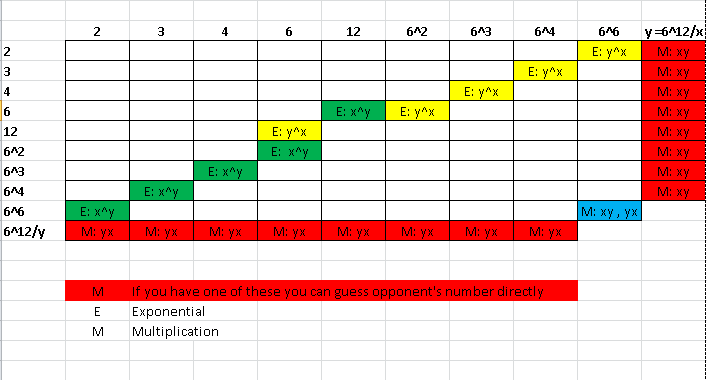
There are two mathematicians with incredibly strong memories sitting in a coffee shop passing the time. Their server proposes a game:

First, he gives them a large number:

$2176782336$

Then he whispers a number $x$ to the first mathematician, and another number $y$ to the second mathematician. Afterwards, he tells them that the first large number is either the product of the two numbers, or one number raised to the power of the other.

He promises to pay for their order if they can each demonstrate that they know the other's number without offering each other any additional information.

After along silence this conversation takes place .

• The first mathematician declares: I don't know your number.
• The second mathematician says: Now I know your number.
• The first mathematician states: Now I think I know both numbers.

Before anyone announces these numbers, the manager of the cafe promises to award a prize to the first person in the coffee shop who succeeds in discovering what they are. Can you find $x$ and $y$?

-Note:

• (x, y) differs from (y, x)

• Silence is significative , and everyone knows this

Answer 1

The first mathematician: F

The second mathematician: S

F has $x$ and S has $y$.

F thinks that S has a number in the form of $6^{12/x}$ or $6^{12}/x$.

S thinks that F has a number in the form of $6^{12/y}$ or $6^{12}/y$.

Let's say $G_1=\{2,3,4,6,12\}$ are the sets of number that can be used in $6^{12/z}$ and to get a whole number (I didn't include $1$ for obvious reasons) and let $G_2=\{6^2,6^3,6^4,6^6\}$ be the numbers that can not be used in $z^{G_1}$. We call $G$ as the union of $G_1$ and $G_2$.

Now see a table:

From OUR point of view:

If F had numbers in the red row he could easily guess S's number. He says I don't know, which means he has $6,6^2,6^3,6^4,6^6$.We are confused between green cells and red cells, for $6^6$ between green and blue cell.

S says at first he didn't know, which means he didn't have a number in red column, if not he could easily guessed F's number. Also he knows that F does not have a number in red row, if F had he would guess S's number. Since we eliminated red rows now we are not confused in yellow and red cells. The only possible situation that F and S has that conversation is that both of them has $6^6$.

Mission accomplished.

As the OP noted I took $(x,y)$ different than $(y,x)$, if not there would be multiple solutions. To see the solutions color yellow cells to green.

Answer 2

Proof of uniqueness: this analysis is from the first, non-modified version of the puzzle.

Let's denote $S=\{2,3,4,6,12,36,216,1296,46656\}$ as the set of valid solutions.

If someone would have been informed a number bigger than a number from this set $S$, he declares that he knows other number immediately before the conversation would take place.

---

the first said I don't know numbers means:

Since the second mathematician didn't declare anything revealing from his number, we know the first doesn't have $\{2,3,4,12,36,216,1296\}$. These numbers are to be multiplied by a bigger number than $6^6$ which is not the case.

Second, these numbers can be one of either the exponent, or the base, to attain the huge number result:

$2$ leads to either $2*1088391168$ or $46656^2$ , the first is excluded before the first statement. So the first would know henceforth.

the second statement: I just know numbers.

If the first person has $6$ the second would have had consequently either $12$ or $36$, if he has $12$, the result is written as $6^{12}$. In the other case it is $36^6$, both cases the second mathematician should be aware of first number.

The other choice is $46656$, as $2$ is excluded before the conversations starts. Supposing the second mathematician is aware of this, the result can't be written as $46656^2$. The unique case left is $46656*46656$.

Set of valid solutions is ${12,36,46656}$

the third statement: Me too I know them

This doesn't fit the number $6$ because it leads to two options of $y$ either $12$ or $36$. He said I know, so that leave us with the last unique choice: $(x,y)=(46656,46656)$

Answer 3

I think the answer is :

(6, 12) or (6, 36)

If x is NOT one of 6, 12 or 36, then the first mathematician would know the other one's number, so the numbers are definitely 6, 12 or 36.

If x was 6, then y could be either 12 or 36 (as 36 to the 6th power is also an answer). So first mathematician can't be sure. Therefore his number is 6.

The second mathematician's number is either 12 or 36, so he knows x must be 6. Then the first mathematician knows that the numbers are either (6, 12) or (6, 36).

Answer 4

NOTE: This actually fails in the same way as this puzzle (my answer).

---

Call the first mathematician $\alpha$ and the second mathematician $\beta$. $\alpha$'s number is $x$ and $\beta$'s number is $y$. I will assume that $x,y \in \mathbb{N}$

The set of numbers which (as factors are divisors) are raised to some power to equal $2176782336$ is $f = \{2,3,4,6,12,36,216,1296,46656\}$. This set is complete. Also note that $\forall z \in f. 2176782336 \mod z \equiv 0$ - i.e. all members of $f$ are also factors.

First Comment: The only way that first comment can occur is if $x \in f$. All members of $f$ present ambiguity regarding product and exponent.

Second Comment: If $\beta$ had the number $y \not\in f$ (but still at least in the factors of $2176782336$) then he would know the value of $x$. Similarly if $y=2$ then he would know that the only way this can occur is if $x=46656$. Similarly, for all $y \in f \land y\not=46656$ he would also know. The only case which still presents ambiguity for $\beta$ is $y=46656$ ($\alpha$ could still have either $x=2 \lor x=46656$).

Third Comment: We ALL know that $y=46656$. So $\alpha$ declares this.

Fourth Comment: Unfortunately, $\beta$ knowing that $\alpha$ knows $y$ does not help him. The first three comments could have occurred with either $x=2 \land y=46656$ or $x=46656 \land y=46656$. The fourth comment cannot occur using the initial assumption, and I suggest would fail from infinite solutions if instead $x,y \in \mathbb{R}$, say. Thus, the puzzle is inconsistent. The fourth comment should have been "I still don't know your number".