This is a beautiful variant of "The Hardest Logic Puzzle Ever". I learned it in high school math class a while ago. (I do not know if my teacher invented this variant.) I know the solution but I want to share with you.
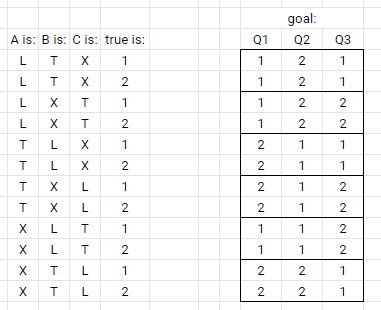
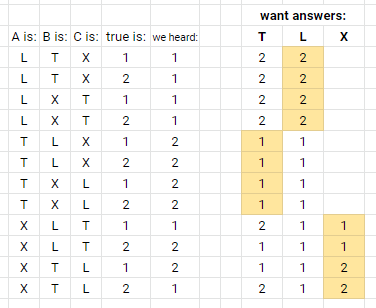
Three gods A, B, and C are called, in no particular order, True, False, and Xor. True always speaks truly, False always speaks falsely, while Xor speaks by xor in his head the answer of True and False if the question asked of him had been asked of the other two. For example, if we ask Xor: "Are you True?" then if the question had been asked of True, he would have answered "Yes", the same for False, hence Xor answer "No". In other words, if the answers of true and false were in agreement Xor answer "No", otherwise Xor answers "Yes". Your task is to determine the identities of A, B, and C by asking three yes-no questions; each question must be put to exactly one god. The gods understand English; but will answer all questions in their language, in which the words for yes and no you don't know a priori. However, you know that their language is based on the English alphabet, so they are distinguishable words.
No "paradoxical" question is allowed.