First of all, I'm not sure this is exactly the correct answer based on two issues, which I will highlight as they appear.
The first observation is...
that a domino is divided into two parts by exactly one line.
This means
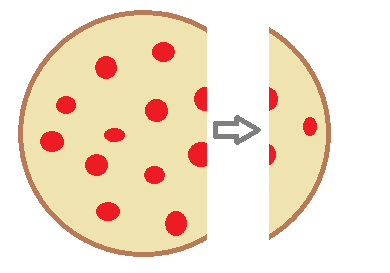
The pizza is not sliced into standard "triangular" sectors, but rather it is sliced by a chord.
As shown here:
Now we can find
the angle of each section using the formula $\theta = \frac{s}{r}$ where $s$ is the arclength and $r$ is the radius.
First couple: $\theta_1 = \frac{54.97\ \rm{cm}}{18\ \rm{cm}} = 3.0539\ \rm{rad}$
Second couple: $\theta_2 = \frac{16.49\ \rm{cm}}{18\ \rm{cm}} = 0.9161\ \rm{rad}$
Third couple: $\theta_3 = \frac{5.49\ \rm{cm}}{18\ \rm{cm}} = 0.3050\ \rm{rad}$
Now we calculate
the area of these slices, using the formula $A = \frac{r^2}{2}(\theta-\sin\theta)$.
First couple: $A_1 = \frac{(18\ \rm{cm})^2}{2}(3.0539-\sin3.0539) = 486.09\ \rm{cm}^2$
Second couple: $A_2 = \frac{(18\ \rm{cm})^2}{2}(0.9161-\sin0.9161) = 145.82\ \rm{cm}^2$
Third couple: $A_3 = \frac{(18\ \rm{cm})^2}{2}(0.3050-\sin0.3050) = 48.55\ \rm{cm}^2$
Note that while $\theta$ is found in radians, the $\sin$ function is evaluated as though its argument is in degrees. This is the first major issue; I only initially considered this by a mistake in my calculator settings. Regardless, I think this path notable because scaling our pizzas to the unit circle, we get
First couple: $A_1 = \frac{1}{2}(3.0539-\sin3.0539) = 1.5003$
Second couple: $A_2 = \frac{1}{2}(0.9161-\sin0.9161) = 0.45006$
Third couple: $A_3 = \frac{1}{2}(0.3050-\sin0.3050) = 0.14983$
Which are all extremely close to a ratio of small integers. Unfortunately, I had no luck trying to construct them using numbers given the couples' constraints (eg. consecutive odd).
However,
Looking back at the sector area in $\rm{cm}^2$, we find a rather simple means of constructing the area from two numbers:
Shift the smaller of the numbers one place to the left, then add.
This works for each of the given couples:
First couple: $\{44,46\}\Rightarrow 440+46 = 486\approx486.09\ \rm{cm}^2$
Second couple: $\{13,15\}\Rightarrow 130+15 = 145\approx145.82\ \rm{cm}^2$
Third couple: $\{4,9\}\Rightarrow 40+9 = 49\approx48.55\ \rm{cm}^2$
Note the second major issue--the first couple uses numbers which are outside the given allowable range of $[1,25]$.
With a function for determining the arclength identified, we can now find the arclength for the first two primes.
$\{2,3\}\Rightarrow 20+3 = 23\ \rm{cm}^2$
Solving $23\ \rm{cm}^2=\frac{(18\ \rm{cm})^2}{2}(\theta-\sin\theta)$:
$\theta = 0.1445\ \rm{rad}$
$s = r\theta = (18\ \rm{cm})0.1445 = 2.60\ \rm{cm}$
Therefore, the first two prime numbers share a slice with an arclength of
2.60 centimeters