12
$\begingroup$

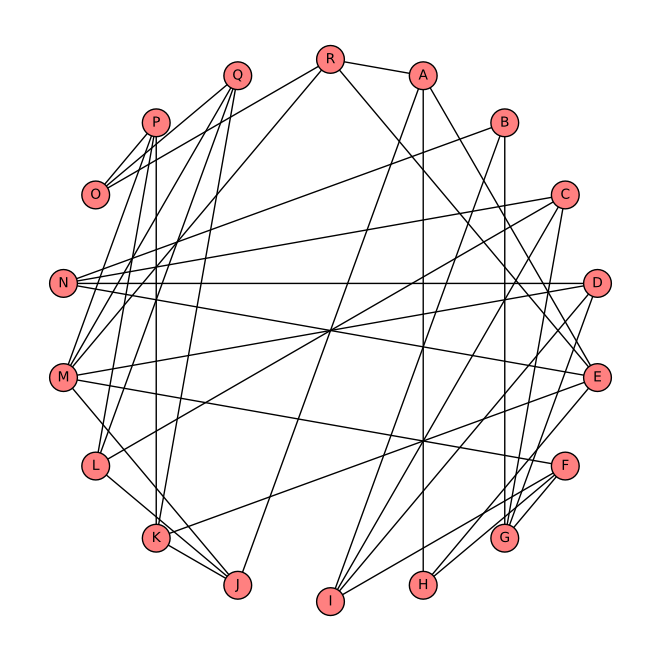
I have 18 students in my graph theory course this semester: Anne, Bernard, Clare, David,..., and Rachel. At the start of the course I asked them to draw the graph below, in which each of them is represented by a vertex, two of which are joined by a line if, and only if, they represent two students who are friends.

Fewer than half my students turned up for class last Friday. However, because each of the absent students happened to be friends with at least one of those who did attend, I was able to return everyone's assignments either personally or through a friend. In fact, had a smaller group of students shown up for my class on Friday, I would have been unable to do this.

How may students attended my class on Friday, and who were they?enter image description here

Improve this question
$\endgroup$

2 Answers 2

Reset to default
11
$\begingroup$

This is an excellent question to demonstrate why the greedy algorithm doesn't always work
The minimum number is actually

$4$

Here are the students which would satisfy the criteria (I think this group is unique)

$F, J, N, O$ (Frank, Jack, Norman, Orville)

Proof that this is minimal

As El-Guest pointed out the maximum number of friendships in the group are held by $M$ & $E$ with $6$ & $5$, respectively. Every other person has four friends or fewer. Therefore, the maximum possible number of people covered by three students is $7+6+5 = 18$. This could only possibly be achieved if $M$ and $E$ were in the group of three but, as El-Guest explored, you need to add three more students to cover everyone else.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I used a solver, getting the same solution and only this one ; hence i am pretty sure it is unique. $\endgroup$
    – aluriak
    Commented Mar 15, 2019 at 18:26
  • 1
    $\begingroup$ For those interested in the mathematical details, this is called the Set Cover problem. It is a well known problem in computer science and is NP-Hard.The best known linear programming approaches take exponential time. $\endgroup$
    – darksky
    Commented Mar 16, 2019 at 17:49
4
$\begingroup$

I'd assume that you'd want

to have the students attend which have the maximum number of friendships...

So:

M & E have 6 & 5 friendships respectively, then P,Q,R,D,F,J don't have to go thanks to M; and A,H,K,N,R don't have to go thanks to E. This leaves us with B,C,G,I,L,O who we need to deal with. O is only connected to P,Q,R, so they have to attend; C is connected with G,I,L; and then B is the last one left who has to show up.

The minimum number of students is therefore

5, and they are Megan, Ethan, Billy, Chris, and Orville.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I figured as much, I didn't like how two of the students had to double up. $\endgroup$
    – El-Guest
    Commented Mar 15, 2019 at 15:00
  • 3
    $\begingroup$ How do you know this is minimal? $\endgroup$
    – noedne
    Commented Mar 15, 2019 at 15:23
  • 1
    $\begingroup$ I didn’t, as clearly demonstrated by hexomino’s answer. $\endgroup$
    – El-Guest
    Commented Mar 15, 2019 at 15:52

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.