Arrange numbers to 3x3 square with concentric (Addition and Difference)

Question

2 Similar questions:

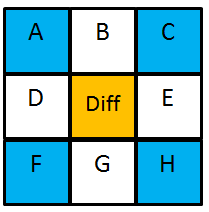
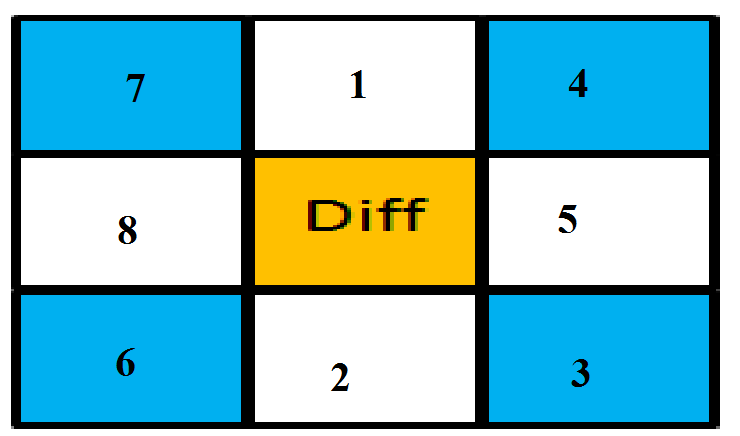
I. Difference

Arrange numbers 1 to 8 to replace the letters so:
A = abs (B – D)
C = abs (B – E)
F = abs (D – G)
H = abs (E – G)
To eliminate duplicates by rotation and reflection, let’s set the rules: A > C, A > F, A > H and F > C
There are 2 solutions

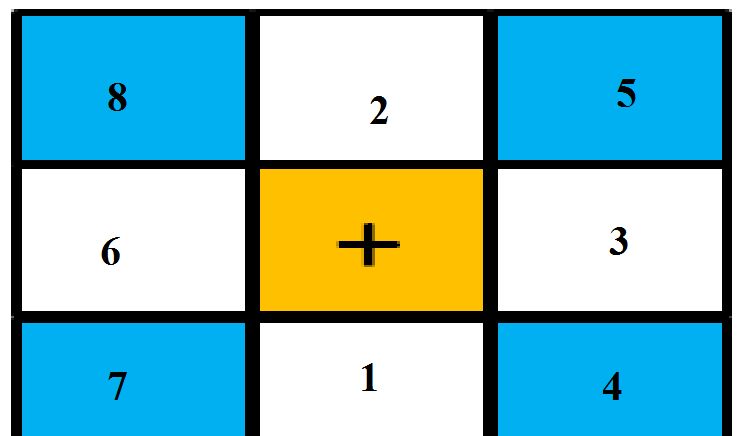
II. Addition

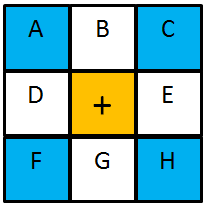
Arrange numbers 1 to 8 to replace the letters so:
A = B + D
C = B + E
F = D + G
H = E + G
To eliminate duplicates by rotation and reflection, let’s set the rules: A > C, A > F, A > H and F > C.
There is just 1 solution

Answer 1

SUBTRACTION

A=7
B=1
C=4
D=8
E=5
F=6
G=2
H=3
A>C
A>F
A>H
F>C

METHOD
I tried to get the higher values inside the result box. I chose 7 out of 7 and 8. And then, got the following things working -

8-1 = 7
8-2 = 6(Next big number)
5-1 = 4(Next big number)
5-2 = 3(Final big number)

ADDITION

A=8
B=2
C=5
D=6
E=3
F=7
G=1
H=4
A>C
A>F
A>H
F>C

METHOD

For addition, I tried getting the bigger numbers in the result box.

6+2 = 8(Biggest)
6+1 = 7(Next biggest)
3+2 = 5(Next biggest)
3+1 = 4(Final)

Answer 2

Difference 1

 5 8 2
 3 - 6
 4 7 1

Reasoning:

8 must go on a white square. 7 on an adjacent white is quickly dismissed. 1 must go on a blue square, otherwise we need another 7, and it can't be next to 8.

Difference 2

 7 1 4
 8 - 5
 6 2 3

Reasoning:

4 cannot go opposite 8, as 6 must be in a corner, which gives a 4-2 arrangement. And 4 cannot be adjacent to 8, and so must be in an opposite corner. 7 in the other opposite corner causes problems with placement of the 1.

Addition

 8 2 5
 6 + 3
 7 1 4

Reasoning:

We know A+B+C+D+E+F+G+H=36, and that A+C+F+H=2(B+D+E+G), so that B+D+E+G=12. There are only two ways to do this: 1236, 1245. In the second case 1 and 4 must be opposite, but 1+5=2+4. In the first 1 and 2 must be opposite, and 1+3$\ne$1+6$\ne$2+3$\ne$2+6.