Which side is White: the reds or the blues?

Question

Happy Halloween!

This problem by Miguel Ambrona, 2024, has the following stipulation. We don't know which way round the board is, but we are told that one player retains castling right. Which side is White: the reds or the blues?

This problem gives an example of a so-called "chess vampire", i.e. a position where the mirror reflected position is illegal. So it is thematic for Halloween. See chess.stackexchange for more details, or Miguel's Halloween-themed app, where you too can play with chess vampires. He cautions that he is only investigating one of the 16 vampire clans, and that moreover the deepest passageways of the dark vampire labyrinth are not yet fully mapped out. The puzzle above is from a different clan than the one featured in the game.

Answer 1

I will first find a proof game:

1. a3 a6 2. Nf3 Nf6 3. Nh4 Nd5 4. Ng6 Rg8 5. Nf4 Nf6 6. Ne6 Nd5 7. Nxd8 Nf6 8. Ne6 Nd5 9. Nf4 Nf6 10. Ng6 Ne4 11. Nh8 Nc3 12. Ra2 Nxd1 13. Ra1 Nc3 14. Ra2 Nxa2 15. Nc3 Nb4 16. Nb1 Nd5 17. Nc3 Nf6 18. Nd5 Ne4 19. Nf4 Nd6 20. Nfg6 hxg6 21. Kd1 Nc6 22. Ke1 Ne4 23. Kd1 Nf6 24. Ke1 Nh7 25. Kd1 Nd4 26. Ke1 Nb5 27. Kd1 Nc3+

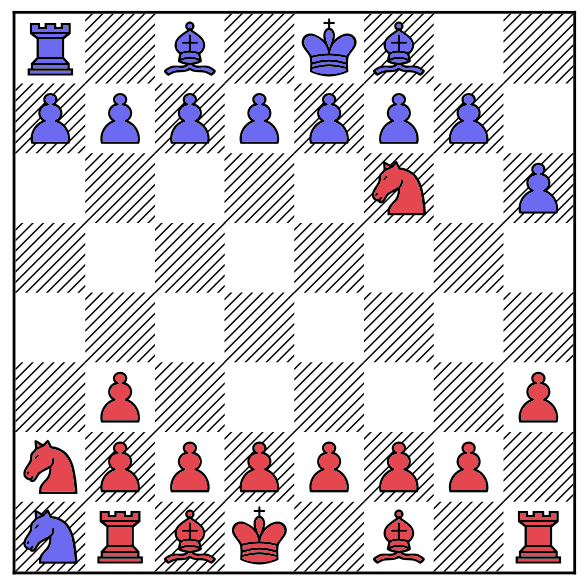
Here is the proof white is blue:

Suppose white is red. Then:

White cannot castle (king has moved) so black can castle (so a8 rook and black king did not move).

All 16 pawns are on the board, so no promotions.

Black cannot move the bishops and black has made exactly 1 pawn move. The black king and queen are stuck on d8 and e8. The black rooks are stuck on a8, b8, g8, h8, h7.

White has made one pawn capture (axb3). White could not have captured a rook, queen, bishop (because these black pieces cannot reach b3) or a pawn (black has 8 pawns) or a king (cannot reach b3) so white captured a knight on b3. The other black knight is on a1 so black made an even number of knight moves.

Black has made 0 queen moves (queen cannot move unless king is captured, which is impossible).

White has made 2 pawn moves, an even number of knight moves, an odd number of rook moves (b1 rook moved an odd number of times and h1 rook moved an even number of times), and an odd number of king moves. The white bishops cannot move. The white queen cannot move unless the white king is captured (which is impossible). So white made an even number of moves. Since white made the last move (black is in check), black made an odd number of moves.

So black's rooks moved an even number of times. Black's queenside rook moved an even number of times so Black's kingside rook moved an even number of times. This means Black's kingside rook was captured on h8 by a white knight (other white pieces and pawns cannot reach h8).

There are 3 events:
Event A is when white plays axb3. Event B is when white plays Nxh8. Event C is when white plays Nf6+. After white plays Nf6+, black must move the king (but black can still castle so this is impossible) or capture the knight (but white still has two knights). So event C was the last move and happened after event A and event B.

Call black's knight on a1 the A-knight and the other knight the B-knight. The A-knight must reach a1 from b3. After the B-knight is captured (event A), the A-knight cannot move (it cannot move to b3 or c2 as these are occupied by white pawns).

After event A and event B have both occurred, it is black to move. Black's A-knight cannot move. Black's B-knight is captured. Black's a8 rook cannot move. Black's kingside rook is captured. Black's queen, bishops and king never moved, and 7 of black's pawns never moved. The only black piece or pawn that black can still move is the h-pawn (which moved once in the game) so black could move the h-pawn at most once after event A and event B. So black made at most 1 move after event A and event B. But event A and event B cannot be the last move of the game, so black made exactly 1 move after event A and event B.

Then white played event C to end the game. The game must go [some moves, event A, some moves, event B, 1 move, event C] or [some moves, event B, some moves, event A, 1 move by black, event C].

Suppose the first possibility. Then the white knight on h8 must be on f7, g6 or h8 after event C but this is not possible.

Suppose the second possibility. Then the a2 square is empty just after event A but occupied by a white knight after event C so event C was a knight move to a2 and a knight move to f6. Contradiction.

So white is blue.