7
$\begingroup$

The following puzzle is a variant of a puzzle published in the May 8, 1926 issue of THE WINNIPEG TRIBUNE MAGAZINE:

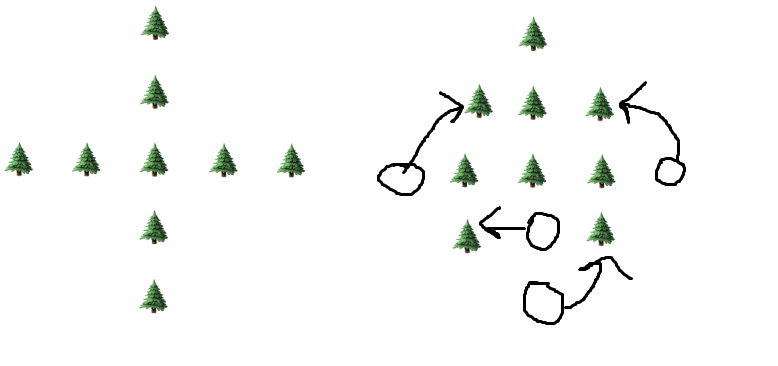
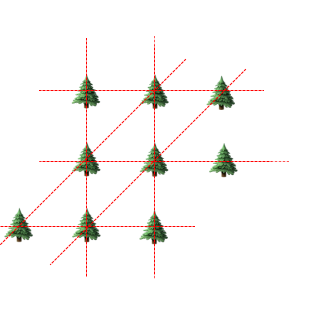
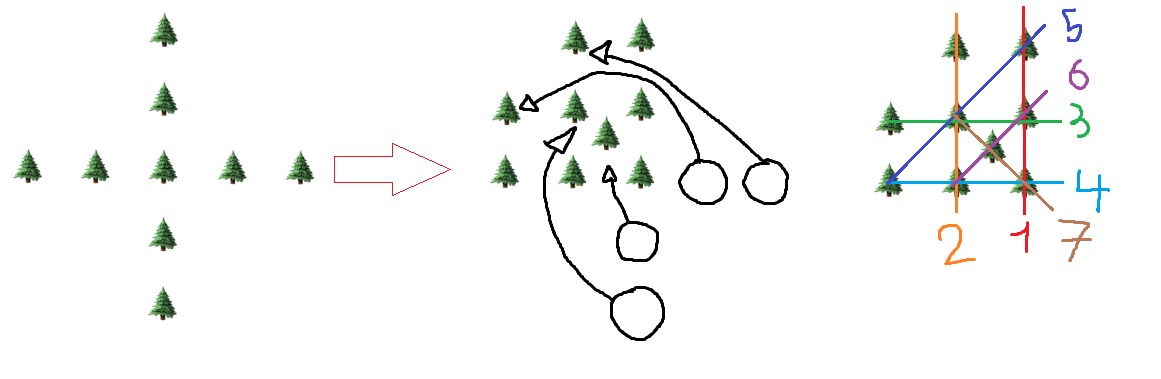
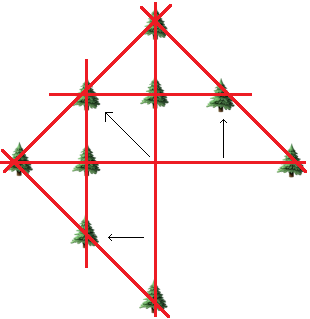
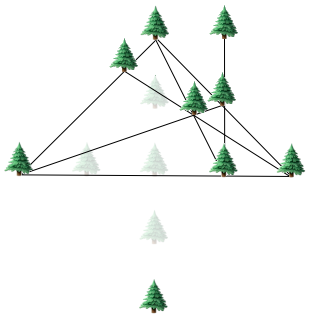
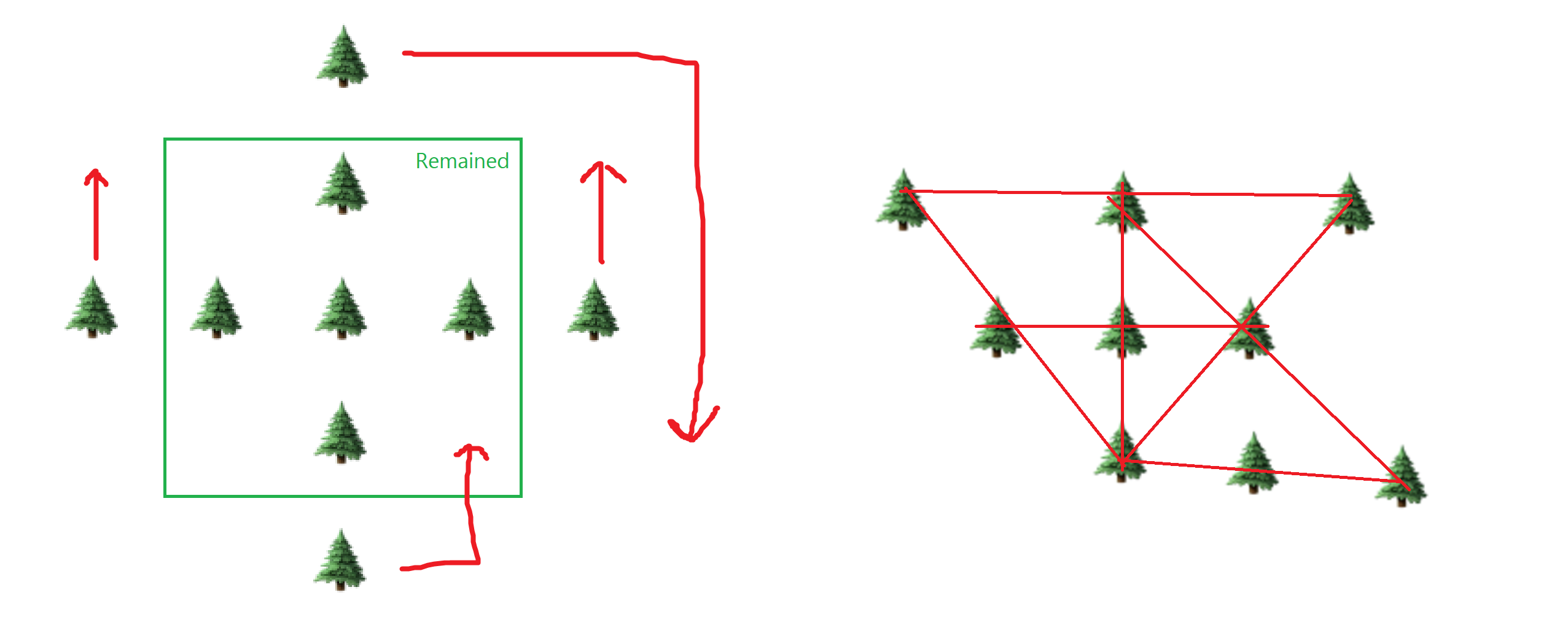
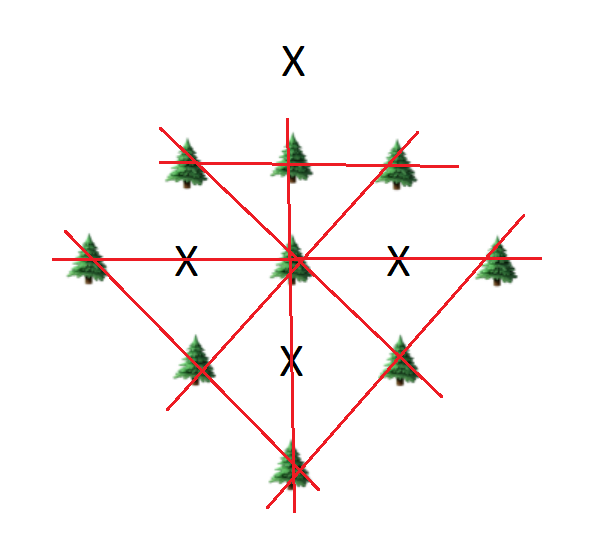
In the picture below there are nine trees arranged in two rows with five trees in each row. The puzzle is to reposition four of the trees leaving the other trees untouched so that you wind up with exactly seven rows with three trees in each row. Rows can be horizontal, vertical or slanted at any angle.

9 trees arranged in the shape of a PLUS sign (5 trees horizontal and 5 trees vertical)

Improve this question
$\endgroup$
6
  • 10
    $\begingroup$ I might be the odd one out, but I would argue this puzzle could be improved by replacing the word 'row' with 'line'. Calling two perpendicular lines rows seems weird/confusing to me? $\endgroup$
    – David Mulder
    Commented Aug 24, 2023 at 8:52
  • 2
    $\begingroup$ @DavidMulder I am sorry that the word "row" was confusing. However, "row" is the standard word for this type of puzzle (I checked a number of sources). I will update my question. $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 24, 2023 at 9:31
  • 2
    $\begingroup$ @DavidMulder A row of trees going north-south is as much as a row of trees going east-west. $\endgroup$
    – Pete Kirkham
    Commented Aug 24, 2023 at 13:12
  • $\begingroup$ @PeteKirkham Taking each row on its own I would wholeheartedly agree, but when you have e.g. a 2x2 grid I would say that that grid has two rows and two columns, not four rows and two columns. And I believe the same would apply to any set/group/collection of rows. $\endgroup$
    – David Mulder
    Commented Aug 25, 2023 at 6:14
  • 4
    $\begingroup$ You should clarify exactly 7 rows. My first thought was to arrange in a 3x3 grid. $\endgroup$
    – qwr
    Commented Aug 25, 2023 at 14:45

8 Answers 8

Reset to default
16
$\begingroup$

A different solution is possible:

enter image description here

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Nice symmetrical solution! Nicely explained too! $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 24, 2023 at 18:24
  • 1
    $\begingroup$ Just put them into a 3x3 box at first, then did stuff to get rid of one row. $\endgroup$
    – Nautilus
    Commented Aug 25, 2023 at 14:58
13
$\begingroup$

How about the following arrangement?

solution

Made from moving the four trees farthest from the center.

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ Yes, that works. Can you find a symmetrical solution? $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 24, 2023 at 0:56
  • $\begingroup$ @WillOctagonGibson Ah, yes, simply shift the top row over by one. I'll edit my answer $\endgroup$
    – DanDan面
    Commented Aug 24, 2023 at 7:42
  • 12
    $\begingroup$ This new solution makes 8 rows (not just 7), with the long diagonal from bottom left to top right... (This is also achieved by just positioning the 9 trees in a 3x3 square grid.) $\endgroup$
    – Stiv
    Commented Aug 24, 2023 at 7:51
  • 2
    $\begingroup$ if you move the left tree in the first row of your original answer down three rows then you get a symmetric solution with 7 rows (and not more) $\endgroup$
    – daw
    Commented Aug 24, 2023 at 8:53
  • 1
    $\begingroup$ My bad, I'll revert my edit. $\endgroup$
    – DanDan面
    Commented Aug 24, 2023 at 19:50
11
+150
$\begingroup$

I'm quite happy with the result, and had fun thinking about it

enter image description here

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ I like your symmetrical solution. You explained your answer well. $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 26, 2023 at 2:31
8
$\begingroup$

This doesn't quite follow the rules

but why move 4 trees when it can be done in 3?
enter image description here

Improve this answer
$\endgroup$
8
$\begingroup$

Yet another answer. Who said all the trees must be used?

enter image description here

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ Cool! Thinking outside of the box! $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 26, 2023 at 2:38
  • 3
    $\begingroup$ I like the way you show the original positions of the moved trees here. That's a really good visual representation. $\endgroup$
    – Toby Speight
    Commented Aug 26, 2023 at 9:41
6
$\begingroup$

I can actually make 9 rows with 3 trees in each row by just moving 4 trees. I will keep the top and bottom tree and the three center ones in place and move the other 4 to build the following pattern (F for fixed trees and M for the ones I moved).

solution

Sorry for not actually having nice trees in my picture. Feel free to edit.

Improve this answer
$\endgroup$
2
  • $\begingroup$ Ideally I am looking for a solution with exactly 7 rows. $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 24, 2023 at 9:18
  • 3
    $\begingroup$ Move the top right M further out along its line and you eliminate 2 rows to leave 7. $\endgroup$
    – Ergwun
    Commented Aug 24, 2023 at 12:32
2
$\begingroup$

I'm not sure if this would be allowed, because this solution involves

trees being not exactly grid aligned, and also one row having way different spaceing between trees. Though, I did try to keep the distance within each row the same, because this would be really easy if any adjacent trees in a row can be any distance apart.

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ That looks perfectly good to me, except you forgot to draw one line. $\endgroup$
    – Florian F
    Commented Aug 27, 2023 at 20:40
  • $\begingroup$ @FlorianF That looks like seven lines to me though.. Three horizontal, one vertical, two negative diagonals, and one positive diagonal. $\endgroup$
    – Kusane Hexaku
    Commented Aug 28, 2023 at 4:53
  • 1
    $\begingroup$ Oops, it seems I cannot count. I saw an additional row from the top left to the bottom right tree, but I didn't see that you moved the bottom right tree to avoid that. So no objection. Grid alignment was not a requirement. $\endgroup$
    – Florian F
    Commented Aug 28, 2023 at 8:51
2
$\begingroup$

Found this one:

Answer

Here's how I did it.

Explanations

Improve this answer
$\endgroup$
1
  • $\begingroup$ Nice symmetrical solution. $\endgroup$
    – Will.Octagon.Gibson
    Commented Aug 29, 2023 at 20:09

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.