World Tour of Planet Rhombicosidodecahedria

Question

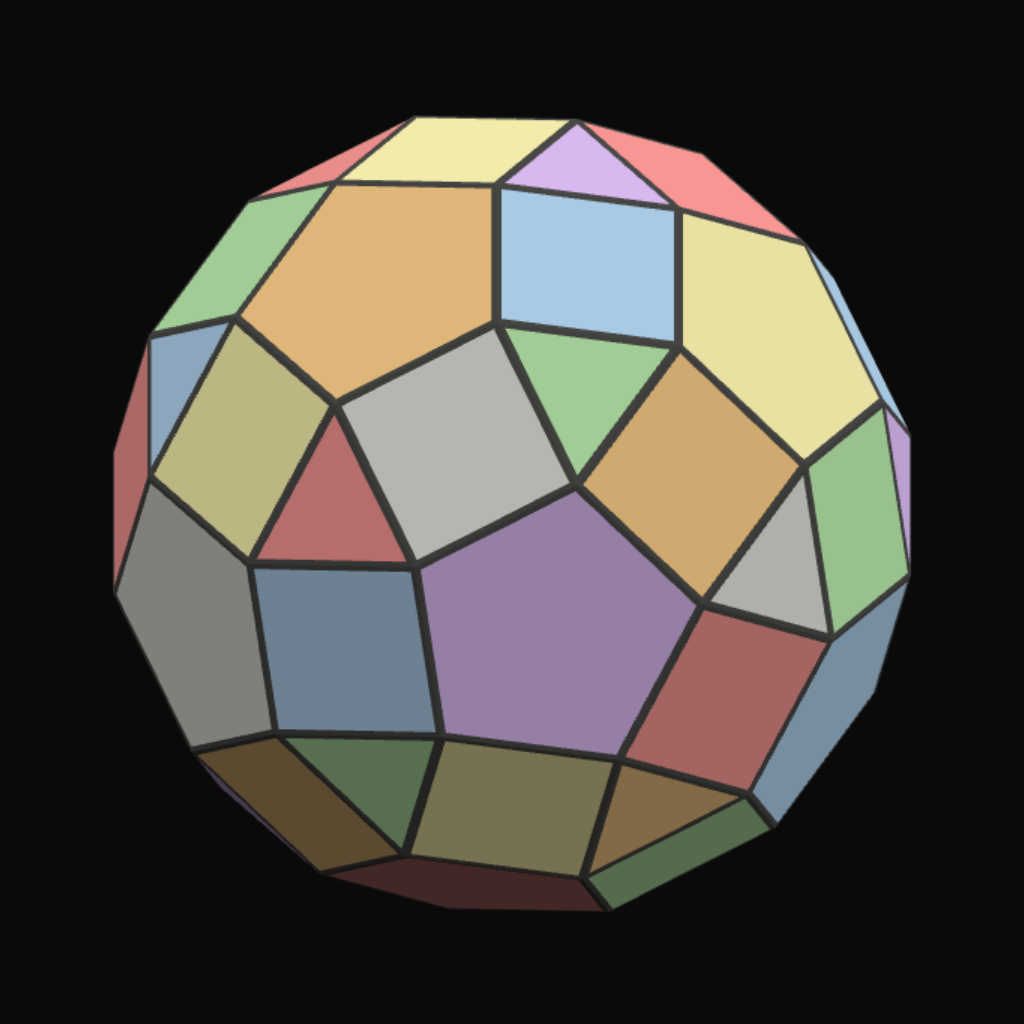
This is the planet Rhombicosidodecahedria:

This lovely planet has 62 countries, each with its own distinct history and culture. By an amazing coincidence, the countries all happen to coincide perfectly with the faces of a rhombicosidodecahedron. You, as a tourist, are naturally hoping to visit all of them.

How many different ways can you travel though each of the countries on this planet, visiting each exactly once?

Some rules:

• Your tour may start in any country you wish.
• You must visit each country exactly once. Once you have visited a country, you cannot visit it again. (The word "tour" should not be taken to imply a requirement that you return to your starting point.)
• You may only move between countries that are adjacent. In other words, each new country you visit must share a border with the previous one. (If they just touch at the corners that doesn't count.)
• For purposes of this puzzle, every country should be treated as unique, and reflections and rotations all count as different paths. (In other words, the orange pentagon counts as a different starting point from the purple pentagon.)

Answer 1

There are exactly

Zero ways to complete such a tour.

Proof:

There are 12 pentagon countries and 20 triangle countries. Of these 32 countries, no two share a border. Any path visiting each of these countries exactly once would necessarily pass through 31 other countries, but there are only 30 square countries. At least one square country must be visited twice.