9
$\begingroup$

And it's the Math Test Day again! What a great day!

You are given this task (imagine the teacher writing on the blackboard if it helps you in any way):

Find a way to cut any given pentagon with a straight line into two equal parts.

That is, you have to find how to cut any given (convex) pentagon into two parts with an equal area, using a single straight cut, starting at one point of the circumference, and ending at another one. There are no tricks here, no folding, nothing like that.

And the test starts... Now! You have exactly 45 minutes left 'till the test ends. (caused misconceptions)

So, how exactly can you do this?

Improve this question
$\endgroup$
8
  • $\begingroup$ is it perfect pentagon? $\endgroup$
    – Oray
    Commented Oct 24, 2016 at 9:46
  • 1
    $\begingroup$ Is it 45 mins from when you posted the question or 45 mins from when we read it? $\endgroup$
    – deep thought
    Commented Oct 24, 2016 at 9:53
  • 1
    $\begingroup$ @Oray There is "any given convex pentagon" $\endgroup$
    – oleslaw
    Commented Oct 24, 2016 at 10:03
  • 3
    $\begingroup$ Can we use a straightedge? A compass, protractor, divider? $\endgroup$
    – frodoskywalker
    Commented Oct 24, 2016 at 11:37
  • 2
    $\begingroup$ I'm assuming you can't just cut it along the plane of the actual pentagon (that is, so you end up with two of the same pentagon - the "front" and "back" parts)? $\endgroup$
    – Glen O
    Commented Oct 24, 2016 at 16:24

9 Answers 9

Reset to default
9
$\begingroup$

Let $A,B,C,D,E$ - vertices of the pentagon.

Draw a line $AB$.
Draw a line $c$ paralel to $BD$ and passing through $C$. Find $C'$ as an intersection of $c$ and $AB$.
Draw a line $e$ paralel to $AD$ and passing through $E$. Find $E'$ as an intersection of $e$ and $AB$.
Find $M$, a midpoint of the $C'E'$ segment.

Segment $DM$ cuts the pentagon $ABCDE$ into halves.

Whoops, I just realized the method fails, if...

if the $M$ point is not between $A$ and $B$.

It almost surely can be solved by appropriate choice of the first side of the pentagon, but I'm not sure now, which one is appropriate...

A fix:

If $M$ falls outside the $AB$ segment, say past the $B$ end, then we need to shift it to the original pentagon's edge in such a way, that the 1:1 area ratio is preserved.
This can be done with the help of an $m$ line, passing through $M$ and parallel to $BD$. It intersects $BC$ at some point $P$ and triangles $BDP$ and $BDM$ have equal areas, hence pentagon $ABPDE$ keeps its area equal to the triangle $E'MD$ which is half of $ABCDE$.
Finally $DP$ is a desired cut.

Here is a picture:


enter image description here

Improve this answer
$\endgroup$
10
  • $\begingroup$ Does it even matter if M is not between A and B? I think this answer might be correct, even though I think it needs some explaining as to why it is correct $\endgroup$
    – Ivo
    Commented Oct 24, 2016 at 14:23
  • $\begingroup$ Example with M between AB: i.sstatic.net/SdlR1.png Example with M outside AB: i.sstatic.net/iKbkT.png If I had to guess both look right to me $\endgroup$
    – Ivo
    Commented Oct 24, 2016 at 14:26
  • $\begingroup$ @IvoBeckers I'm afraid it does. Triangles $BDC$ and $BDC'$ have equal areas, as they share the $BD$ base and a height (the distance between $BD$ and $c$). Similary the other side. Then triangle $E'C'D$ has area equal to the pentagon's, and I half it by halving the base. However if $M$ falls outside $AB$, say at $B$ side, then $DM$ cuts $BC$ at $Q$ and there appears a small trangle $BMQ$, which belongs to $E$-side half-triangle, but does not belong to $E$-side half of pentagon. Hence the pentagon's area is not halved in this case. $\endgroup$
    – CiaPan
    Commented Oct 24, 2016 at 14:34
  • 1
    $\begingroup$ I expect choosing the longest side for $AB$ will suffice, but it's no more than feeling now; it still needs a proof. $\endgroup$
    – CiaPan
    Commented Oct 24, 2016 at 14:39
  • 1
    $\begingroup$ @BmyGuest Here it is. Just for completeness, not for upvotes :-) $\endgroup$
    – CiaPan
    Commented Dec 30, 2016 at 19:14
4
$\begingroup$

One way to construct the line:

1. Divide the pentagon into quadrangle and triangle, by connecting any two of its non-adjacent vertices
2. Find the centroid of the triangle as an intersection of its medians
3. Find the centroid of the quadrangle - there are two ways how to divide it in two triangles - for both of these connect the triangles' centroids - intersection of these two lines is the quadrangle's centroid
4. The answer is the line crossing both the centroids

This works because

any line crossing centroid of a triangle or a quadrangle divides it into two parts with the same area - therefore the line we constructed divides the pentagon in two parts, each having equal area of the triangle and the quadrangle

Improve this answer
$\endgroup$
5
  • 4
    $\begingroup$ I don't think it's necessarily true that the intersection of the diagonals of a quadrangle coincides with the centroid. You may have to come up with a different way to construct this. $\endgroup$
    – hexomino
    Commented Oct 24, 2016 at 12:18
  • $\begingroup$ you're right, i'll fix that. $\endgroup$
    – pepo
    Commented Oct 24, 2016 at 12:29
  • 3
    $\begingroup$ jwilson.coe.uga.edu/emt668/EMT668.Folders.F97/Patterson/… $\endgroup$
    – paparazzo
    Commented Oct 24, 2016 at 12:30
  • 4
    $\begingroup$ "any line crossing centroid of a triangle or a quadrangle divides it into two parts with the same area". This is definitely not true. Just consider a regular triangle with a line through the centroid parallel to one of the sides. $\endgroup$
    – Ivo
    Commented Oct 24, 2016 at 14:05
  • 1
    $\begingroup$ More info on centroids and triangle area bisectors, most of which do not pass through the centroid. $\endgroup$
    – user2357112
    Commented Oct 24, 2016 at 16:57
4
$\begingroup$

I know not maths to my eternal shame but

could you draw a square/rectangle around the pentagon where one line is on top of a line in the pentagon and the other three lines touch the remaining verts. Then calculate the area of the triangles formed around it. Then draw a line straight down from the vert opposite the line touching the square (parallel to the side of the square). Then draw a line from that same vert to form a triangle with an area equal to the difference in area between the two outer triangles on either side and cut along the diagonal line?

I don't expect this to be correct and I haven't showed full working but I'm hoping that this could at least be "helpful" to someone.

enter image description here

I realise I probably got the equation on the picture back to front, but there is probably a more correct way to express a calculation of the difference between two sums anyway?

Improve this answer
$\endgroup$
4
$\begingroup$

Building upon the "rectangle around pentagon" idea, I think I can assert that...

You can definitely do this problem.

I have yet to do it by hand for reasons you will see, but I have basically solved this for all obtuse pentagons.

Step 1. The box.

BOX
Simple as simple goes...

Step 2...

... Define multiplication with construction.
You can read about this at our friend here: https://math.stackexchange.com/questions/139340/representing-the-multiplication-of-two-numbers-on-the-real-line

Step 3.

Find the distance from the bottom left corner of the rectangle, to the median of the pentagon. Using tons of algebra, of course.

Note:

With multiplication and trivial addition/subtraction and somewhat-trivial division defined, we can solve for the median's position by first finding the areas of the four triangles, subtracting them from the rectangle, and dividing the result by 2. This returns the area enclosed by half of the rectangle, and the pentagon's median. This is a trapezoid. Henceforth, we use trivial algebra. All operations are well defined by construction after the base unit is defined as well.

Example:

Here is the double the area of the bottom-right triangle stored as a length:
COMPLICATED

Similar constructions can be used to solve for the area of an "acute", or whatever-you-name-it, pentagon.

If the internet demands that I finish the construction for internet points, I will consider it when I finish reading my history textbook. Peace.

Improve this answer
$\endgroup$
1
  • $\begingroup$ I demand that you finish the construction! Because I still haven't wrapped my head around this, and actually I can't even remember how I thought this would work... $\endgroup$
    – Brent Hackers
    Commented Apr 13, 2017 at 9:17
3
$\begingroup$

How about this algorithm?

If we imagine that we have constructed this pentagon as, say, a piece of card, and place a pin in one of its vertices, we hang the polygon from that vertex against a wall. We allow it to freely rotate, so that its own weight ensures an equal distribution to either side of the pin. We then hang a plumb line from the pin, and that line is the line that divides the pentagon in half.

As jwg pointed out in the comments, this unfortunately doesn't actually work since the line passing through the centre of mass isn't necessarily on the line dividing the area in half.

Improve this answer
$\endgroup$
6
  • 2
    $\begingroup$ Maybe this method can be improved to one not requiring "weighing". You are hardly ever able to do such things during a test :) $\endgroup$
    – oleslaw
    Commented Oct 24, 2016 at 11:38
  • 4
    $\begingroup$ I love this solution. Sure it won't work in an exam room scenario, but when I can only kind of sort of understand people's answers to a maths problem, and then someone comes along with a (sort of) none-maths answer that I can actually fully understand, well... I love this solution. +1 $\endgroup$
    – Brent Hackers
    Commented Oct 24, 2016 at 13:17
  • 13
    $\begingroup$ The problem with this method is that it doesn't work. Because weight which is further from the fulcrum contributes more torque, the line goes through the center of gravity, but doesn't divide the area into two. One example is if you have a pentagon which is close to a triangle and you hang it up so that one edge is vertical - the piece which is next to that edge will have 5/9 of the area. $\endgroup$
    – jwg
    Commented Oct 24, 2016 at 16:27
  • 1
    $\begingroup$ Nice, but it doesn't work. That is a method to find (an approximation of) a center of mass. And, as Ivo Beckers points out in this comment, the line passing the centroid does not, in general, half the area. $\endgroup$
    – CiaPan
    Commented Oct 24, 2016 at 20:05
  • $\begingroup$ "There are no tricks here, no folding, nothing like that." And it does not work. $\endgroup$
    – paparazzo
    Commented Oct 24, 2016 at 21:22
2
$\begingroup$

We should be able to:

1. Strategically enclose the pentagon in a rectangle such that we can exactly halve the rectangle and quite approximately halve the pentagon with a single line parallel to 2 of the rectangle's sides. This line shall also be completely within the pentagon. (ie This line goes from one side of the pentagon to another, or from one side to a vertex.)
2. Figure out the area of each half of the pentagon, then subtract to find the positive difference. We halve this difference (D) to know what we need to take from one side and give to the other.
3. Assuming D is not 0, duplicate our line and move one of its points along the side of the rectangle/pentagon (towards the bigger side) until we've created a triangle with an area equal to D/2.
4. If we drew our rectangle properly, this 2nd line will halve the pentagon and still remain within it.

Some math:

(BL)/2 = D/2, B is base, L is the length of our first line
BL = D
B = D/L, B is how far we move along the edge of the rectangle/pentagon with 1 point of the 2nd line

I just realized I had the same idea as Brent.

Improve this answer
$\endgroup$
1
  • $\begingroup$ At least you knew the maths (I assume). Sadly it goes over my head a bit. +1 $\endgroup$
    – Brent Hackers
    Commented Oct 25, 2016 at 6:24
2
$\begingroup$

This is a reply to the answer by helps_7766,
and it should be posted as a comment, but it's too long, so I decided to post it as an answer.

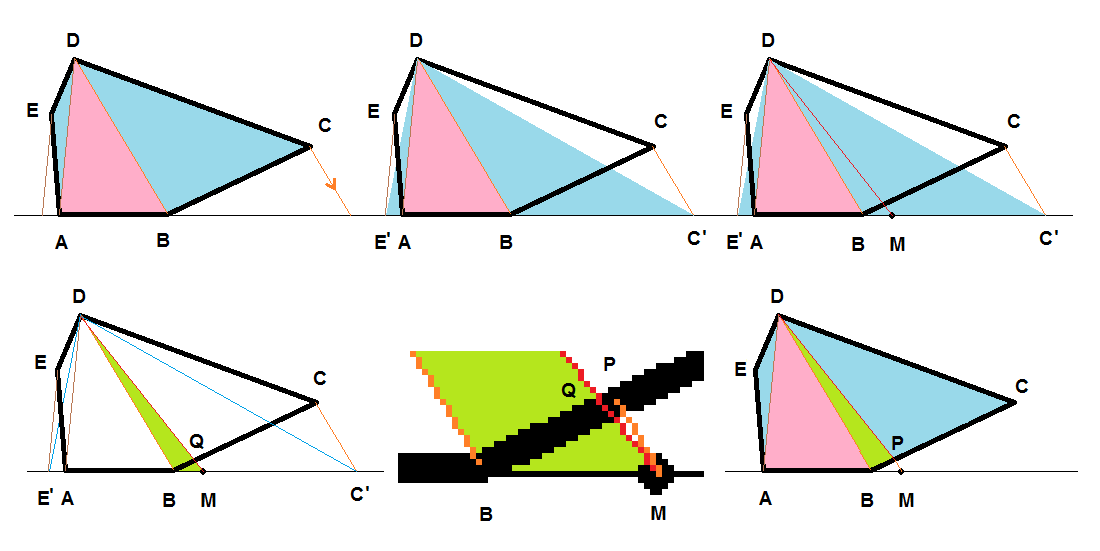
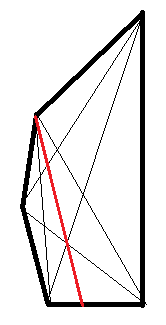
Unfortunately, the method presented does not 'yield the center of mass of the pentagon'. As a counterexample let's see the image:

triangle to pentagon

The center of mass of the black triangle (on the left) is at $1/3$ of its height.

If we add two narrow red triangles along the black triangle's sides, with their 'free' vertices close to the central triangle's base (in the centre), then their centers of mass lie close to the $1/3$ of the new figure's heigth, too. Thanks to the symmetry, the triangle defined by the three centers (blue dots) will have its resulting center (marked with a blue diamond) somewhere close to the center of mass of the black triangle.

However, if we shift the fourth and fifth vertex close to the apex (image to the right), the centers of mass of red triangles will also move up – up to $2/3$ of the figure's height. Then the resulting triangle defined with blue dots will have the center of mass (blue diamond) at approx. $\left(\frac 13 + \frac 23 + \frac 23\right)/3 = 5/9$ of the figure's height.

Please note that we can make the red triangles as thin as we want, effectively obtaining a spatial distribution of the mass of our pentagon indistinguishable from the black triangle. Anyway, the resulting point will appear at positions described above.

That's because the three blue points' heights depend linearly on the heights of vertices of respective triangles, but they do not depend on the triangles' areas. Hence they can be shifted significantly with appropriate vertex shifts with no significant change in the area.

As a result, the supposed 'center of mass' has little to do with actual mass distribution and with an actual center of mass.

Not to mention a line through the center of mass does not half the area, usually. For example a 'horizontal' line through the triangle's center of mass divides its area as $\frac 49$ (the top triangular part) to $\frac 59$ (the bottom trapezoid part). If you want to half a triangle with a line parallel to the base, you should draw it at $1-\frac 1{\sqrt 2}\approx 0,293$ of the triangle's height, that is approx. $\frac 1{25}$ of the triangle's height below the center of mass!

Improve this answer
$\endgroup$
0
$\begingroup$

Like this?

I don't think this works for most unfortunately


enter image description here

You draw the diagonals and then draw a line through the middle pentagon

I have used an irregular pentagon here and the green line is the half line

You have to use your brain where you draw the line, for instance oleslaw gave the example:


enter image description here

Where it obviously isn't equal, however it would be if you draw the line more horizontally through the middle pentagon

Improve this answer
$\endgroup$
8
  • 1
    $\begingroup$ This wouldn't work for a "less perfect" pentagon. At least with this amount of details. $\endgroup$
    – oleslaw
    Commented Oct 24, 2016 at 10:11
  • $\begingroup$ @oleslaw well I was using an irregular pentagon in the pic and it has to be convex $\endgroup$
    – Beastly Gerbil
    Commented Oct 24, 2016 at 10:15
  • 2
    $\begingroup$ How about this pentagon: i.sstatic.net/i9Hv6.png ? I believe I used your algorithm, but the halves are certainly not equal areawise. Maybe selection of the vertex to start from is important? $\endgroup$
    – oleslaw
    Commented Oct 24, 2016 at 10:20
  • $\begingroup$ @oleslaw Good counterexample. Selection of the vertex to start from in Beastly Gerbil's method won't always work, because for any candidate vertex to start from, you could just move all the vertices so that they, and the dividing line, are in oleslaw's counterexample diagram. Any method must work for any positions of the vertices that make a convex pentagon. $\endgroup$
    – Rosie F
    Commented Oct 24, 2016 at 10:31
  • 1
    $\begingroup$ But even a horizontal line through the rightmost vertex of the inner pentagon won't create two halves of equal area. $\endgroup$
    – M Oehm
    Commented Oct 24, 2016 at 11:18
0
$\begingroup$

Consider the pentagon ABCDE, divide it into three triangles and find their centroids, then find the centroid of the triangle formed from those three points.

This yields the center of mass of the pentagon and a line drawn from it to any of its vertices bisects the area of the pentagon.

Improve this answer
$\endgroup$
2
  • $\begingroup$ imgur.com/a/CRdi7 $\endgroup$
    – greenturtle3141
    Commented Oct 25, 2016 at 0:57
  • 1
    $\begingroup$ No, this method does NOT yield the center of mass. And even if it did, a line through the centre of mass does NOT, in general, half the area of a figure. Please see this answer. $\endgroup$
    – CiaPan
    Commented Oct 27, 2016 at 21:48

Not the answer you're looking for? Browse other questions tagged or ask your own question.