11
$\begingroup$

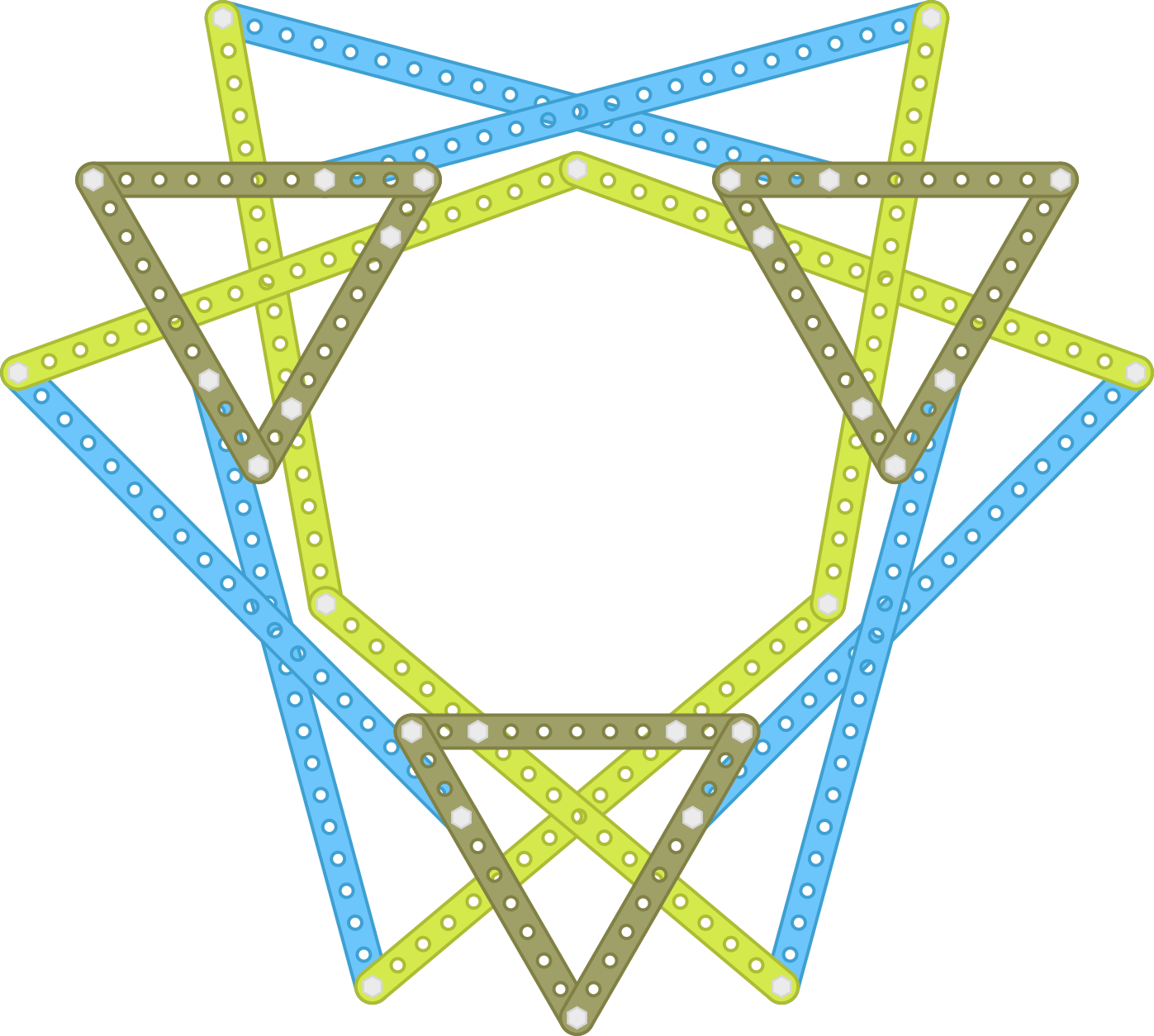
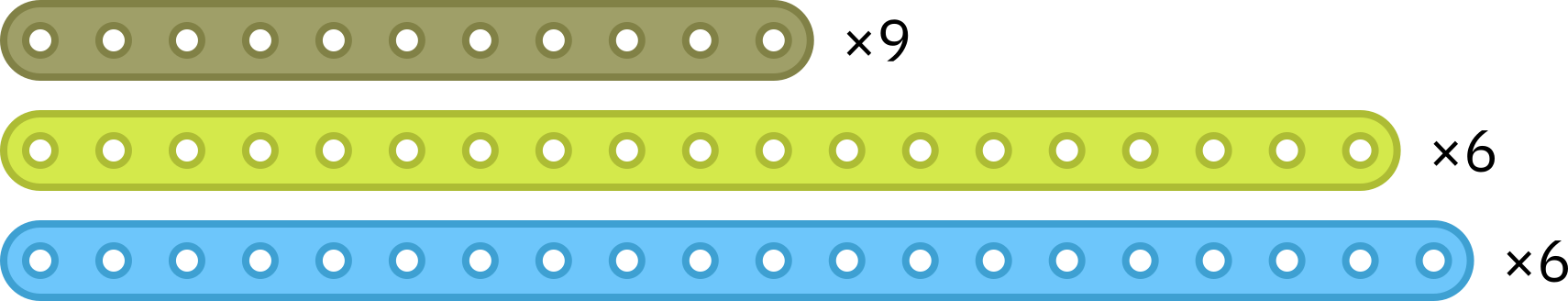
You are given 21 Meccano strips, where the distance between adjacent holes is 1 unit:

  • 9 strips of length 10 (hence having 11 holes)
  • 6 strips of length 18 (19 holes)
  • 6 strips of length 19 (20 holes)

By inserting nuts and bolts in the holes so as to form hinges between the strips, it is possible to create a rigid framework where

  • portions of nine different strips form a perfectly regular nonagon of side length 6 (with vertices coincident with hole centres)
  • no strip is redundantly long, i.e. all end holes are part of some hinge linking at least two different strips

Can you find this rigid framework and prove its rigidity?

Improve this question
$\endgroup$
5
  • $\begingroup$ Do you already know the answer, and are posing it as a puzzle, or do you not know the answer, and are asking out of curiosity? $\endgroup$
    – user21820
    Commented Dec 3, 2021 at 14:38
  • 1
    $\begingroup$ @user21820 I know the answer and it is somewhere on MSE. $\endgroup$
    – Parcly Taxel
    Commented Dec 3, 2021 at 15:14
  • $\begingroup$ My first guess is no simply because it's not constructible, but if you say it's "yes" then I've no idea. $\endgroup$
    – user21820
    Commented Dec 3, 2021 at 15:25
  • $\begingroup$ @user21820 for what it's worth, I have found the solution on MSE, and it is not what I expected - if anyone is still interested in this puzzle, your first instinct to use the 9 identical pieces as the sides and the others as scaffolding is terribly wrong :-) $\endgroup$
    – htmlcoderexe
    Commented Apr 29, 2022 at 12:11
  • $\begingroup$ @htmlcoderexe: Yes I saw that post already last year because I went to look for it. Thanks for telling me though! =) $\endgroup$
    – user21820
    Commented Apr 29, 2022 at 12:16

2 Answers 2

Reset to default
1
$\begingroup$

The answer from my MSE question, drawn in the style of the picture in this question:

Improve this answer
$\endgroup$
0
$\begingroup$

The MSE answer is mathematically correct, appropriate for that site. But the puzzle on this site just asks for a nonagon that is "part of" strips about a unit wide. A much simpler answer fits that criterion, based on triangles with sides of 4,4, and 6 units

Bolt the ends of two 10-unit strips together, then bolt two holes six units apart on another strip to holes 4 units from that hinge. (I used the holes 2 units in from the ends of another 10-strip in the figure below.) This gives a 4,4,6 triangle with rigid 6-unit extensions at an angle close to the 140 degrees in a regular nonagon. Make 2 more of these. Connect the ends of the arms to get a slightly irregular nonagon. The vertices of a regular nonagon, shown in black, are within about 1/9 of a unit (~0.11115916 units to be more precise) of the center of the bolts, probably within the radius of the bolt and definitely "part of" the strips.

To meet the second criterion, connect the extra ends of each strip used for the long side of a triangle to two 18- or 19-unit strips and connect the other ends of those strips to each other. (I used two 18-units strips in the figure.) Nine strips of 10 units each in a slightly irregular nonagon, with six strips of 18 occupying the leftover ends. Regular nonagon in black.

Improve this answer
$\endgroup$
1
  • $\begingroup$ If that was the answer, I would have provided only 6 length-10 and 3 length-6 strips. $\endgroup$
    – Parcly Taxel
    Commented Nov 24, 2022 at 6:06

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.