21
$\begingroup$

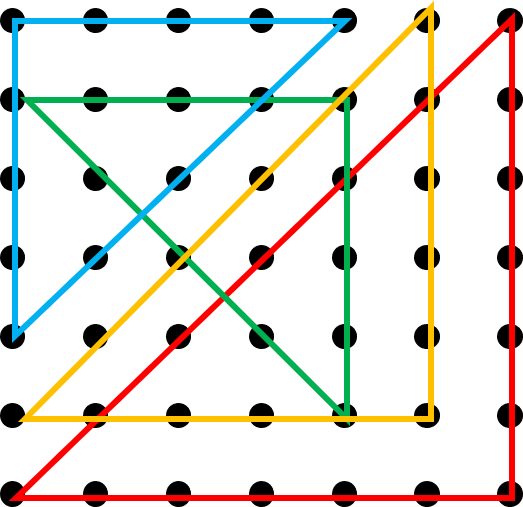
49 dots are drawn as a 7x7 regular square grid. Can you draw 4 triangles that pass through every dot? The corners of the triangles must lie on the dots, ie., they cannot lie outside the grid.

The 7x7 puzzle is a harder version of the 5x5 puzzle: Three triangles passing through every dot of a 5x5 grid

Improve this question
$\endgroup$
3
  • $\begingroup$ @msh210 Changed the wording to make it clear. $\endgroup$
    – Dmitry Kamenetsky
    Commented Jun 28, 2021 at 7:34
  • 2
    $\begingroup$ It is even less clear than before (a slight difference between "this is a link:" and "here is a link: ...". But information from the other puzzle clarifies that 4,7x7 is harder than 3,5x5. $\endgroup$
    – z100
    Commented Jun 28, 2021 at 7:45
  • $\begingroup$ I can get all but one... $\endgroup$
    – emanresu A
    Commented Jun 28, 2021 at 8:50

1 Answer 1

Reset to default
24
$\begingroup$

The construction:

enter image description here

I don't know if it's the only solution, but that's the only one I found.

Improve this answer
$\endgroup$
7
  • 5
    $\begingroup$ That last triangle (green in your picture) is so hard to find / easy to overlook! This is a great puzzle and well done on finding the solution. I'm almost sure it is the only solution (up to rotation/reflection). $\endgroup$
    – Jaap Scherphuis
    Commented Jun 28, 2021 at 9:52
  • $\begingroup$ Correct and well done! Yes that green triangle is very hard to find. If we use the approach in the previous 5x5 puzzle then it is not too hard to find a solution with one dot missing. But getting that extra dot is the challenge here. $\endgroup$
    – Dmitry Kamenetsky
    Commented Jun 28, 2021 at 9:54
  • 1
    $\begingroup$ @DmitryKamenetsky Thanks! Nice puzzle! $\endgroup$
    – Jerry Dean
    Commented Jun 28, 2021 at 9:56
  • 1
    $\begingroup$ @DmitryKamenetsky I planned to change it every 3 months or so to keep track of how I'm doing here ;) (That's why I wrote the date as well) $\endgroup$
    – Jerry Dean
    Commented Jun 28, 2021 at 11:10
  • 3
    $\begingroup$ I did the computer search. It is the only solution modulo symmetries and permutations of the triangles. And btw if you go for non-right triangles you need 6 of them. $\endgroup$
    – Florian F
    Commented Jun 30, 2021 at 21:24

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.