Toroidal Pipes Puzzle: T's and Bulbs Only

Question

A continuation in the Pipes puzzle series.

Problem statement for math nerds: Let $G(N)$ denote the graph consisting of cardinally adjacently linked lattice points on an $N \times N$ toroidal grid. For which $N$ does there exist a spanning tree of $G(N)$ in which every vertex has degree $1$ or $3$?

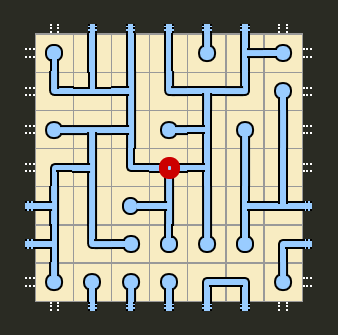
On the same website I linked to in the last problem, there is a curious variant of Pipes that removes the borders of the game, and instead allows pipes to wrap around in their solution (but as always, they must remain loop-free):

                                      

One reason I like Pipes played on a torus besides the added difficulty is that it is more essentially minimalist; there is no "edge" and "center," instead we have a topologically homogeneous space.

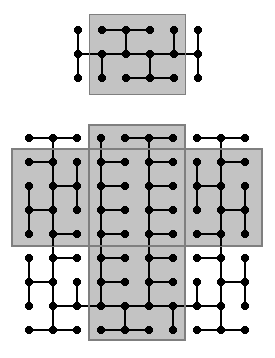
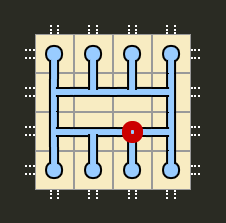
What if we endeavored to make a version that's even more minimalist, and even more difficult? There are four types of tile that may comprise a Pipes puzzle: The bulbs with only one neighbor, the corners and straights with two neighbors, and the T's with three neighbors. We obviously need to keep the bulbs in any version of the game, and among all the tiles I would say in my experience playing Pipes that T's are the most ornery, so let's also keep them. The only problem is: Are there even any (square grid) Pipes puzzles to play when you use only bulbs and T's?

(Extra credit: What is the situation—with and without border wrapping—on arbitrary $m \times n$ grids?)

Answer 1

Suppose we have a solution, which is a tree graph with vertices of degree 1 and 3 only. This graph has the following property:

Any tree graph can be given a 2-colouring of vertices. This is a well-known fact that is easily proved by induction.
These bulb and T graphs have a stronger property: the number of vertices of each colour is odd.

This can be proved by induction:

This is obviously true for the base case where $v=2$, a graph consisting of two bulbs connected together. Induction step: Find any T vertex that has (at least) two neighbouring bulbs. This must exist, because otherwise you could travel from T vertex to T vertex indefinitely without retracing your steps, and this is not possible in a finite tree. You can replace this T and the two neighbouring bulbs by a single bulb. This reduces the number of vertices by 2, but both are of the same colour so the parities of the colours do not change. The resulting smaller tree graph must have an odd number of vertices of each colour by the induction hypothesis, and therefore so does the original tree graph.
By induction, the number of vertices of each colour is odd.

This property has some consequences for the $M\times N$ grids that can be filled with Ts and bulbs.

Clearly the total number of vertices is odd+odd=even, so $MN$ is even.
Furthermore, $M$ and $N$ cannot both be even. The colouring of the tree graph induces a 2-colouring on the grid, which is the essentially unique checkerboard colouring. This has an even number of squares of each colour, which contradicts that there must be an odd number of each.
Together this means that exactly one of the dimensions of the grid must be even.

If the grid is not on a torus, but a rectangular grid with hard borders, then we can strengthen this to say that the even dimension cannot be a multiple of 4. Here the checkerboard colouring of the grid is forced, and the colouring of each line in the even direction has an even number of each colour, and therefore this is not possible for the same reason. This does not necessarily hold on a torus because then there can be paths between adjacent squares of even length by wrapping around the board in the direction of the odd-dimension.

For square grids $N\times N$ this means that

there are no solutions.

Edit:

Here are pictures of general solutions for some grid sizes, not necessarily toroidal.

The top shows a general solution for a $3\times(4n+2)$ rectangle.
The bottom is a general solution for any $(4m+5)\times(4n+6)$ rectangle.

Edit 2:

Here is a solution for a grid that only works on a torus:

A toroidal (or cylindrical) solution for a $3\times4$, which cannot be solved without wrap-around borders at the top/bottom. By repeating the middle section it can be extended to any even length.

Answer 2

To complement Jaap's excellent answer, a family of non-toroidal grids for odd length $4k+3$:

(and a family that allows $k=0$, for good measure:)

EDIT: And a family covering all the toroidal grids:

Answer 3

I thought that I'd found counter-examples to the proofs given in the other answers:

However, I then realised that

These violate the constraint that the solution must not contain loops.

Instead we can

use these as a base of a more intuitive proof of impossibility, or a way to more easily visualise why those proofs work...

In particular,

to convert these non-solutions into a solution, we would need to break the loop in precisely one place, which could be trivially done by converting two adjacent T pieces into a straight or turn piece. However, other changes to convert these back to either a bulb or a T piece will necessarily involve making or breaking a link, which will either reconnect the loop, or create a disconnected section.