How do you arrange 6 pencils so that each one touches the other five? And what about 7 or 8?
$\begingroup$
$\endgroup$
0
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
1
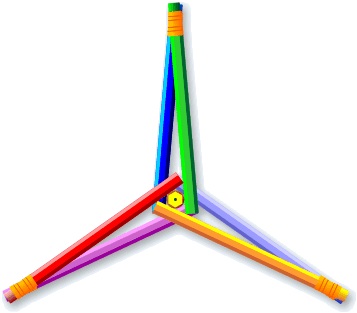
You need 2 layers. First arrange 2 pencils that spread outwards touching by the erasers, like a V, then slide one with the pencil tip in between. Then you can repeat the process with 3 more, sideways, laying on the original, this achieves 6:
You can also achieve an arrangement of 7 by making 3 V's of 2, an arranging them like a ninja star (not really sure how else to explain it) you may need to tie them with the V's because 1 pencil has to be on the other, it could be tough to balance. This is 6, you can add one more, upright to achieve a 7th:
I believe 8 may be impossible.
Note: The matchstick version, and images, can be found at BrainDen.
I did not use it for the first solution, I found that myself (really proud) but it's where the second answer came from.
answered Oct 25, 2014 at 14:56
-
$\begingroup$ What surprises me so much is I achieved the first one all on my own, I'm smarter then I thought. My train of thought was "Alright, Maybe I can make a ladder of sorts" But that didn't work out properly. Then I thought "maybe there's a trick to this... What if I used 3 dimensional space... I can use 2 layers!" Then it all fell into place. $\endgroup$ Commented Oct 26, 2014 at 14:43
$\begingroup$
$\endgroup$
5
The solution for 7 pencils - without using the ends - was only recently discovered:
http://arxiv.org/abs/1308.5164
Seven mutually touching infinite cylinders - Sándor Bozóki, Tsung-Lin Lee, Lajos Rónyai. It was presented at "Gathering 4 Gardner" in May 2014, because the original 7-cigarette version, allowing the ends to be used, was popularised by a Martin Gardner column in Scientific American 50 years ago.
-
1$\begingroup$ Wow. I didn't expect it to be possible, but it is. I edited your answer by adding the picture from the quoted paper, which clarifies the post even more. $\endgroup$ Commented Oct 25, 2014 at 20:38
-
1$\begingroup$ I cannot remember the pattern while @warspyking's one instantly stuck into my head. Also it is symmetrical: hence it is the elegant solution. $\endgroup$ Commented Oct 26, 2014 at 8:55
-
$\begingroup$ @warspyking: your solution does need the ends. If, for example, your yellow pencil doesn't end where it does (but goes on infinitely), it has to pass under or over the red pencil. If it passes over the red pencil yellow doesn't touch blue anymore. If it passes under the red one, red doesn't touch blue anymore. $\endgroup$ Commented Oct 27, 2014 at 8:01
-
$\begingroup$ And yes, this answer is an elegant solution, but the question was about finite "cylinders", not infinite ones. And for finite cylinders, I think warspyking's solution is even more elegant because it can actually be demonstrated easily in reality :) $\endgroup$ Commented Oct 27, 2014 at 8:03
-
1$\begingroup$ @oerk I didn't realize what was meant by ends... $\endgroup$ Commented Oct 27, 2014 at 17:50
$\begingroup$
$\endgroup$
9
My 7 year old daughter came up with this. I can't find anything wrong with it...
-
3$\begingroup$ They don't touch exactly. There would be a bit of space between the two pencils going diagonally like this [/] and the far right pencil that's almost vertical. $\endgroup$– Deusovi ♦Commented Aug 13, 2015 at 7:32
-
4$\begingroup$ @ChaslyfromUK: Actually, yes. I can prove my objection. For two 'bundles' of two pencils to fully touch, they must be on parallel planes. But if all three planes are parallel, the two not in the middle must not be touching because they're too far apart. $\endgroup$– Deusovi ♦Commented Aug 13, 2015 at 15:17
-
2$\begingroup$ @chaslyfromUK By that I just mean that all four pencils in two bundles are touching all the others. $\endgroup$– Deusovi ♦Commented Aug 13, 2015 at 15:33
-
3$\begingroup$ @chaslyfromUK Sorry for being unclear - a bundle is just two pencils next to each other. Two parallel lines define a plane, so each "bundle" has its own plane defined by the centers (where the lead is in the pencils). Because the pencils are touching, the lead between two touching bundles has a consistent vertical distance, meaning the planes defined by touching bundles are parallel. The three planes are parallel and a fixed distance apart (because the pencil widths are constant), so the outer bundles cannot touch. $\endgroup$– Deusovi ♦Commented Aug 13, 2015 at 18:44
-
4$\begingroup$ @chaslyfromUK: If the pairs aren't parallel, then they cannot touch each other. I'm not assuming that they're placed on a surface at all. If one of the pencils up there is not parallel to the one next to it, then it can't be touching the other ones because it'd be above or below them! And if two of the planes defined by the pairs of pencils are not parallel, then we have the same problem that I originally mentioned. $\endgroup$– Deusovi ♦Commented Aug 15, 2015 at 4:20
$\begingroup$
$\endgroup$
1
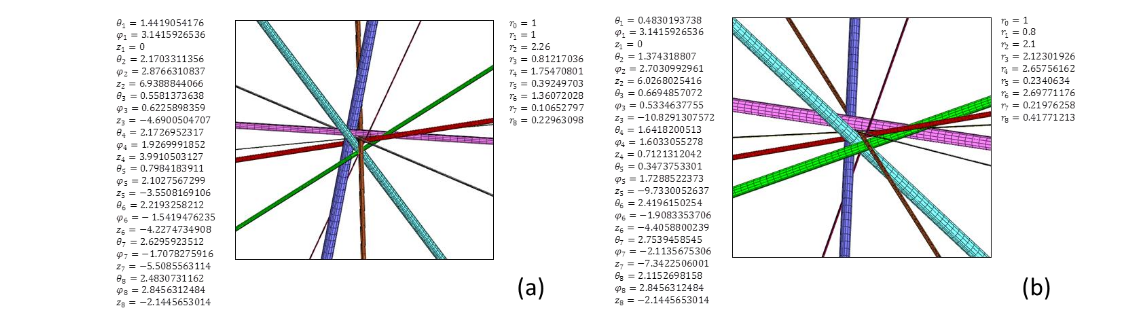
If the pencils are cylinders, and do not have to have equal radii, nine mutually touching cylingers is possible. See page 15 of this paper.
-
$\begingroup$ It's very late, but could you provide an image or something so that this isn't just a link-only answer? $\endgroup$ Commented Oct 21, 2017 at 21:44