What fraction of the larger semicircle is filled? The two smaller semicircles are of equal size.
This is a puzzle originally set by Catriona Agg, who is a puzzle setting genius.
What fraction of the larger semicircle is filled? The two smaller semicircles are of equal size.
This is a puzzle originally set by Catriona Agg, who is a puzzle setting genius.
The fraction is
$\frac{2}{3}$
Without loss of generality let the radius of the smaller circle equal 1.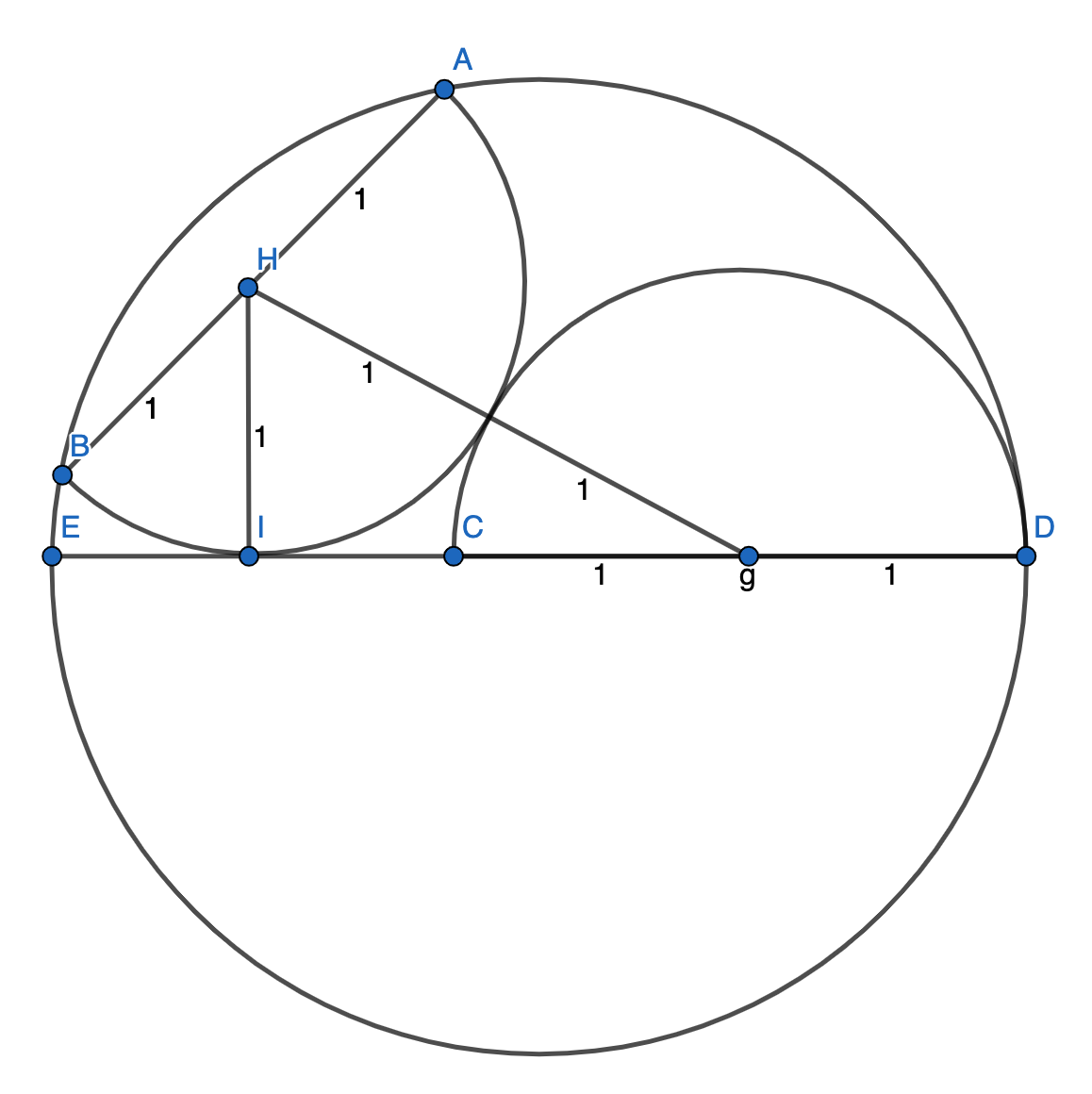
Edit:
Bubbler has observed in a comment that from here it is faster to continue like this
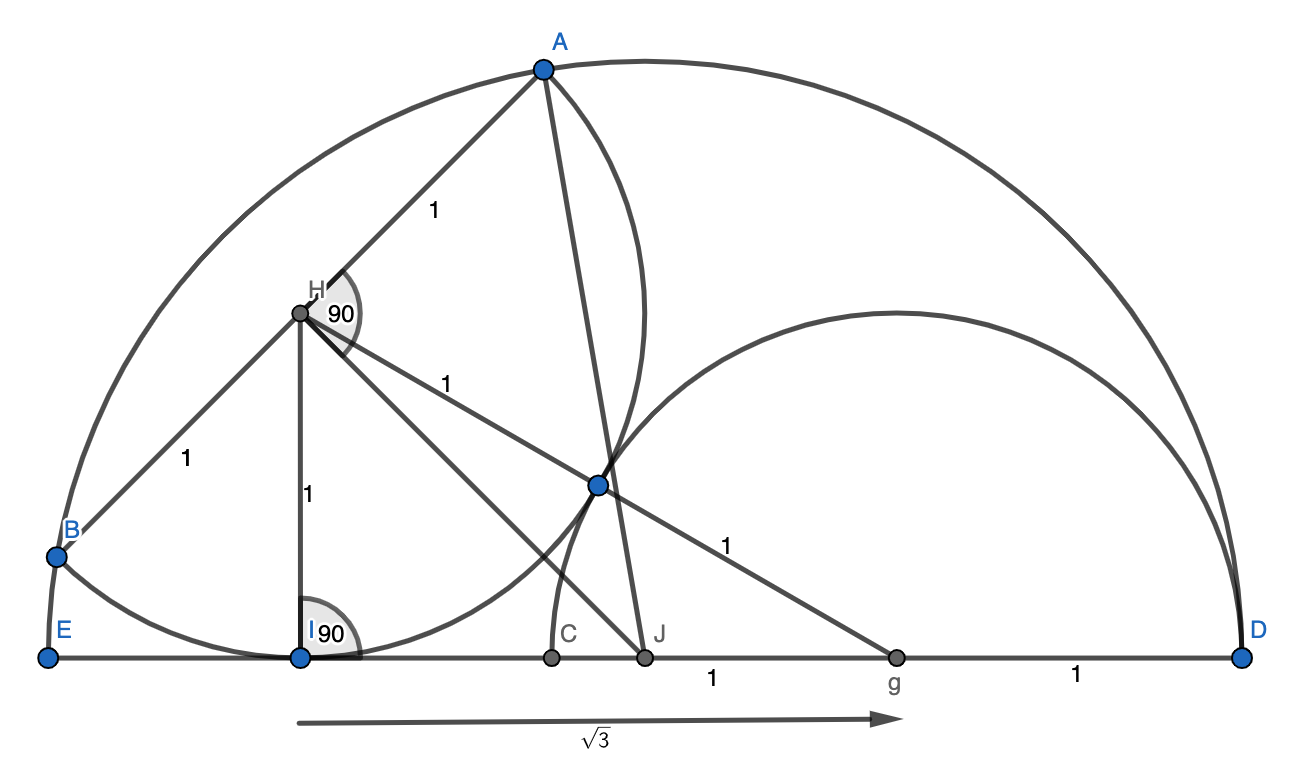
We have $JA=JD=r$, and since JH bisects the chord AB, $\angle{JHA}=90^\circ$.
We also have
$$JA^2=HA^2+(HI^2+IJ^2)$$ $$r^2=1^2+(1^2+(1+\sqrt{3}-r)^2)$$ Solving for r, $$r^2=1^2+1^2+r^2-2 \sqrt{3} r-2 r+2 \sqrt{3}+4$$ $$r=\frac{6+2\sqrt{3}}{2+2\sqrt{3}}=\sqrt{3}$$
Since $\angle HIG=90^\circ$ and $\arcsin(\frac{1}{2})=30^\circ$, we have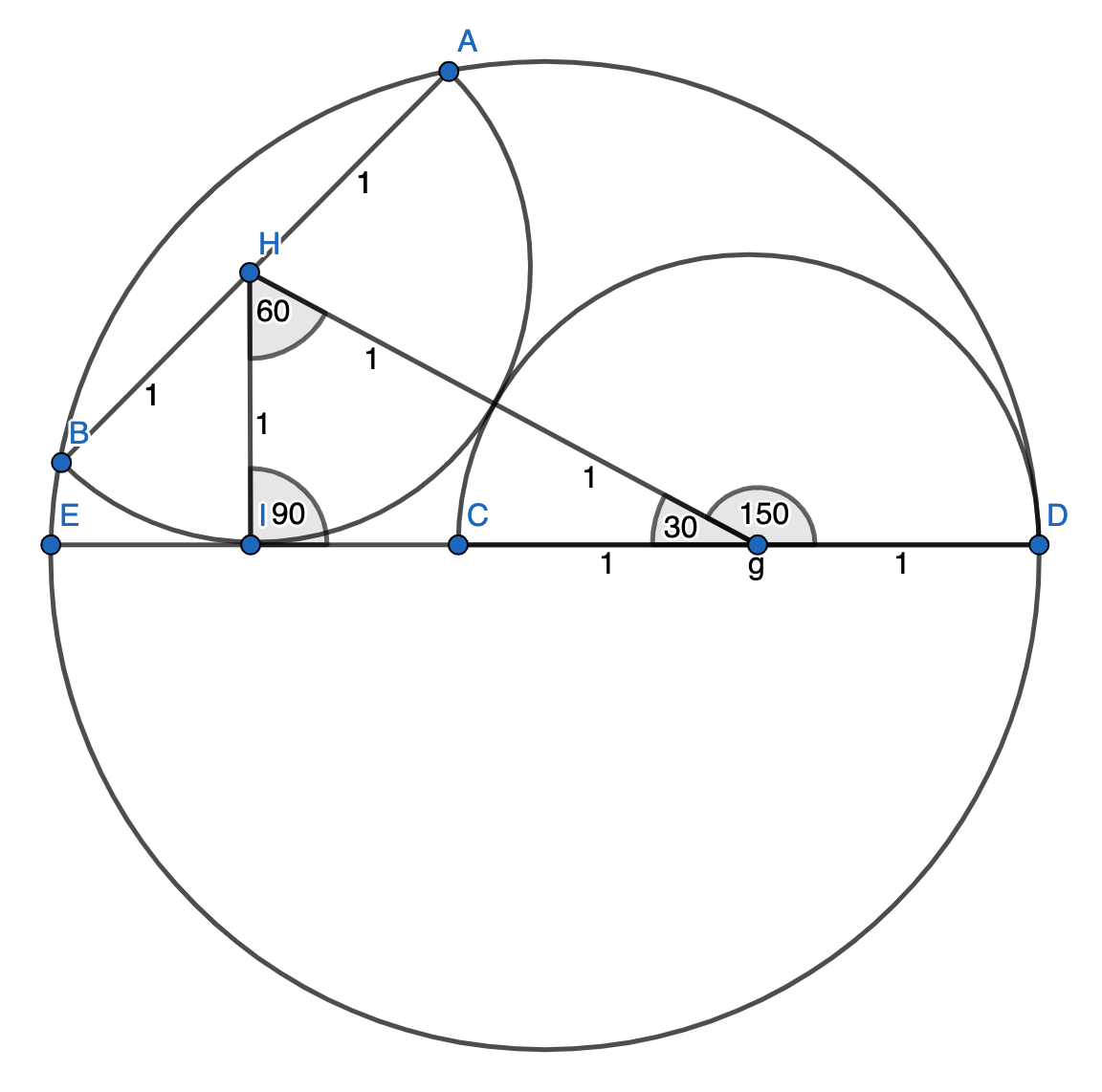
By the pythagorean theorem, ${HD}^2=HI^2+ID^2=1^2+(1+\sqrt{3})^2=5+2 \sqrt{3}$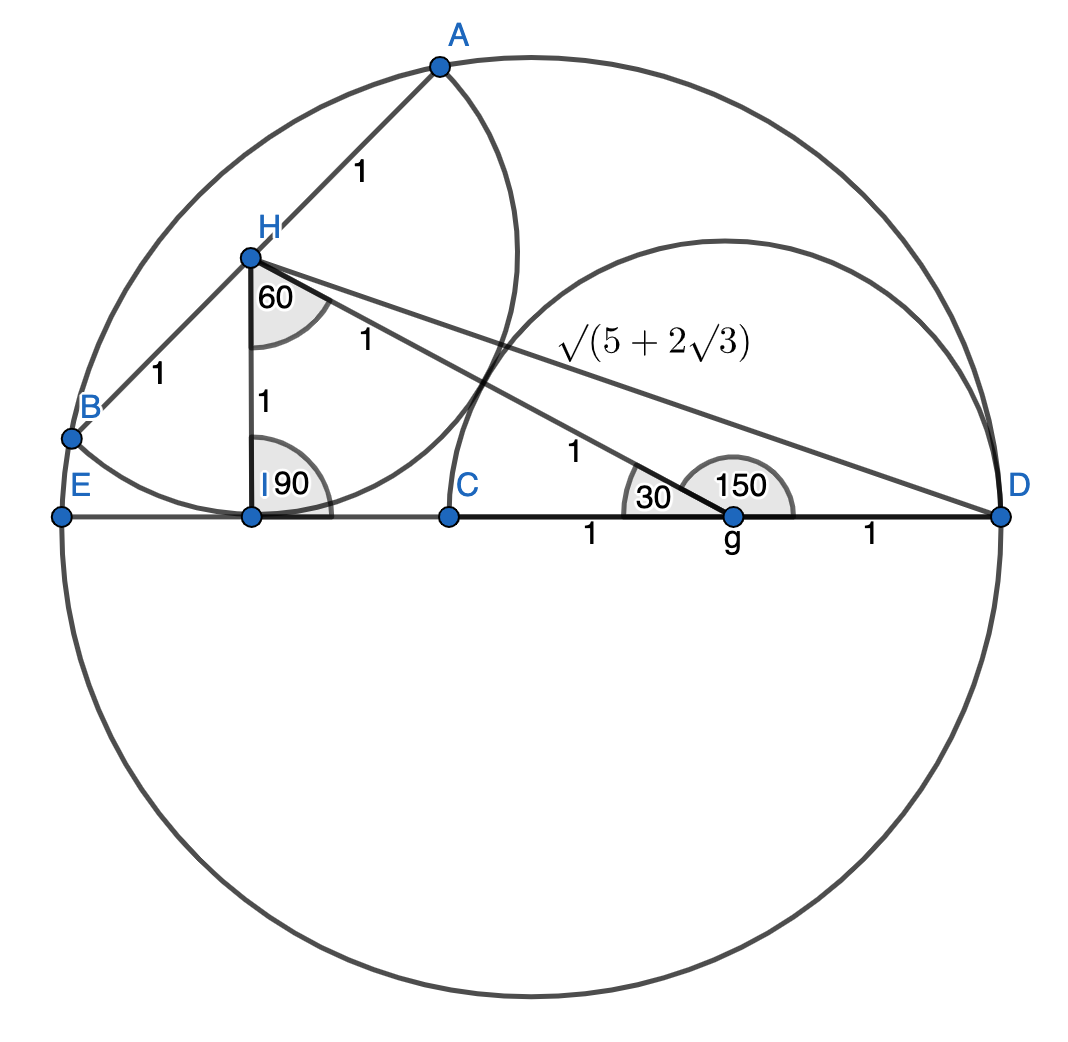
Now label the centre of the large circle J. We have $JA=JD=r$, and since JH bisects the chord AB, $\angle JHA=90^\circ $.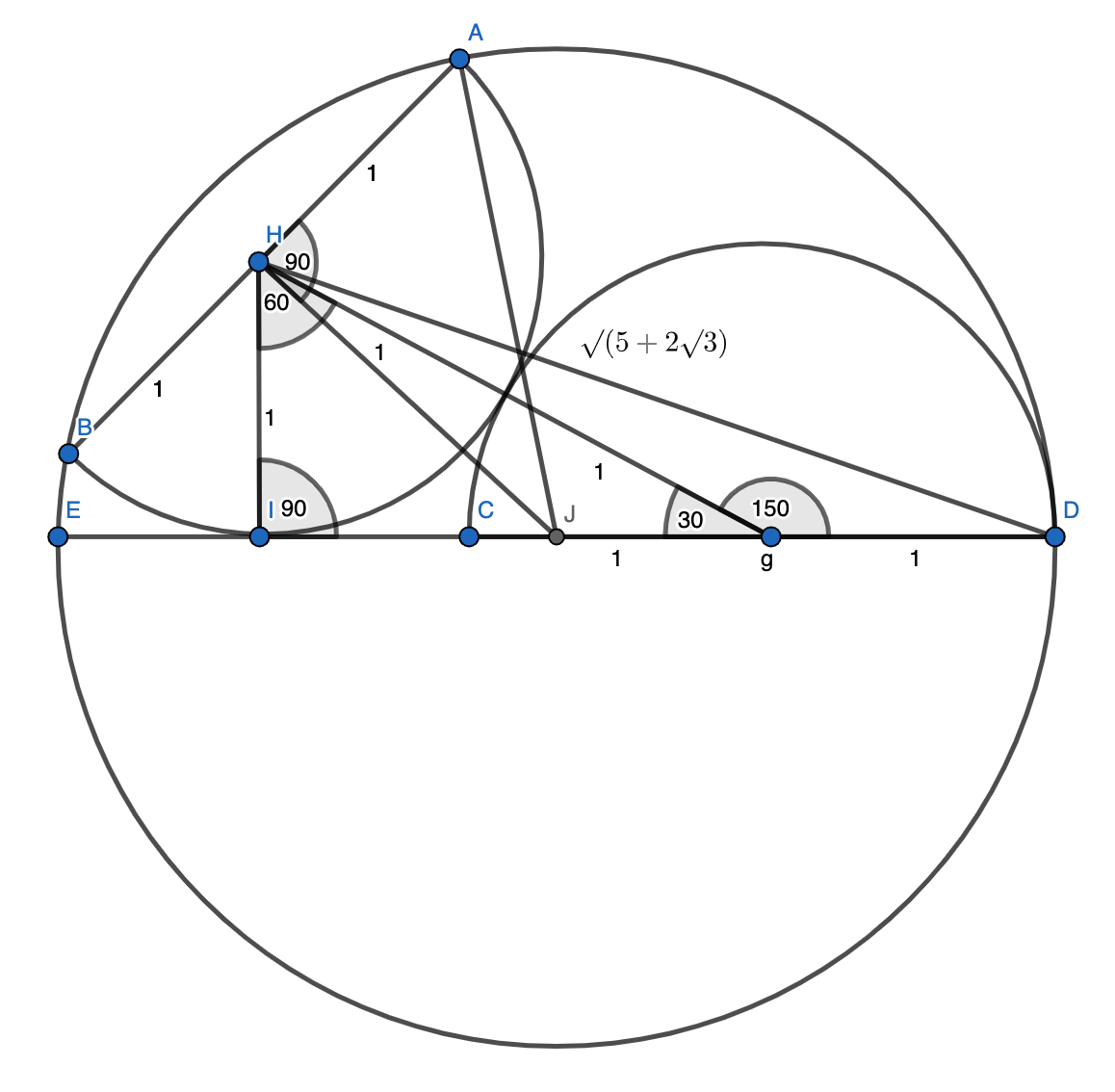 By the pythagorean theorem $$JH^2+1^2=r^2$$
By the pythagorean theorem $$JH^2+1^2=r^2$$
And by the cosine rule $$JH^2=JD^2+HD^2-2\cdot HD\cdot JD\cdot\cos(IDH)$$ $$JH^2=(r^2)+(5+2\sqrt{3})-2\cdot r\cdot \sqrt{5+2\sqrt{3}} \cos(IDH)$$ Combining these 2 equations, $$JH^2=r^2-1^2=(r^2)+(5+2\sqrt{3})-2\cdot r\cdot \sqrt{5+2\sqrt{3}} \cos(IDH)$$ Solving for r produces $$r=\frac{6+2\sqrt{3}}{2\sqrt{5+2\sqrt{3}}\cdot \cos(IDH)}$$ We can use the pythagorean theorem to show that $\cos(IDH)=\frac{\sqrt{4+2\sqrt{3}}}{\sqrt{5+2\sqrt{3}}}$ which now gives $$r=\frac{6+2\sqrt{3}}{2\sqrt{5+2\sqrt{3}}\cdot \frac{\sqrt{4+2\sqrt{3}}}{\sqrt{5+2\sqrt{3}}}}=\frac{6+2\sqrt{3}}{2\sqrt{4+2\sqrt{3}}}$$ Squaring both sides,
$$r^2=\left(\frac{6+2\sqrt{3}}{2\sqrt{4+2\sqrt{3}}}\right)^2=\frac{48+24\sqrt{3}}{4(4+2\sqrt{3})}=3$$ $$r^2=3$$ $$r=\sqrt{3}$$
And the ratio of the areas is
$$\frac{\ \frac{2\cdot \pi \cdot (1^2)}{2}\ }{\ \frac{\pi \cdot (\sqrt{3})^2}{2}\ }=\frac{2}{3}$$
UPDATE: Added more formal proof, see bottom
Here is a less rigorous but maybe more intuitive answer:
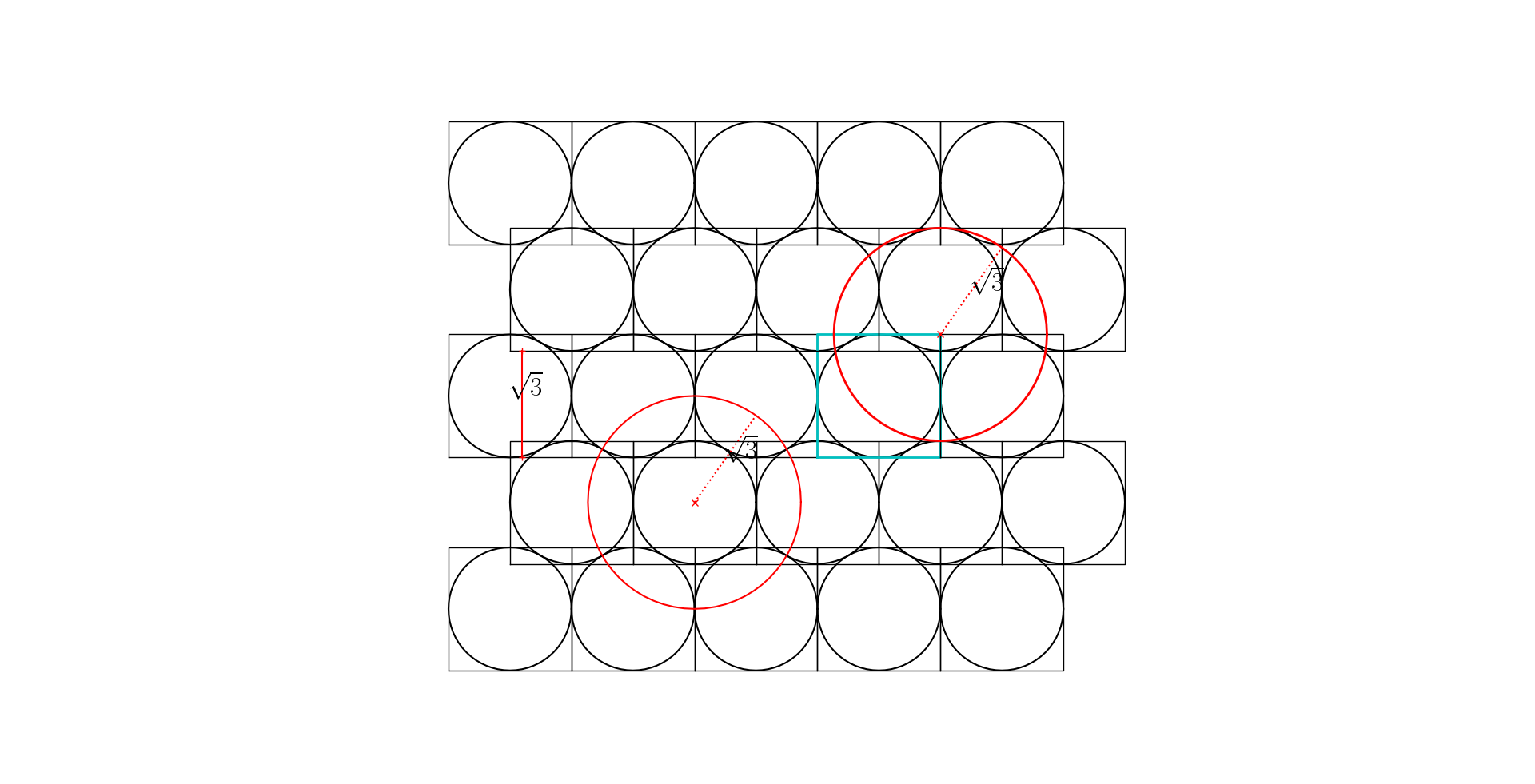
First of all it is easy to see that the small half circles are compatible with a hexagonal packing. Indeed, we can start by taking the mirror image of the entire set wrt its base line to obtain a triangle of touching circles. So we have the familiar grid with units 1 and $\sqrt 3$. If we insert a circle with radius the larger grid unit and we center it at a small circle center then it is easy to parse the picture.
The puzzle is constructed around an "off center" copy of that circle (fat red circle). What one needs to grasp is that by aligning a side of the circle with the corresponding side of a small circle (top in the visualization) we will also have the center on a corner of a grid square (teal square). 45° symmetry as regards intersections with the small circle in the teal cell follows immediately and from there it is a straight-forward calculation to confirm that the large circle cuts the small one at opposite points.
More formal proof:
All in units of the smaller radius. We start by mirroring. This yields the regular triangle DD'X with height $CX=\sqrt 3$ or, equivalently, the 30-60-90 triangle DCX. We can shift this to the right by one unit aligning former circle centers D and X with right ends of circumferences Ds and Xs. We observe that CsDsDC is a unit square. Now we define YZ as the diameter of the unit circle around D that is perpendicular to DCs. Pythagoras on triangle DYCs gives $YCs=\sqrt 3$ and similar for $ZCs$, in other words Y,Z,Xs lie on a circle around Cs.
Here's something resembling a solution using mostly just image editing magic and Pythagoras:
We can continue the pattern to the left by adding a copy of the image to itself, mirrored over the vertical line crossing the centre of the obliquely halved circle.
Here's the result of that operation:
Adding up the parts gives the diameter of the bigger circle in small circle radius units:
$ 1 + \sqrt{3} + \sqrt3 - 1 = 2\sqrt3$
Which gives us the ratio of radiuses
$\frac{1}{\sqrt3}$
Which we can square to get the ratio of areas
$\frac{1}{3}$
And as long as we remember that there are two of the smaller semicircles in the picture, we get the final result:
the covered area is two thirds of the larger semicircle.
Yes, there might conceivably be a gap or some overlap between the seemingly tangent circles in the picture. <handwaving type="energetic">If there were, the answer would be something else, which wouldn't fit the known distances between optimally packed circles. </handwaving>
This answer is a bit of a sanity-check for the other answers posted.
Note: Depending on how you view the image, its dimensions might be different than my measurements here, but the individual dimensions here don't matter; rather, it's the ratio between them that matter.
To find the ratio of the area of the small semicircles to the large semicircle: $$\text{ratio} = \frac{\text{Area of the small semicircles}}{\text{Area of the large semicircle}}$$ ...we really need to know only two things: (1), the diameter of the small semicircles, and (2), the diameter of the large semicircle.
After measuring it with a ruler (yes, that's right), I found the diameter of the small semicircles to be $3$ inches, whilst the diameter of the large one is $5.25$ inches:
We need to compute their respective areas, and to do that, we need to know their respective radii. That's half of their diameters, so the radius of the small semicircles is $\frac{3 \text{in}}{2} = 1.5 \text{in}$ , while the radius of the large is $\frac{5.25\text{in}}{2} = 2.625\text{in}$
The area of a semicircle is just half the area of a full circle with the same radius, ($A = \frac{r^2\pi}{2}$), so to get the radius of the semicircles, we just compute the area of full circles with the same radius, and halve them.
For the small semicircles: $$\text{Area of the small semicircles} = \frac{1.5\text{in}^2\pi}{2} × 2$$ ($× 2$ since there are two small semicircles)
For the large one: $$\text{Area of the large semicircle} = \frac{2.625\text{in}^2\pi}{2}$$
Thus, $$\frac{\text{Area of the small semicircles}}{\text{Area of the large semicircle}}$$ $$= \dfrac{\dfrac{1.5\text{in}^2\pi}{2} × 2}{\dfrac{2.625\text{in}^2\pi}{2}}$$ $$= \frac{32}{49}. $$
And since
$$\frac{32}{49} \approx 0.653 \approx 0.\overline{6} = \frac{2}{3},$$
...we see that the portion of the large semicircle that is shaded is likely
$$\dfrac{2}{3}$$
Slightly late to the party. This finds $r$ of the larger circle (where the smaller circle is a unit radius) using $FHD$ as a circular segment on that larger circle:
Some generalities, not all of which are used:
$$\begin{align} AB & = BC = BJ = DE = EF = EK = EG = GB = 1 \\ AC & = EB = 2 \end{align}$$
Use Pythagoras to establish relative location of $E$, $B$ and $K$:
$$\begin{align} ∠EKB & = π/2 \\ EB^2 & = EK^2 + BK^2 \\ BK & = √(EB^2 - EK^2) \\ & = √(4 - 1) = √3 \end{align}$$
Cartesian coordinates for all the points identified except $D$, $F$, $L$ as they are more complicated:
$$\begin{align} A & = (2r, 0) \\ B & = (2r-1, 0) \\ C & = (2r-2, 0) \\ E & = (2r-1-√3, 1) \\ G & = (B + E)/2 \\ & = 1/2(2r-1 + 2r-1-√3, 1) \\ & = 1/2(4r-2-√3, 1) \\ & = (2r-1-√3/2, 1/2) \\ H & = (r, 0) \\ J & = (2r-1, 1) \\ K & = (2r-1-√3, 0) \end{align}$$
Establish $EH$ using Pythagoras, in order to get the apothem of the segment:
$$\begin{align} HK & = H - K \\ & = r - (2r-1-√3) \\ & = r - 2r + 1 + √3 \\ & = 1 + √3 - r \\ HK^2 & = (1 + √3 - r)^2 \\ & = (1 + √3)^2 - 2r(1 + √3) + r^2 \\ & = 1 + 2√3 + 3 - 2r(1 + √3) + r^2 \\ & = 4 + 2√3 - 2r(1 + √3) + r^2 \\ EH^2 & = HK^2 + EK^2 \\ & = 4 + 2√3 - 2r(1 + √3) + r^2 + 1 \\ & = 5 + 2√3 - 2r(1 + √3) + r^2 \\ & = r^2 - 2r(1+√3) + 5+2√3 \\ EH = & √(r^2 - 2r(1+√3) + 5+2√3) \end{align}$$
$r$ in terms of $h$ (the sagitta, $EL$) and $c$ (the chord length, $DF$) via the intersecting chord theorem:
$$\begin{align} r & = c^2/8h + h/2 = HL \\ c & = DF = 2 \\ h & = EL = HL - EH \\ & = r - √(r^2 - 2r(1+√3) + 5+2√3) \\ r & = 4/8h + h/2 = 1/2h + h/2 \\ 2hr & = h^2 + 1 \\ h^2 - 2hr + 1 & = 0 \end{align}$$
Expand out $h^2$ and $2hr$ because then it is very clean to complete the equation above:
$$\begin{align} h^2 & = r^2 - 2r√(r^2 - 2r(1+√3) + 5+2√3) + r^2 - 2r(1+√3) + 5+2√3 \\ & = 2r^2 - 2r√(r^2 - 2r(1+√3) + 5+2√3) - 2r(1+√3) + 5+2√3 \\ 2hr & = 2r^2 - 2r√(r^2 - 2r(1+√3) + 5+2√3) \end{align}$$
Then we have:
$$\begin{align} -2r(1+√3) + 5+2√3 + 1 & = 0 \\ -2r(1+√3) + 6+2√3 & = 0 \\ -r(1+√3) + 3+√3 & = 0 \\ r(1+√3) - (3+√3) & = 0 \\ r(1+√3) & = (3+√3) \\ r & = (√3+3)/(1+√3) \\ & = √3(√3+3)/√3(1+√3) \\ & = √3(√3+3)/(√3+3) \\ & = √3 \end{align}$$
Feeding $r$ back into $EH$:
$$\begin{align} r & = √3 \\ EH & = √(r^2 − 2r(1+√3) + 5 + 2√3) \\ & = √(3 − 2√3(1+√3) + 5 + 2√3) \\ & = √(8 − (2√3+6) + 2√3) \\ & = √(8 − 2√3 - 6 + 2√3) \\ & = √(8 - 6) \\ & = √2 \end{align}$$
Since $EK$ is 1, and $∠EKH$ is a right-angle, $KH$ is also 1, and $∠EHK = π/4$.