7
$\begingroup$

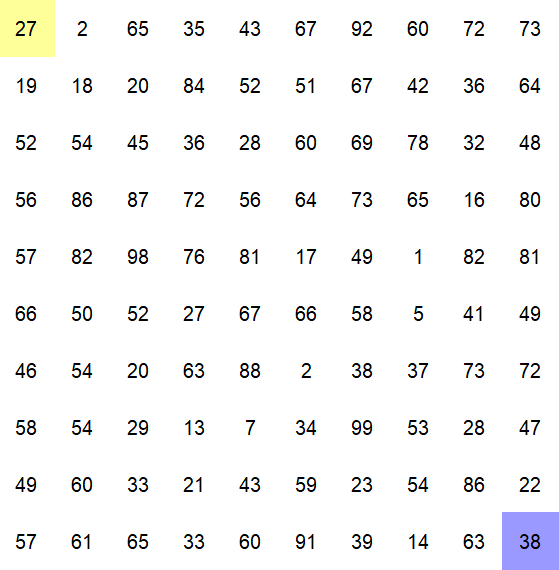
Inspired by Galen's series of puzzles...there is a relation between rectilinear-adjacent squares such that there is a unique rectilinear path from the top-left corner of the grid down to the bottom-right corner of the grid. Each square can participate in the path no more than once. What is the relation and the path it induces?

Puzzle Grid

Improve this question
$\endgroup$
1
  • $\begingroup$ Any comments on my answer? $\endgroup$
    – Culver Kwan
    Commented May 19, 2020 at 5:30

2 Answers 2

Reset to default
4
$\begingroup$

Path(Sorry, but I did on my IPad:

enter image description here

Relation:

Difference adjacent numbers is a power of 2

Improve this answer
$\endgroup$
1
  • $\begingroup$ Perfectly correct! $\endgroup$
    – Jeremy Dover
    Commented May 19, 2020 at 5:32
3
$\begingroup$

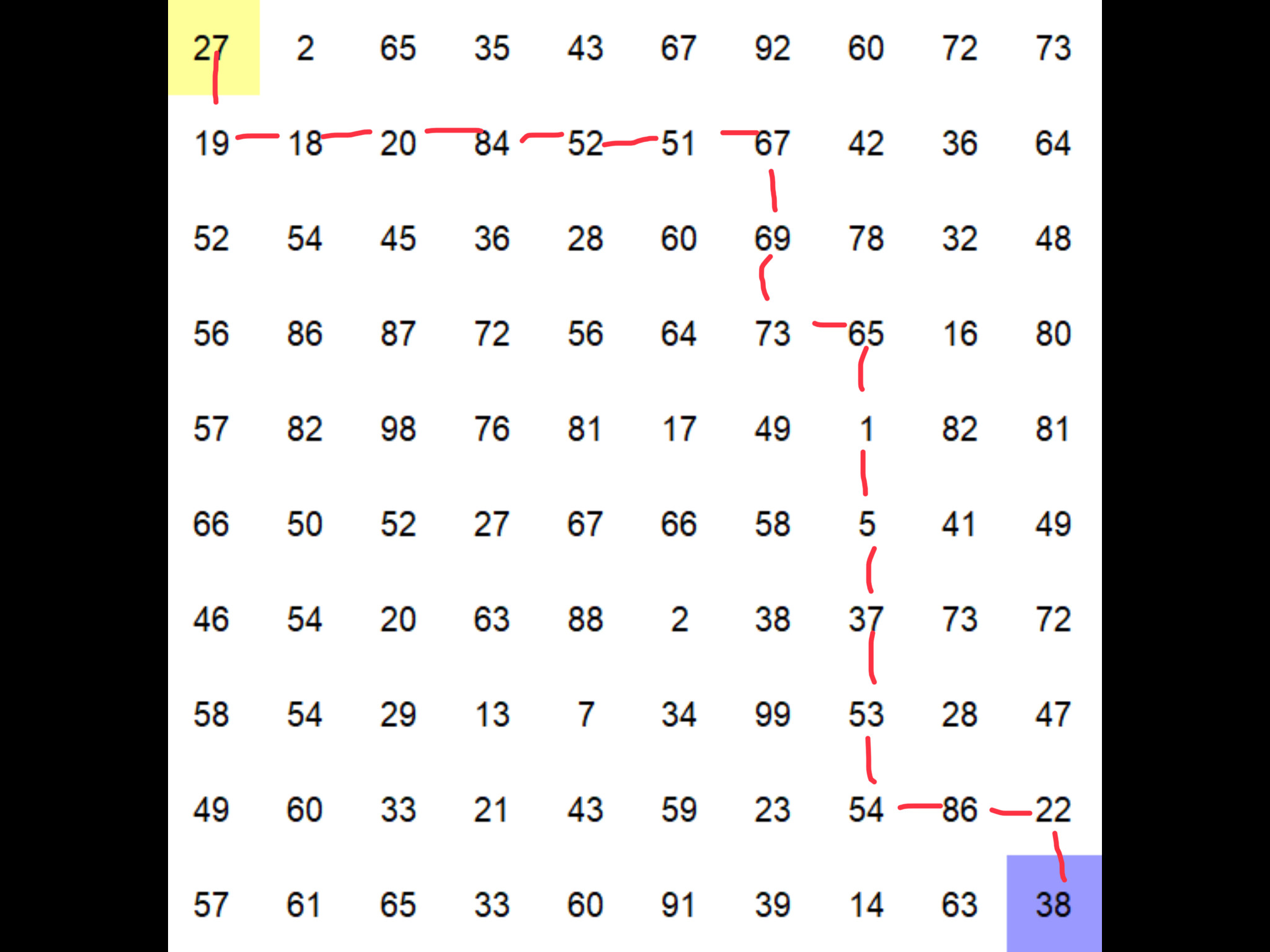
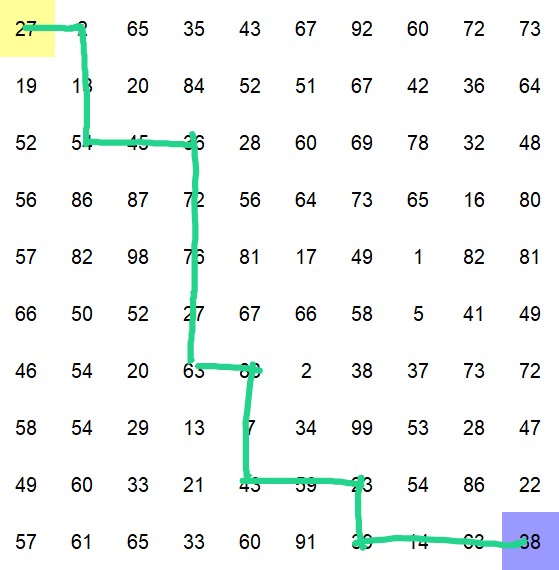
enter image description here
Absolute differences between successive terms are all squares.

Improve this answer
$\endgroup$
5
  • $\begingroup$ yes, this is a path but the relation does not satisfy all of the conditions of the question. $\endgroup$
    – Jeremy Dover
    Commented May 19, 2020 at 1:06
  • $\begingroup$ This path is unique under the given relation; what condition is being violated? $\endgroup$
    – AxiomaticSystem
    Commented May 19, 2020 at 2:41
  • 1
    $\begingroup$ I think you need to check that again. The path is not unique under the given relation. $\endgroup$
    – Jeremy Dover
    Commented May 19, 2020 at 2:50
  • $\begingroup$ Agh, you're right. There's two paths, and rot13(obgu pbagnva ercrngf fb V'z abg fher juvpu bar vf gur pbeerpg bar, vs rvgure vf.) $\endgroup$
    – AxiomaticSystem
    Commented May 19, 2020 at 3:06
  • $\begingroup$ Neither can be correct, since the relation does not produce a unique path. Don't feel bad though, I did my best to bait exactly this trap :-) $\endgroup$
    – Jeremy Dover
    Commented May 19, 2020 at 5:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.