3
$\begingroup$

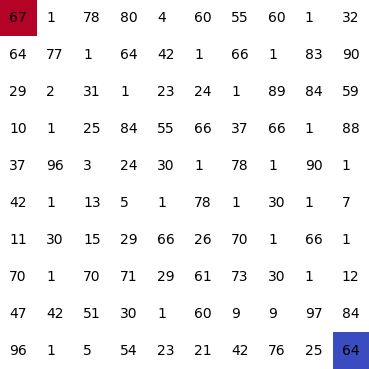
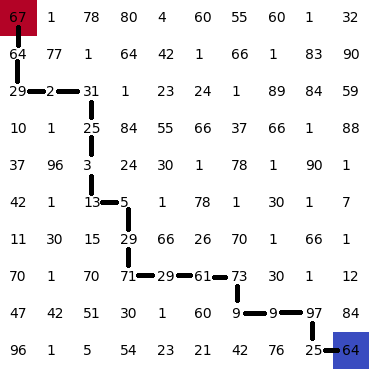
There is a relation between rectilinear-adjacent squares such that there is a unique rectilinear path from the top-left corner of the grid down to the bottom-right corner of the grid. Each square can participate in the path no more than once. What is the relation and the path it induces?

enter image description here

Previous

Improve this question
$\endgroup$

1 Answer 1

Reset to default
4
$\begingroup$

The path is as follows

Solution image

And the relationship is

Numbers in path are primes or prime powers. Put another way, numbers in adjacent squares both have the same number (1) of distinct prime divisors.

Improve this answer
$\endgroup$
3
  • $\begingroup$ rot13(Gur vagraqrq eryngvba jnf gung gjb nqwnprag fdhnerf unq gur fnzr aba-qrtrarengr pbhag bs cevzr snpgbef, ohg lbhe eryngvba vf n fbyhgvba sbe guvf tevq!) Congratulations! $\endgroup$
    – Galen
    Commented May 14, 2020 at 2:10
  • $\begingroup$ rot13(V'z purevgnoyl nffhzvat lbh zrnag gung nqwnprag fdhnerf ubyq gur eryngvba bs obgu univat gur nsberzragvbarq cebcregl, ohg na rqvg jbhyq or nccerpvngrq.) $\endgroup$
    – Galen
    Commented May 14, 2020 at 2:12
  • $\begingroup$ That's great, thanks! $\endgroup$
    – Galen
    Commented May 14, 2020 at 2:26

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.