This is a puzzle for logical minds. Please explain me the answer, with steps.
This puzzle can be found at:
https://puzzlersworld.com/number-puzzles/solve-this-puzzle/
This is a puzzle for logical minds. Please explain me the answer, with steps.
This puzzle can be found at:
https://puzzlersworld.com/number-puzzles/solve-this-puzzle/

Answer will be :
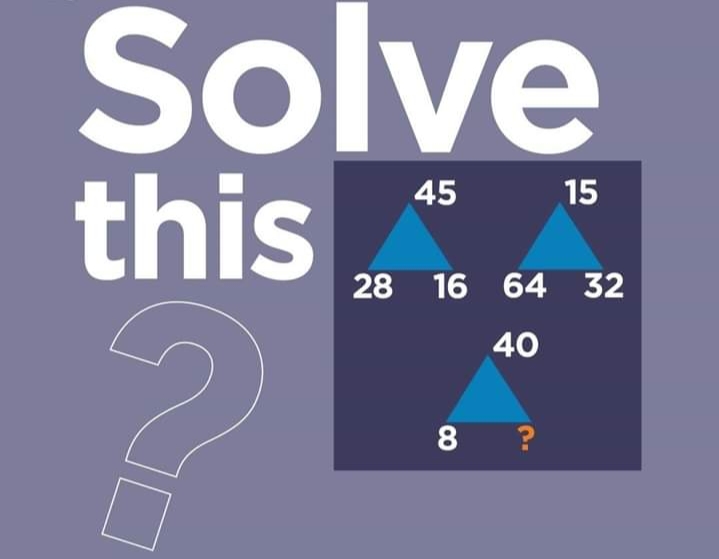
$52$
Because
If you total every number i.e: all number in top in all triangles will give you 100 , same for left numbers and right numbers . i.e:
For top numbers in all triangle : 45+15+40 = $100$
For all number in left of triangle : 28+64+8 =$100$
For all numbers in right of triangle : 16 + 32 + x(third triangle value be x) = 100 => x = 100-48 => x = $52$
The most common problem with numeric puzzles is the absence of definition. Do we search for a trick, a logical explanation or a combination of the two?
For example,
If we assume that the 1st triangle is the left top, the 2nd the right top and the 3rd the bottom, then the numbers increase clockwise, but the relationship changes anti clockwise i.e. we have the following vectors
$<16, 28, 45>, <15, 32, 64>, <8, 40, x>$
Also, a common trick in numeric puzzles is the treatment of numbers as digits and use some or all of them, which transforms the problem more or less to $<\{1,6\}, \{2,8\}, \{4,5\}>, <\{1,5\}, \{3,2\}, \{6,4 \}>, <\{0,8\}, \{4,0\}, x>$
After all of those assumptions, a possible solution is:
$<\{a,b\}, \{c,d\}, \{c\times 2,b-1\}> \space \Rightarrow \space <\{0,8\}, \{4,0\}, \{8,7\}>$
or simply $87$
Around the first triangle, $16*45 = 720$. $(28-1)^2 = 729$.
Second : $15*64 = 960$. $(32-1)^2 = 961$
We can assume the numbers from the remaining edge of the triangle will be multiplied, namely the bottom one. $8x$ must be barely less than $39^2=1521$ (be the largest number giving a smaller result). Assuming this is the intended logic, the answer is $190$.