Given a square piece of paper. Cut it into 4 pieces that could be used to create a right angle pyramid - the 4 pieces are the faces of the pyramid.
$\begingroup$
$\endgroup$
7
-
$\begingroup$ What's the definition of a right angle pyramid? $\endgroup$– hexominoCommented Jul 12, 2019 at 8:52
-
$\begingroup$ I assume the question means a right pyramid, rather than right angled. A right pyramid has the apex directly above the centre of the base. $\endgroup$– ShowsniCommented Jul 12, 2019 at 12:04
-
$\begingroup$ No. Use this definition: " A right-angled pyramid has its apex above an edge or vertex of the base. In a tetrahedron these qualifiers change based on which face is considered the base" and the requirement is - apes above a vertex. $\endgroup$– MotiCommented Jul 12, 2019 at 15:00
-
$\begingroup$ @Moti The pyramid in our solutions is a right-angled pyramid then. If you consider any of the right-angled triangles as the base, the apex will lie above one of the vertices of the base. $\endgroup$– hexominoCommented Jul 12, 2019 at 16:21
-
$\begingroup$ I am seeking a solution where three faces are perpendicular to each other - three edges are along the cartesian axes and 4 faces (I think your solution contains a face that is not a piece of the cut) $\endgroup$– MotiCommented Jul 12, 2019 at 16:33
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
5
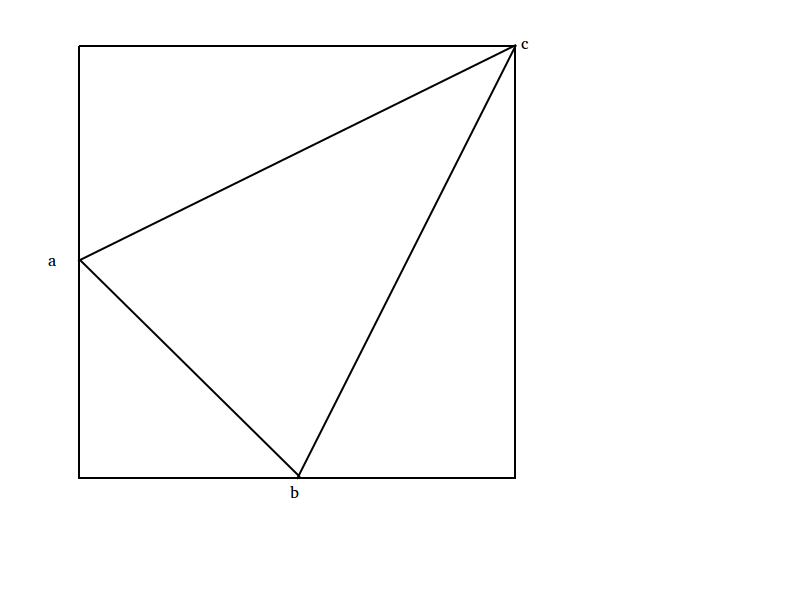
I think you could make cuts as follows
so that $a$ and $b$ are the midpoints of the sides of the square.
Then, the three triangles surrounding the central triangle can be folded up along their adjoining edges to create a pyramid.
answered Jul 11, 2019 at 14:33
-
$\begingroup$ I edited the puzzle to reflect the requirement for a right angle pyramid. Hope I am not disappointing you since you have a nice solution. $\endgroup$– MotiCommented Jul 12, 2019 at 6:54
-
$\begingroup$ I think your solution uses an additional face that is not part of the cuts. $\endgroup$– MotiCommented Jul 12, 2019 at 16:34
-
$\begingroup$ @Moti There are four triangles in the picture. Those are the four faces, why would I need another one? $\endgroup$– hexominoCommented Jul 12, 2019 at 16:37
-
$\begingroup$ @Moti, if we use the small triangle as the base, we could label the coordinates of this pyramid in three-space as (0,0,0), (1,0,0), (0,1,0) and (0,0,2). This is exactly what you've described in your comment. $\endgroup$– hexominoCommented Jul 12, 2019 at 16:40
-
1$\begingroup$ You are right and I am accepting your solution $\endgroup$– MotiCommented Jul 12, 2019 at 17:15
$\begingroup$
$\endgroup$
4
How about this cut? (With Region A as the base of the pyramid)
answered Jul 11, 2019 at 14:37
-
$\begingroup$ Seems to be exactly the same as the answer that preceded yours by a few minutes. $\endgroup$– msh210Commented Jul 11, 2019 at 14:56
-
$\begingroup$ It is, I was putting together the diagram and as I was posting I was notified the other solution was posted. I'm fairly certain this is a unique solution anyway. $\endgroup$ Commented Jul 11, 2019 at 17:48
-
$\begingroup$ There is another solution for a right angle pyramid - see edited puzzle. $\endgroup$– MotiCommented Jul 12, 2019 at 6:52
-
$\begingroup$ See also other comments - your solution is of 5 faces pyramid. $\endgroup$– MotiCommented Jul 12, 2019 at 16:35