Unfold a right angle pyramid into a square

Question

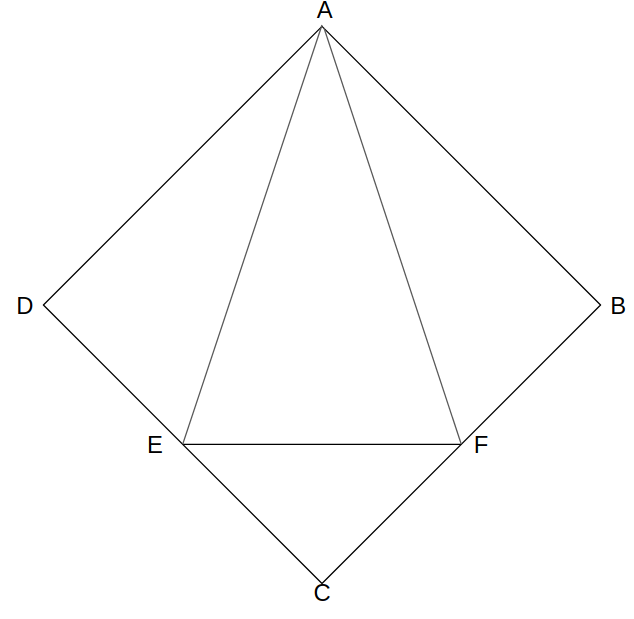
This puzzle refers to a feature of right angle pyramid:

The relation between the areas of the three perpendicular faces and the diagonal surface area is given as - $S^2_x+S^2_y+S^2_z = S^2_d$

Visit the link for details: De Gua's theorem

1. The challenge is to unfold the 3D pyramid surfaces into a 2D shape and than cut it into two pieces to be reassembled into a square. There is one specific case where unfolding will create a square with no need to cut the shape.

2. It is not possible for all pyramids (I think) - what is the condition with regard to the surfaces for this to be solved?

Answer 1

Here's a partial answer, which is for the case where unfolding will create a square with no need to cut the shape:

Proof: ABCD is a square, so we need to show that it can be folded to a right pyramid. Bisect DC at E. Bisect BC at F. Connect AE, AF, and EF. Then fold △AED, △ABF, and △EFC up (or down), so that C, D, and B meet. This can be done because EC=ED, CF=BF, and DA=BA. And clearly angles, D, C, and B are right angles.

Depending on definitions, there is a class of pyramids for which this can be done using the disection technique:

The above shows that any rectangle can be folded into a right pyramid using a similar proof (except the base is a quadrilateral rather than a triangle). Then any rectangle that can be cut and reformed into a square can be done. For example, a 9x16 rectangle can be cut into steps to form a 12x12.