Feb 8th edit:
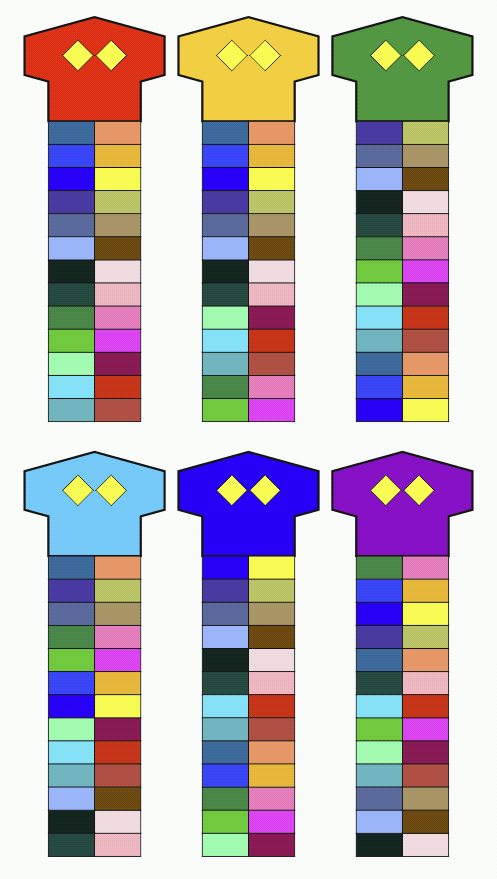
Some more progress has been made since I posted this answer, now I'm choosing to edit it to reflect what we know. I used the keys to finish the scale's color spectrum base; the edited scale has been added by Bmy to the wiki answer with an explanation of the logic involved.
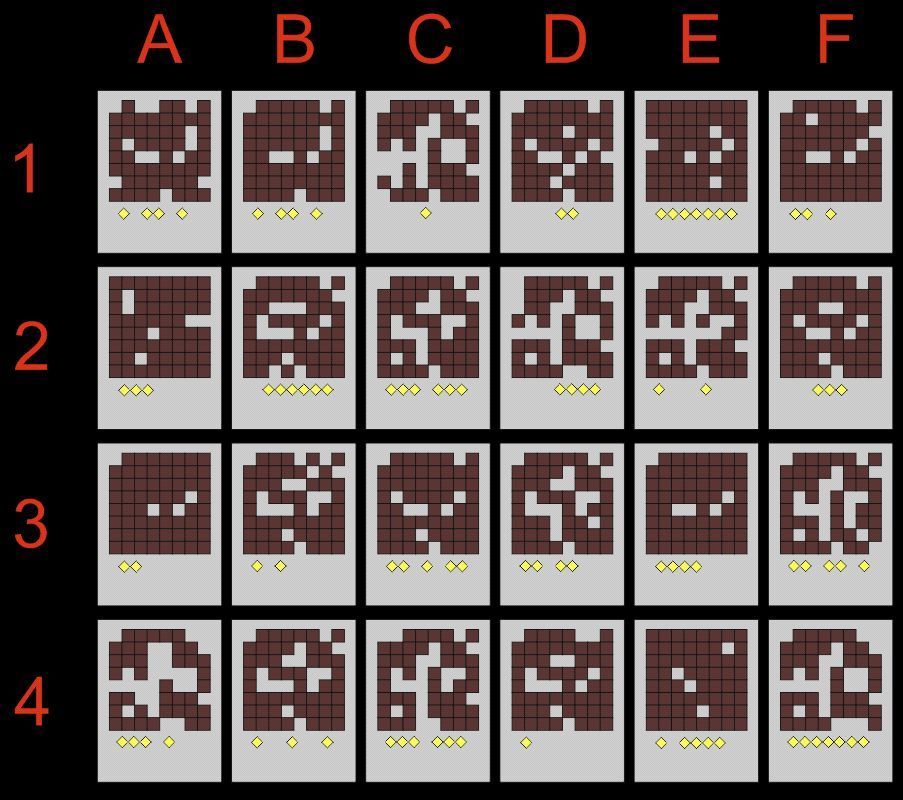
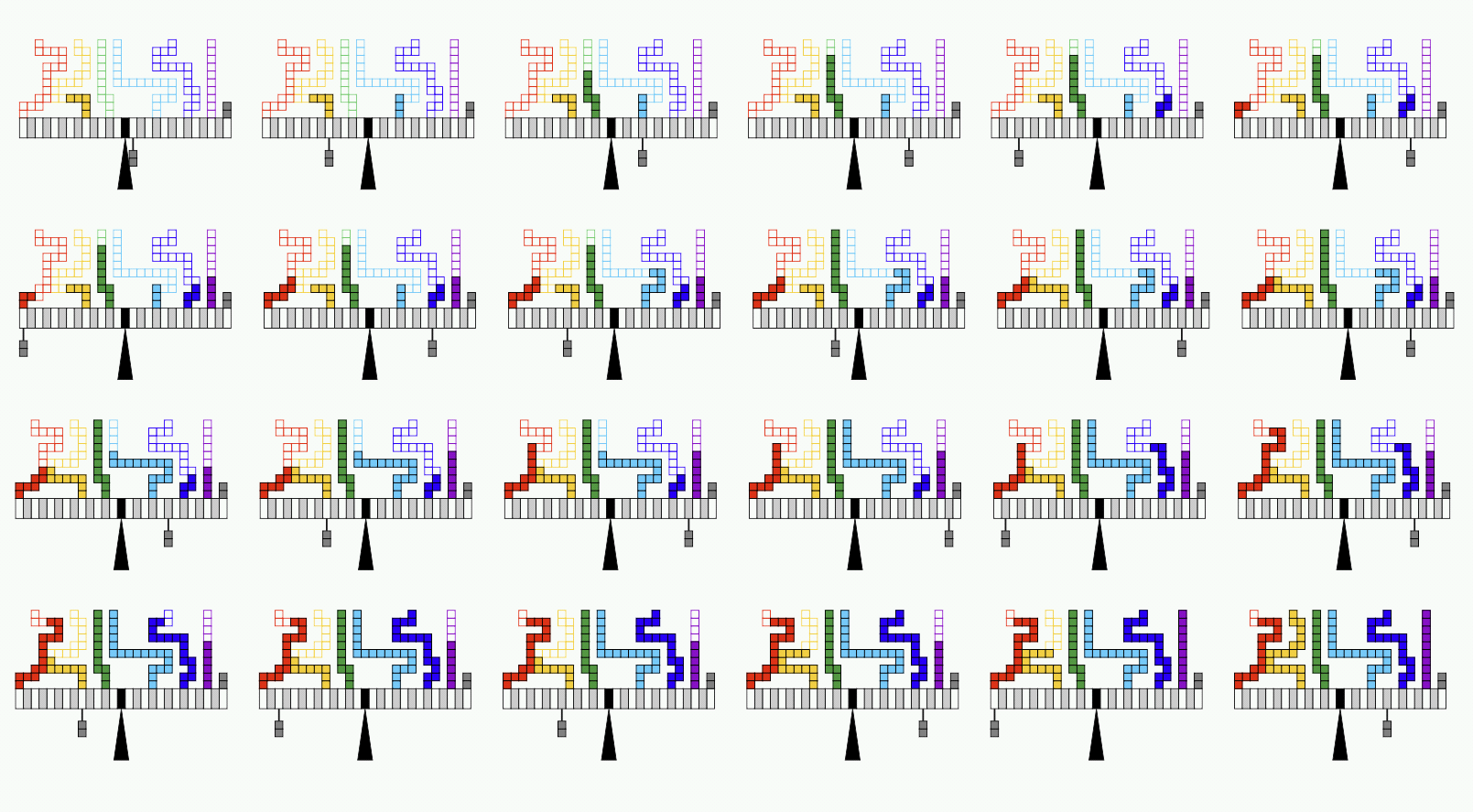
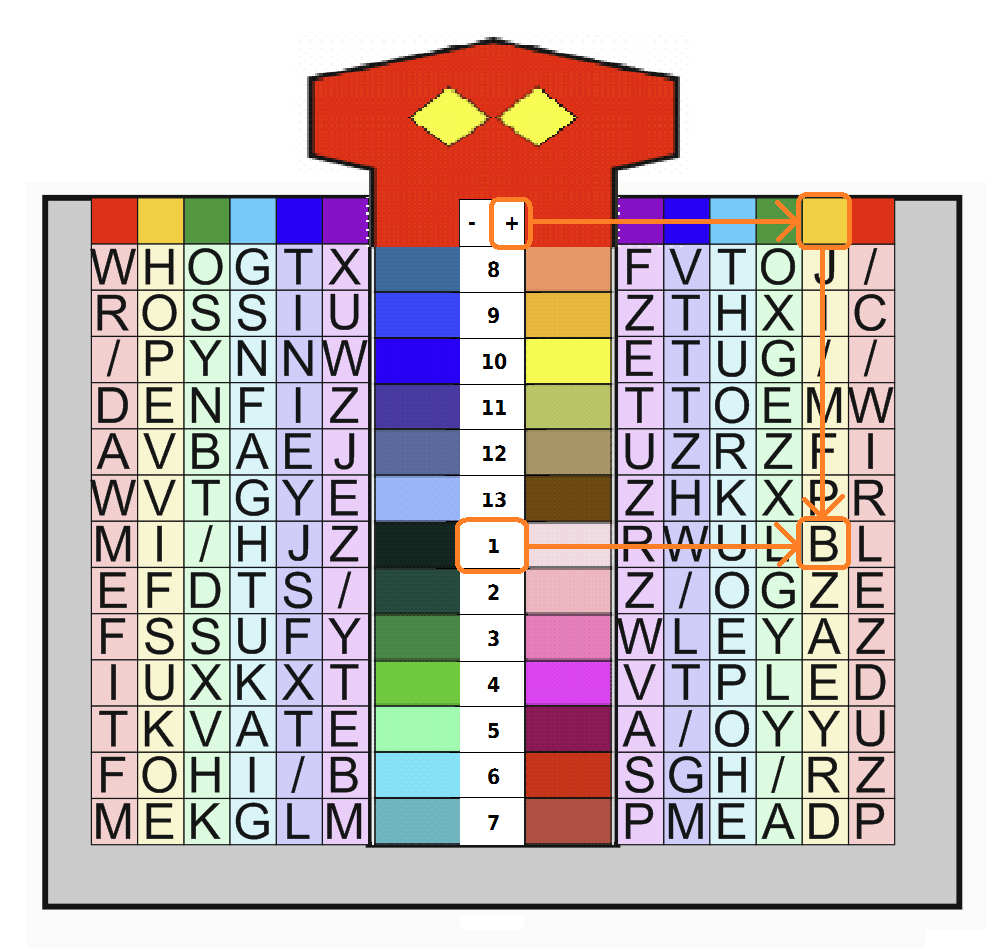
The issue we are facing now is deciding which tubes the bottle contents need to go into. We have supposedly used up the meaning of the 8x8 grid, where it was relevant to determining order. The yellow diamonds are the only label we have not fully understood from the first instruction, and it is believed that this is where we will determine the placement of the contents.
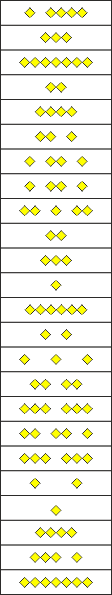
My current line of thinking holds that the yellow diamonds tell us the amount that the respective bottles will put into the tubes. This is supported both by my counting (95 empty tube spaces, 93 diamonds) and by Bmy's upvote. However, if this were all that the diamonds tell us, then we would still have no information on which tubes to fill. The only piece I can imagine being useful is the spacing between the diamonds. It has been made clear that the labels are supposed to be center-aligned, and thus the left/right sides of the diamonds don't mean anything. Only the spacing /between/ them seems to matter here, though it could still be that it has no meaning at all. In such a case, for example, bottle 14 and bottle 20 would be the same with regards to their diamond pattern. The question to be answered, in short, is this: what does the diamond spacing mean?
I did some testing on a selection of ideas. My first idea was that the spacing simply described how many tubes each bottle is divided into. Our first bottle is a 1_4, so this idea would claim that one unit goes in one tube while the other four units go somewhere else. Presumably, this also suggests that the 1 unit will go in a farther left direction than the 4 units, based on their order on the label. This is all well and good, but following it through encountered a problem very early.
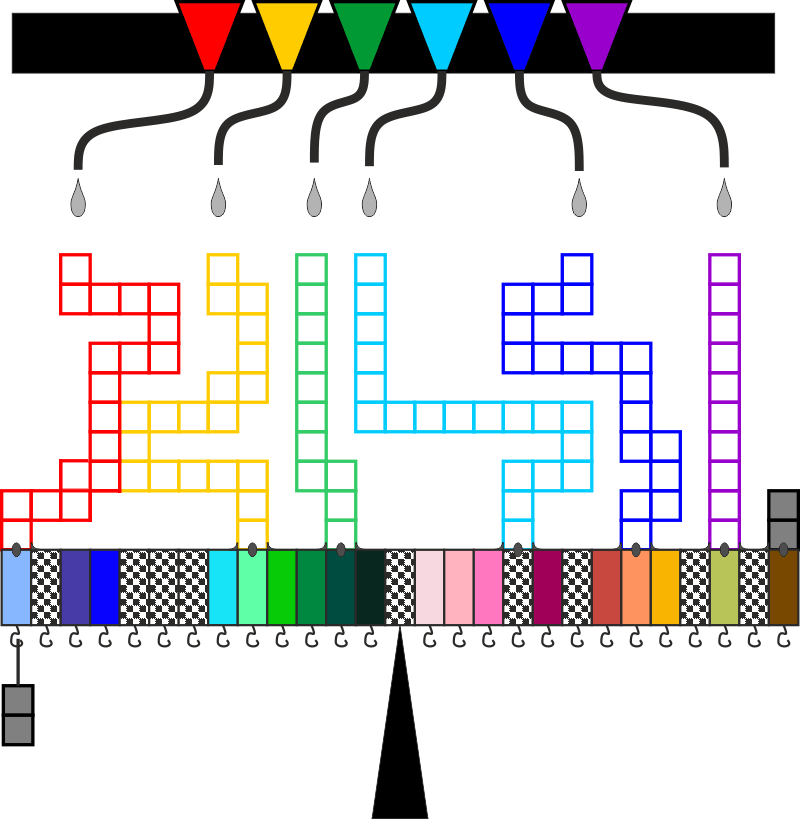
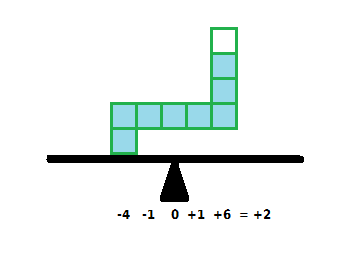
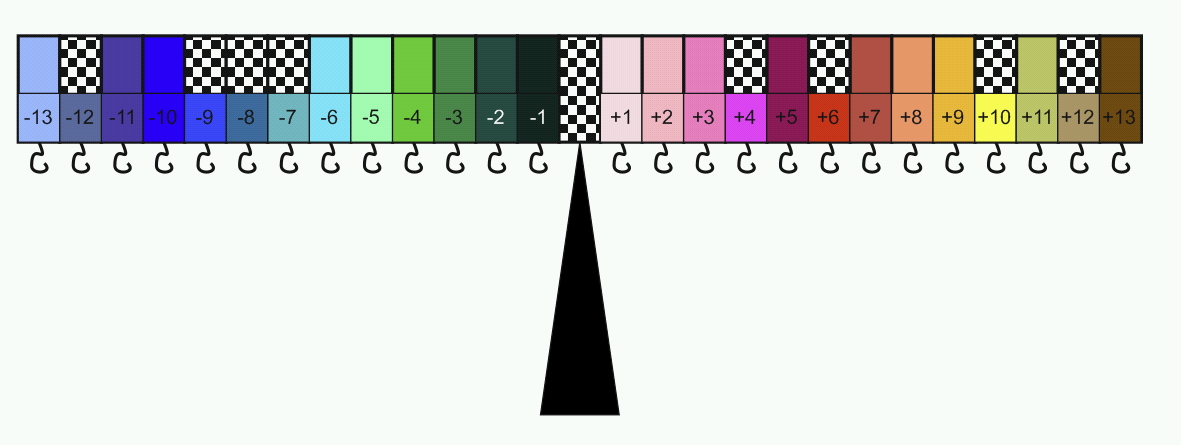
Looking at the scale in more detail, we are supposed to keep it balanced. To make the force calculations and referencing easier, I labeled each hook on the number line -13 to +13. The pipes are placed in specific spots on the scale, specifically at locations
[-13, -5, -2, 4, 8, 11].
This means the force that the bottles apply to the scale will always be in only these 6 locations. The weight can be placed on any hook, which you'd think makes things easier. Unfortunately, the weight is 2 units in size. Therefore, any force the weight provides to the scale will be an even number; an even number multiplied by any number always results in an even number. Therefore, to keep the scale balanced, we must ensure that the forces the filled tubes apply are even amounts as well: to balance an even force on one side requires an equal force placed on the other.
What does this mean?
It means, young padawan, that our bottles that have an odd number of diamonds must fill only tubes standing on even numbers. For example, if we take a 3-diamond bottle and fill the yellow tube at location -5, then that would be -15 units of pressure on the scale. We cannot put the weight anywhere on the scale to perfectly balance 15 units, because the hanging weight cannot provide an odd numbered amount of pressure.
Looking back at the first bottle, our 1_4 bottle, lets assume that we must follow the rules I stated above. The 1 unit must go into an even numbered tube [-2, 4, or 8]. The 4 units should go into any tube to the right of that [4, 8, or 11]. Unfortunately, this is already impossible - putting 4 units into the 4 spot is 16 units of pressure, plus the 26 units of pressure already on the right end = 42 units of pressure. The 1 remaining unit of liquid and the hanging weight can only provide 28 units of pressure combined at maximum, not enough to balance the scale.
That might have been a lot to process, so let's make this easier. We have weight already on the right end. We must put weight on the left end of the scale to balance it. Of course, pouring the first bottle's contents into a tube on the right end would only make things worse. We can't pour the first bottle into the odd-numbered tubes, either, because its force cannot be balanced by our even-numbered hanging weight. By process of elimination, we can only pour the bottle - all 5 units - into the green tube. Things begin to look better: 5 units * -2 distance from the center = -10 units of pressure, + 26 units from the right hand side = +16 units of pressure on the scale. To balance this evenly with an added -16 units of pressure, the weight must be placed on the -8 hook.
You might be thinking, "Great! We have a plan." Unfortunately, this plan doesn't continue very far. Long story short, we can't keep putting odd-numbered diamond bottles into even-numbered tubes, because we will end up favoring the green tube too much and cause it to overflow.
All of this means something is wrong somewhere. Whether it was my analysis of the scale, or my analysis of the diamonds, something is not matching up properly.
My most recent failed idea was ignoring the spaces between the diamonds. I interpreted instruction-2 a bit more literally, and thought maybe the hanging weight's current location was our clue for where to pour the next bottle. The fact that the weight is already hanging on the scale at the start of the puzzle gave the idea some credence, so I followed through. As before, we can't put only one unit nor all 5 units into the red pipe, as it would provide an odd pressure or too much pressure, respectively. The remaining option would be to put 4 units into the red and the remaining one elsewhere. This yielded multiple answers, somewhere around 9 different possibilities, but I remembered that this scenario has an additional rule: the hanging weight must be under a pipe so that we can identify where to pour the next bottle. There was only one solution that allowed this, which was pouring the last unit into the cyan tube and placing the hanging weight under the purple tube. This is where the idea fails, however, as we next have a 3-diamond bottle which is to be placed into the purple tube. 3*11 is 33, an odd pressure, and once again cannot be balanced by our even-sized hanging weight.
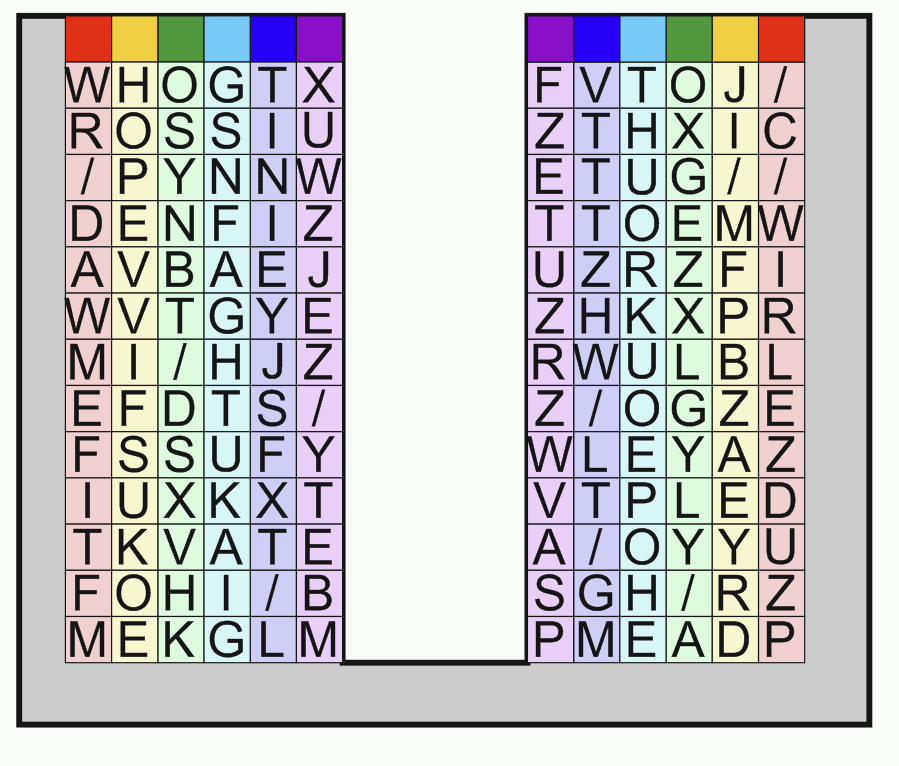
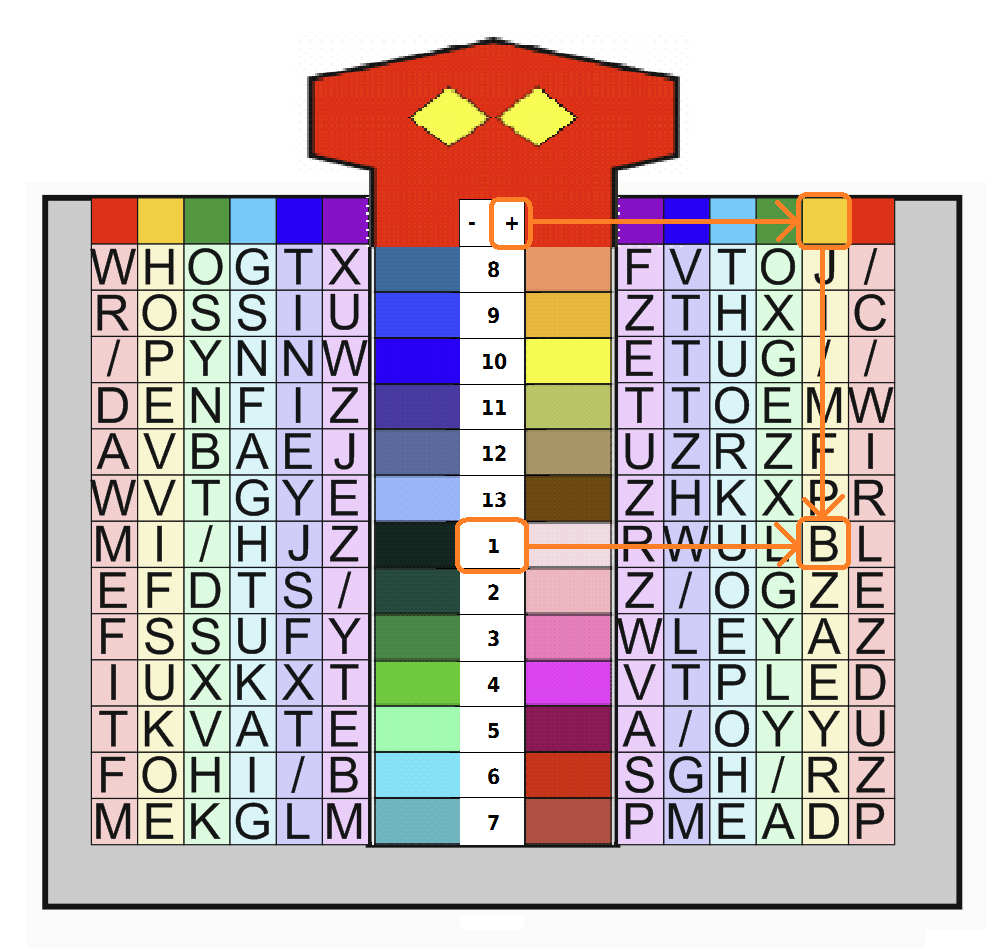
It seems this is the only remaining difficult point to reason out in the puzzle. I figure once we have a system that works without fail, it's just a matter of applying it to reach the answer. Almost certainly, the code will be broken by selecting the key related in color to the pipe that the bottle fills, selecting the line with the color that the hanging weight is beneath, and matching it with the same colored column as the key. For example, if the bottle fills the red pipe and we had to place the hanging weight on hook 4, then we'd insert the red key into the table, select the 4th row from the bottom (where the bright purple is), and select where it meets the red column on the same side, which happens to be the letter D. Noted, for this reasoning to be correct, it assumes that the whole of each bottle is poured into only one tube, which conflicts with most of my other reasoning above. I fear that I have overlooked something very basic and that all of my work and thinking has been flawed from the very beginning.
Original Post
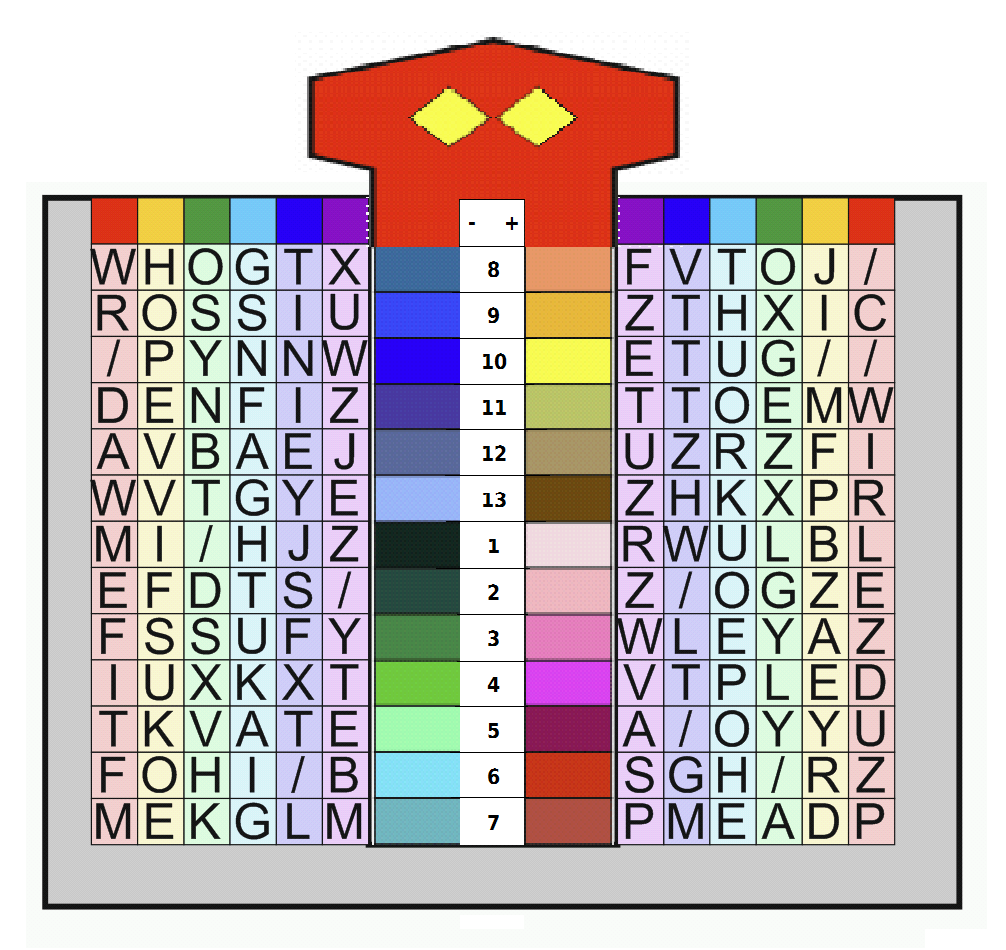
I started into this puzzle today, hopefully I'll get to help you guys out solving this. I see you've made some good progress. I noticed something regarding the scale section, in that there were blank spots where there should be colors (like where the cyan tube ends). The keys offer a solution to this, since all the keys have the same color pairs, and those color pairs match up perfectly to their spacing on the scale.
I've taken the liberty of submitting a complete scale image:

More to do:
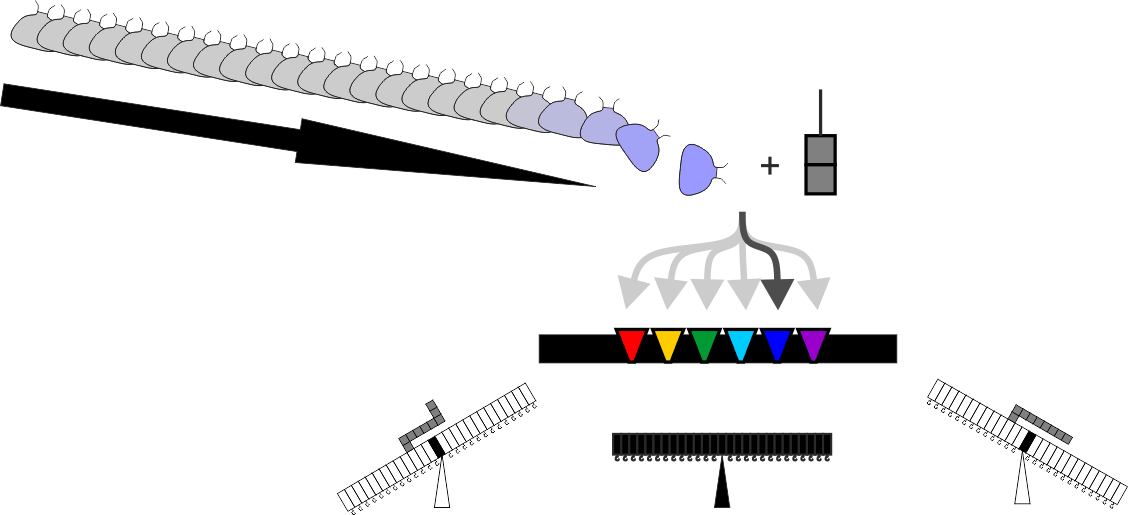
Let's take a look at the tubes. Those things are awkwardly shaped and must have a reason for it. I hypothesize that the tunnels are not actually in the right place. There's that weight on the right hand side; could it actually be a pipe with grey contents? If this is the case, then my hypothesize holds water (excuse the pun) - that is, we now have a correlation between the dots and the scale - 7 dots against 7 pipes. We would probably need to reorganize the pipes on the scale and see where the weight distributes itself when the pipes are full of a color.
My second hypothesis comes from looking more closely at the first instruction. It clearly shows the diamonds on the example bottle, so it's possible that it is giving us a clue about what the diamonds mean. From what it looks like, the diamonds are the amount of a color there is in the bottle, and it is pouring into a tube that already has contents. In this case, I suspect that the contents of the tubes persist, and our weight calculations will compound as we go through the bottles. We will be able to identify our mistakes by any tubes that are overfilled. Furthermore, there are times when we see up to three sets of diamonds on a bottle - this would indicate three different colors, if this hypothesis holds true, and we must compensate for that in our analysis.
*Note: I counted up the number of spaces in the tubes (95 empty) and the number of diamonds on all the bottles combined (93). Assuming I counted accurately, the fact that we can "fill the tubes with diamonds", so to speak, helps support the hypothesis that the diamonds represent the amount of something in each bottle.