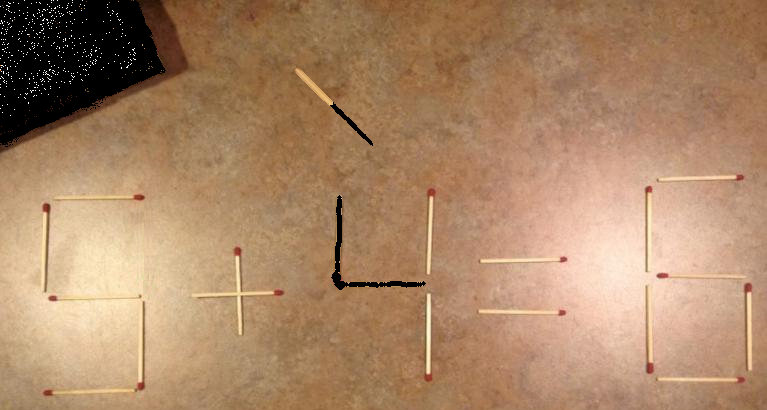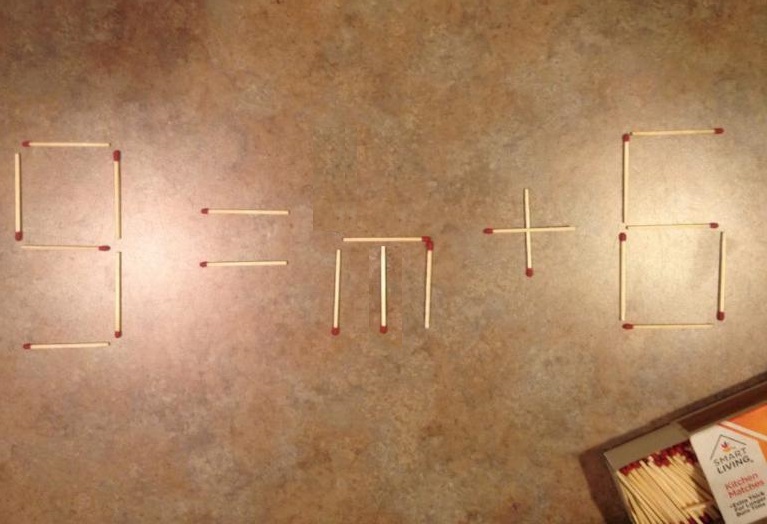13
$\begingroup$

Seems like there's been lots of matchstick puzzles lately, so here's my take on it

Move one matchstick to make the equality right.

EDIT: There are a lot of creative answers, some good, and some definitely questionable at best, but none are "What I was thinking" so I'll drop a hint

Hint: The picture is pretty intentional (besides the glare -- sorry about that)

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Are we allowed to change the spacing? Please see my answer for what I mean by that. $\endgroup$
    – jacoblaw
    Commented Aug 23, 2017 at 1:39

10 Answers 10

Reset to default
10
$\begingroup$

Second-round solution   (accepted)

Following the hint, “the picture is pretty intentional,”...


    ...we can not only move a match but also light it by striking on the pictured box!

Light the vertical match that changes ‘9’ to ‘5’. Move it to burn two matches of ‘4’ to leave it as ‘1’, forming ‘5 + 1 = 6’.


(Nope, couldn't resist torching the entire box of matches while at it.)
Apep spotted a well-devised clue, that match-head positions could lead to undesirable chain combustion everywhere except at the T intersection of ‘4’. This helped eliminate additional burn solutions, such as ‘1 + 4 = 5’ and ‘5 − 4 = 1’, which were included here briefly.


Initial round of solutions   (not accepted)

These three solutions are valid in statistics, engineering and experimental sciences, where...


        ...   ±   (plus-minus) indicates a possible range of numbers.




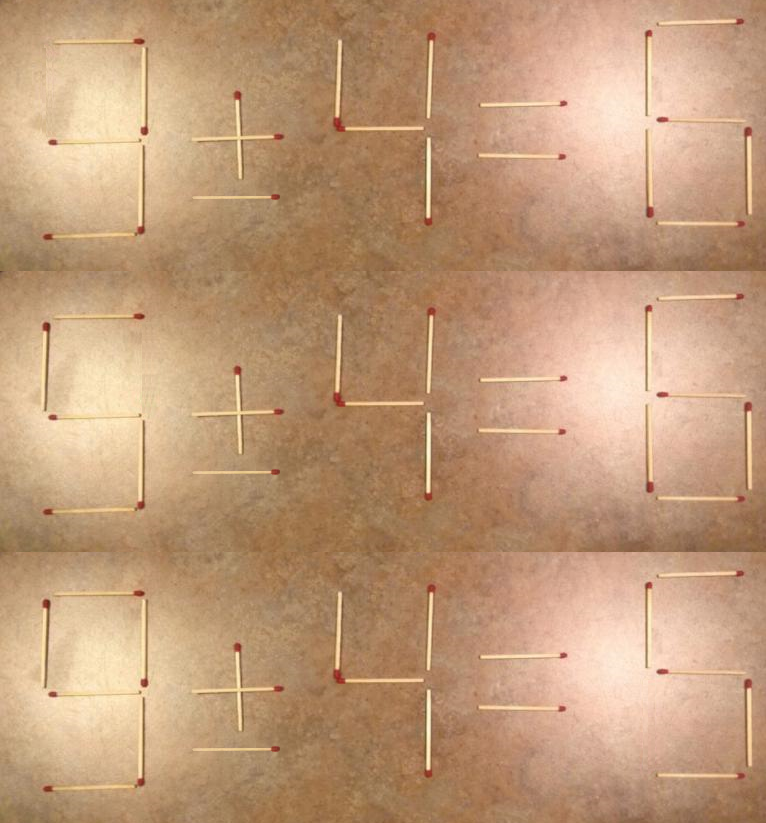
The top two solutions here are  3 ± 4 = 6  and  5 ± 4 = 6,  meaning that 6 is within those  ±  ranges.

That last solution,  9 ± 4 = 5,  is even correct mathematically when  ±  is taken as either-plus-or-minus, as it is sometimes actually used (Taylor series example).

Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ The only issue I see with burning matches is that adjacent matches would be very likely to burn as well, especially if the connection is the head of the match. The arrangement of match heads in the 4 makes your 5+1=6 solution the most likely. There seems to be another "simple" solution using one match to burn entire characters, then placing that match. $\endgroup$
    – Apep
    Commented Aug 26, 2017 at 13:54
  • 3
    $\begingroup$ Yeah, as you guessed based on my placement of which matches are touching and which aren't, the 5+1=6 solution was my intention, but I recognize why this wasn't a very good puzzle now $\endgroup$
    – stacksfiller
    Commented Aug 26, 2017 at 16:27
  • $\begingroup$ The idea is great, @stacksfiller! Takes into account the difference between matches and toothpicks or cotton swabs. You also managed to devise a configuration that brought out a lot of creativity without being solved straightforwardly. $\endgroup$
    – humn
    Commented Aug 26, 2017 at 16:35
  • $\begingroup$ Thank you for noting the positions of the match heads, @Apep! The revised solution is completely safe from unintended combustion. $\endgroup$
    – humn
    Commented Aug 26, 2017 at 22:28
  • $\begingroup$ I refined the solution, @stacksfiller, taking into account match orientations, which are excellent clues I shouldn't have missed in the first place. This solid puzzle is a refreshing variation on matchsticks. $\endgroup$
    – humn
    Commented Aug 26, 2017 at 22:33
7
$\begingroup$

Some "possible" answers

Move the top match of the equal sign down, turning it into a greater than sign. 9 + 4 > 6

Move the vertical match from the +, and put it vertically over the =. 9 - 4 ≠ 6

Take the vertical match from the +, and put it on the the lower left match of the 6. Note that the former is facing up, and the latter is facing down. On contact, the match and anti-match annihilate, emitting a photon. 9 - 4 = 5

And what I believe is the real answer:

Take the upper left match from the 4, and put it into the upper right gap in the six. 9 + -1 = 8

Improve this answer
$\endgroup$
2
  • 2
    $\begingroup$ The question says make the equality right, not to make a true statement. $\endgroup$
    – micsthepick
    Commented Aug 23, 2017 at 1:53
  • 1
    $\begingroup$ @micsthepick Well if we're picking nits, It's impossible to make the equality right with any number of match movements, because if it's right, it's a different equality. $\endgroup$
    – benzene
    Commented Aug 23, 2017 at 2:00
7
$\begingroup$

Another solution

Remove the top-right matchstick from the 9 and place it before and slightly below the 4.

This gives:

$5+1^4=6$

Improve this answer
$\endgroup$
4
$\begingroup$ 

 _            _
|_|    |_|   |_
 _| + -  | =  _|


 9 + -4 = 5                     .

Improve this answer
$\endgroup$
3
  • $\begingroup$ I'm concerned about the lack of space for the - sign $\endgroup$
    – boboquack
    Commented Aug 23, 2017 at 1:36
  • $\begingroup$ @boboquack Oops, I didn't know I couldn't do that $\endgroup$
    – jacoblaw
    Commented Aug 23, 2017 at 1:38
  • $\begingroup$ I don't know whether you can, you'd have to ask stacksfiller. $\endgroup$
    – boboquack
    Commented Aug 23, 2017 at 1:38
4
$\begingroup$

We can move one matchstick from

6 to make it 5 and break it in 2 pieces and put it before 9 and 5

to make the following:

-9 + 4 = -5

Improve this answer
$\endgroup$
2
$\begingroup$

Make the 6 into a capital D. $9_{16}+4_{16}=D_{16}$.

Improve this answer
$\endgroup$
2
  • 3
    $\begingroup$ How does a capital D created with matchsticks look like? Wouldn't it be awfully similar to a zero? $\endgroup$
    – M Oehm
    Commented Aug 23, 2017 at 5:40
  • 4
    $\begingroup$ i prefer to look at it like a 0 looks awfully similar to a D $\endgroup$
    – JMP
    Commented Aug 23, 2017 at 5:55
1
$\begingroup$

Here is my take

Invert your screen.
Pick up the matchstick from inverted 4, and put it in between the other three to make it look like a little 3. Now, the equation says $9 + $ 3 $ = 6$. enter image description here

Improve this answer
$\endgroup$
1
$\begingroup$

Moving a single Match stick

Move the top stick of Digit 9 to the Digit 6 to make it 8. 

               _
|_|    |_|    |_|
 _| +    | =  |_|


 4 + 4 = 8 (bottom stick of first digit have no Mathematical significance)
   OR
 y + 4 = 8 (which solves to "y = 4" (an algebraic eqn.))

Improve this answer
$\endgroup$
1
$\begingroup$

View the equation upside down
($9 = h + 6$)

then,

move the lower leg of the h to the top right of the 6, giving

$9 = 1/1 + 8$

Improve this answer
$\endgroup$
0
$\begingroup$

move two sticks: the vertical from the plus to make the bottom of the three, and the left stick from the original 4 to make the top of the three

9 - 3 = 6

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ The puzzle specifically says to move one stick. (Why is this upvoted?!) $\endgroup$
    – Rubio ♦
    Commented Aug 26, 2017 at 19:54

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.